यदि (1+cosθ+isinθi+sinθ+icosθ)4=cosnθ+isinnθ, तब n बराबर है
1
2
3
4
The correct Answer is:D
Step by step video, text & image solution for यदि ((1+costheta +isintheta)/(i+sintheta+icostheta))^4=cosntheta+isin n theta, तब n बराबर है by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - ERRORLESS HINDICHAPTER - सम्मिश्र संख्यायेंEXERCISE - Exercise117videos
सम्मिश्र संख्यायें
- BOOK - ERRORLESS HINDICHAPTER - सम्मिश्र संख्यायेंEXERCISE - Jee Advanced (More than one correct Answers)23videos
सम्मिश्र संख्यायें
- BOOK - ERRORLESS HINDICHAPTER - सम्मिश्र संख्यायेंEXERCISE - Solved Multiple Choice Questions (सम्मिश्र संख्याओं की ज्यामिति)42videos
सम्मिश्र संख्यायें
- BOOK - ERRORLESS HINDICHAPTER - समुच्चय सिद्धान्त तथा सम्बन्ध EXERCISE - JEE Advanced (Reasoning type questions)3videos
समुच्चय सिद्धान्त तथा सम्बन्ध
- BOOK - ERRORLESS HINDICHAPTER - सरल रेखा EXERCISE - JEE ADVANCE (Matrix Match type questions)4videos
सरल रेखा
Similar Questions
यदि z≠0 एक सम्मिश्र संख्या हो, तो
05:13View Solutionमाना z1 तथा z2 दो सम्मिश्र संख्याएँ इस प्रकार है कि z1≠z2 तथा |z1|=|z2| . यदि z1 धनात्मक वास्तविक भाग तथा z2 ऋणात्मक काल्पनिक भाग है, तब z1+z2z1−z2 होगा
02:10View Solutionसमीकरण z¯z+a¯z+¯az+b=0,b∈R एक वृत्त निरूपित करेगा यदि
06:44View Solutionमाना R वास्तविक संख्याओं का समुच्चय है
कथन-1 : A={(x,y)∈R×R:y−x एक पूर्णांक है} R पर एक तुल्यता संबंध है |
कथन-2 : B={(x,y)∈R×R:x=αy किसी परिमेय संख्या α के लिए} R पर एक तुल्यता संबंध है |05:18View Solution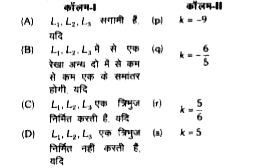
10:06View SolutionIf A=[cosθisinθisinθcosθ], then prove by principal of mathematical induction that An=[cosnθisinnθisinnθcosnθ] for all n∈ N.
07:36View SolutionIf A=[cosθ−sinθsinθcosθ] then show that An=[cosnθ−sinnθsinnθcosnθ]
09:18View SolutionIf z = 3+isinθ4−icosθ is purely real and θ∈(π2,π) ,then arg(sinθ+icosθ) is -
09:11View Solution(cosθ+isinθ)n=cosnθ+isinnθ सभी प्राकृतिक संख्याओं के लिए n
03:27View SolutionIf (1+cosθ+isinθsinθ+i+icosθ)4=cosnθ+isinnθ then n=
03:45View SolutionIf A=[cosθisinθisinθcosθ], then prove by principle of mathematical induction that An=[cosnθisinθisinθcosnθ] for all n∈N.
06:23View Solutionchoose the correct pair of statement :
(i)(cosθ+isinθ)n=cosnθ+isinnθ, x is an integer
(ii)(sinθ+icosθ)n=sinnθ+icosθnθ, n is an integer
(iii) If z=cosθ+isinθ, then z−1=cosθ−isinθ
(iv)z+¯z2=Re(z)05:55View SolutionIf A=[cosθsinθ−sinθcosθ], then prove that An=[cosnθsinnθ−sinnθcosnθ],n∈N.
06:39View Solution(costheta+isintheta)^4/(sintheta+icostheta)^5 =
03:33View Solution(cosθ+isinθ)4(sinθ+icosθ)5 is equal to.
04:31View Solution