यदि z1≠−z2 और |z1+z2|=(1/z1)+(1/z2)∣ तब
कथन-1: z1z2 इकाई मापांक है।
कथन-2: z1 और z2 दोनों इकाई मापांक हैं।
कथन-1 सही है, कथन-2 सही है, कथन- 1 के लिए, कथन-2 का स्पष्टीकरण सही है
कथन-1 सही है, कथन-2 सही है, कथन- 1 के लिए, कथन-2 का स्पष्टीकरण सही नहीं है
कथन-1 सही है, कथन-2 गलत है
कथन-1 गलत है, कथन-2 सही है
The correct Answer is:C
Step by step video, text & image solution for यदि z_1 ne -z_2 और |z_1+z_2|=(1//z_1)+(1//z_2)| तब कथन-1: z_1z_2 इकाई मापांक है। कथन-2: z_1 और z_2 दोनों इकाई मापांक हैं। by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- सम्मिश्र संख्यायेंBOOK - ERRORLESS HINDICHAPTER - सम्मिश्र संख्यायेंEXERCISE - Jee Advanced (Comprehension)
26 Videos
- सम्मिश्र संख्यायेंBOOK - ERRORLESS HINDICHAPTER - सम्मिश्र संख्यायेंEXERCISE - Jee Advanced (Integer)
9 Videos
- सम्मिश्र संख्यायेंBOOK - ERRORLESS HINDICHAPTER - सम्मिश्र संख्यायेंEXERCISE - Jee Advanced (More than one correct Answers)
23 Videos
- समुच्चय सिद्धान्त तथा सम्बन्ध BOOK - ERRORLESS HINDICHAPTER - समुच्चय सिद्धान्त तथा सम्बन्ध EXERCISE - JEE Advanced (Reasoning type questions)
3 Videos
- सरल रेखा BOOK - ERRORLESS HINDICHAPTER - सरल रेखा EXERCISE - JEE ADVANCE (Matrix Match type questions)
4 Videos
Similar Questions
माना A, B, C तीन सम्मिश्र संख्याओं के समुच्चय निम्न प्रकार से परिभाषित हैं
A={z:Imz≥1}
B={z|z−2−i∣=3}
C={z:Re((1−i)z)=√2} .
तब समुच्चय A∩B∩C में अवयवों की संख्या है
View Solutionमान लीजिए कि z कोई सम्मिश्र संख्या है जिसके लिये |z−3−2i|≤2 सत्य है, तो |2z-6+5i| का न्यूनतम मान है
View Solutionमाना z1 तथा z2 दो सम्मिश्र संख्याएँ इस प्रकार है कि z1≠z2 तथा |z1|=|z2| . यदि z1 धनात्मक वास्तविक भाग तथा z2 ऋणात्मक काल्पनिक भाग है, तब z1+z2z1−z2 होगा
View Solutionमाना R वास्तविक संख्याओं का समुच्चय है
कथन-1 : A={(x,y)∈R×R:y−x एक पूर्णांक है} R पर एक तुल्यता संबंध है |
कथन-2 : B={(x,y)∈R×R:x=αy किसी परिमेय संख्या α के लिए} R पर एक तुल्यता संबंध है |
View Solution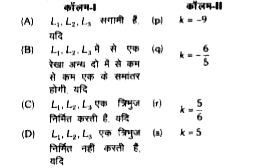
View SolutionIf z1andz2 are two complex numbers for which |(z1−z2)(1−z1z2)|=1and|z2|≠1then (A) |z2|=2 (B) |z1|=1 (C) z1=eiθ (D) z2=eiθ
View SolutionIf z1&z2 are two complex number & if arg z1+z2z1−z2=π2but|z1+z2|≠|z1−z2| then the figure formed by the points represented by 0,z1,z2&z1+z2 is
View SolutionIf |z1|=|z2|=1,then|z1+z2| =
View SolutionIf z1,z2 are complex numbers such that, ∣∣∣z1−3z23−z1.¯z2∣∣∣=1 and |z2|≠1 then find |z1|
View SolutionIf Z1,Z2 be two non zero complex numbers satisfying the equation ∣∣∣Z1+Z2Z1−Z2∣∣∣=1 then Z1Z2+¯¯¯¯¯¯¯¯¯¯¯¯(Z1Z2) is__________
View SolutionIf |z1−1|=Re(z1),|z2−1|=Re(z2)andarg(z1−z2)=π3,then Im(z1+z2)=
View SolutionIf z_1,z_2 The two complex numbers are such that |(z_1+z_2)/(z_1-z_2)| = 1 then z_1/z_2 Is the number that will be
View SolutionIf |z1|=|z2|=1, then prove that |z1+z2|= ∣∣∣1z1+1z2∣∣∣
View SolutionIf ∣∣∣z1z2∣∣∣=1 and arg(z1z2)=0 , then a. z1=z2 b. |z2|2=z1⋅z2 c.z1⋅z2=1 d. none of these
View SolutionIf z1,z2 are nonreal complex and ∣∣∣z1+z2z1−z2∣∣∣=1 then z1z2 is
View Solution
