The velocity →v of a particle of mass 'm' acted upon by a constant force is given by →v(t)=A[cos(kt)¯i−sin(kt)¯j] . Then the angle between the force and the velocity of the particle is (Here A and k are constants)
90∘
0∘
180∘
45∘
The correct Answer is:A
Topper's Solved these Questions
- LAWS OF MOTIONBOOK - AAKASH SERIESCHAPTER - LAWS OF MOTIONEXERCISE - PRACTICE EXERCISE
98 Videos
- LAWS OF MOTIONBOOK - AAKASH SERIESCHAPTER - LAWS OF MOTIONEXERCISE - EXERCISE - I
151 Videos
- GEOMETRICAL OPTICSBOOK - AAKASH SERIESCHAPTER - GEOMETRICAL OPTICSEXERCISE - ADDITIONAL PRACTICE EXERCISE (LEVEL-II PRACTICE SHEET (ADVANCED) INTEGER TYPE QUESTIONS)
6 Videos
- LOGIC GATESBOOK - AAKASH SERIESCHAPTER - LOGIC GATESEXERCISE - Exercise (Very Short Answer)
16 Videos
Similar Questions
The velocity →v of a particle of mass 'm' acted upon by a constant force is given by →v(t)=A[cos(kt)¯i−sin(kt)¯j] . Then the angle between the force and the momentum of the particle is (Here A and k are constants)
View SolutionA particle moving with velocity →V is acted by the three forces shown by the vector triangle PQR. The velocity of the particle will :
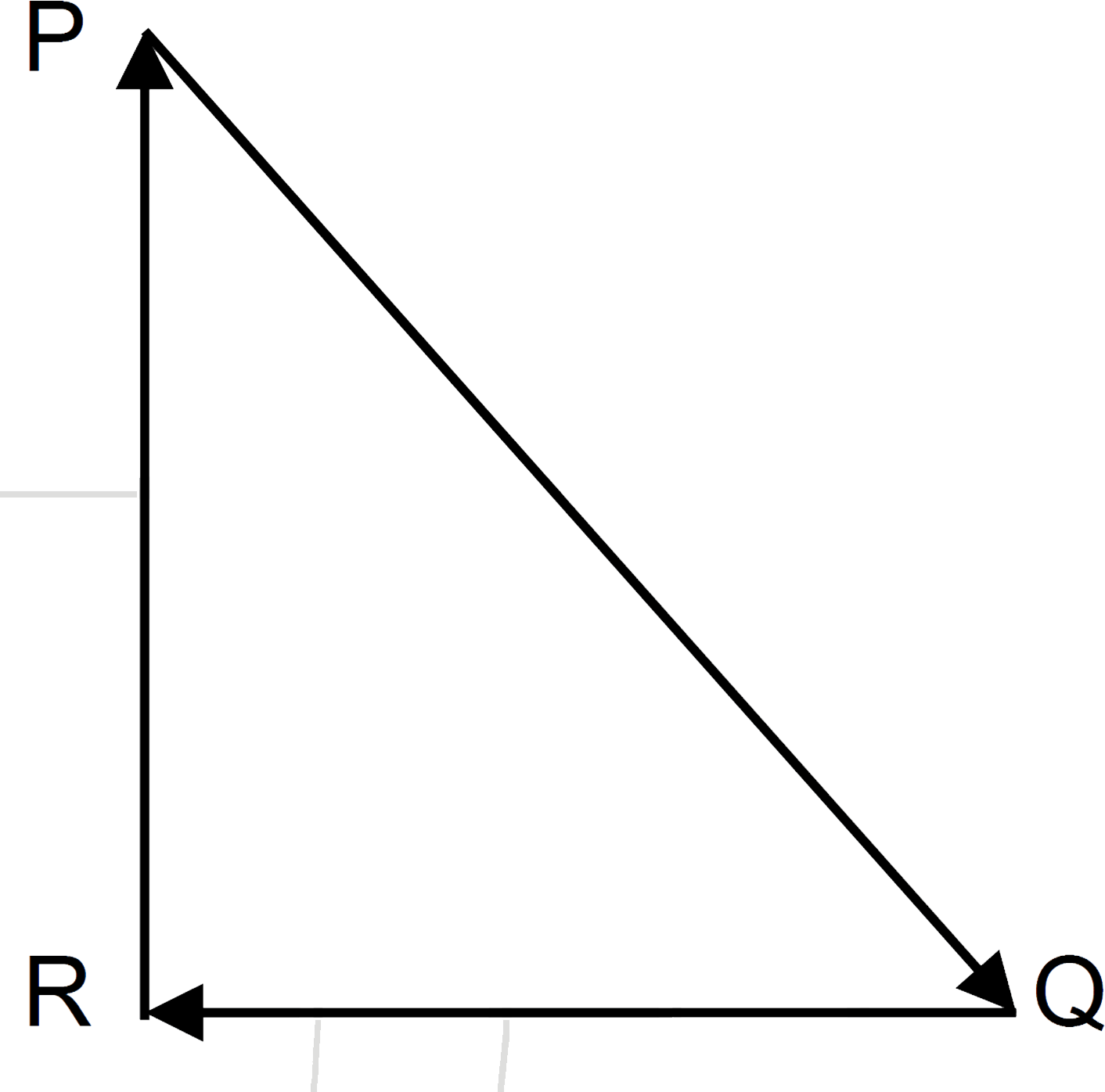
View Solution
Knowledge Check
A particle moving with velocity →V is acted by the three forces shown by the vector triangle PQR. The velocity of the particle will :
 Achange according to the smallest force →QRBincreaseCdecreaseDremain constant
Achange according to the smallest force →QRBincreaseCdecreaseDremain constantThe restoring force F acting on a particle of mass (m) executing a S. H. M. is given by F= -Kx, where x is the displacement and K is a constant. Then the angular velocity of the particle is given by
A√mKB√KmCmKD√mKA force of constant magnitude F acts on a particle moving in a plane such that it is perpendicular to the velocity →v(|→v|=v) of the body and the force is always direct towards a fixed point. Then the angle turned by the velocity vector of the particle as it covers a distance S is (take mass of the particle as m)
AFS2mv2B2FSmv2CFS2mvDFSmv2
A force of constant magnitude F acts on a particle moving in a plane such that it is perpendicular to the velocity →v(|→v|=v) of the body and the force is always direct towards a fixed point. Then the angle turned by the velocity vector of the particle as it covers a distance S is (take mass of the particle as m)
View SolutionA particle moves in the xy plane under the influence of a force such that its linear momentum is →P(t)=A[ˆicos(kt)−ˆjsin(kt)], where A and k are constants. The angle between the force and momentum is
View SolutionA particle moves in the xy plane under the influence of a force such that its linear momentum is →P(t)=A[ˆicos(kt)−ˆjsin(kt)], where A and k are constants. The angle between the force and momentum is
View SolutionA particle moves in the x-y plane under is influence of a force such that its linear momentum is →P(t)=A[ˆicos(kt)−ˆjsin(kt)], where A and k are constants Angle between the force and the momentum is:
View SolutionA particle moving with velocity →V is acted by the three forces shown by the vector triangle PQR. The velocity of the particle will :

View Solution
