The area of cross-section of railway track is 0.01m2. The temperature variation is 10∘C. Coefficient of linear expansion of steel =10−5/∘C. (Young's modulus of steel =1011Nm−2). Calculate the energy stored per meter in the track.
Let α= Coefficient of linear expansion.
∴ Elastic energy =12×stress ×strain ×volume
or U=12×(Y×strain)× strain × volume
or U=1×Y×(strain)2×volume2
α=lL×t=straint
∴α=Change in lengthoriginal length×temperature change
or strain =αt
∴U=Y×α2t2×(area×length)2
or U=1011×(10−5)2×(10)2×0.01×12=5J
∴ Energy stored =5J
Topper's Solved these Questions
- PROPERTIES OF BULK MATTERBOOK - MTG GUIDECHAPTER - PROPERTIES OF BULK MATTEREXERCISE - NEET Cafe (Topicwise Practice Questions)
200 Videos
- PROPERTIES OF BULK MATTERBOOK - MTG GUIDECHAPTER - PROPERTIES OF BULK MATTEREXERCISE - Check Your Neet Vitals
22 Videos
- PHYSICAL WORLD AND MEASUREMENTBOOK - MTG GUIDECHAPTER - PHYSICAL WORLD AND MEASUREMENTEXERCISE - AIPMT / NEET MCQ
14 Videos
- THERMODYNAMICSBOOK - MTG GUIDECHAPTER - THERMODYNAMICSEXERCISE - AIPMT/NEET (MCQs)
26 Videos
Similar Questions
The area of cross-section of a railway track is 0.01m2 . The temperature variation is 10∘C. Coefficient of linear expansion of material of track is 10−5/∘C . The energy stored per meter in the track is ______ J/m.
(Young's modulus of material of track is 1011Nm−2 )
View SolutionThe Young's modulus of steel is 1.9×1011Nm−2 . Calculate its value in dyne cm−2.
View Solution
Knowledge Check
A uniform metal rod of 2mm2 area of cross section is heated from 0∘C to 20∘C. The coefficient of linear expansion of the rod is 12×10−6/∘C. Its Young's modulus of elasticity is 1011N/m2, then the energy stored per unit volume of rod is,
A1440J/m3B2880J/m3C1200J/m3D3880J/m3A uniform rod of cross-section 4mm2 is heated from 0∘C to 10∘C. The coefficient of linear expansion of the rod, ∝=12×10−6/∘C and Young's modulus =1011N/m2. The strain produced in the rod is
A8×10−4B12×10−4C8×10−5D12×10−5A steel rail of length 5 m and area of cross section 40cm2 is prevented from expanding along its length while the temperature rises by 10∘C . If coefficient of linear expansion and Young's modulus of steel are 1.2×105K1 and 2×1011Nm2 respectively, the force developed in the rail is approximately:
A2×107NB1×105NC2×109ND3×10−5N
A uniform metal rod of 2mm2 area of cross section is heated from 0∘C to 20∘C. The coefficient of linear expansion of the rod is 12×10−6/∘C. Its Young's modulus of elasticity is 1011N/m2, then the energy stored per unit volume of rod is,
View SolutionA steel of length 5 m and area of cross-section 40 cm2 is prevented from expanding along its length while the temperature rises by 10∘C. If coefficient of linear expansion and Young's modulus of steel are 1.2×10−5K−1and2×1011Nm−2 respectively, the force developed in the rail is approximately:
View SolutionA steel bolt of cross-sectional area Ab=5×10−5m2 is passed through a cylindrical tube made of aluminium. Cross-sectional area of the tube material is At=10−4m2 and its length isl=50cm. The bolt is just taut so that there is no stress in the bolt and temperature of the assembly increases through △θ=10∘C. Given, coefficient of linear thermal expansion of steel, αb=10−5/∘C.
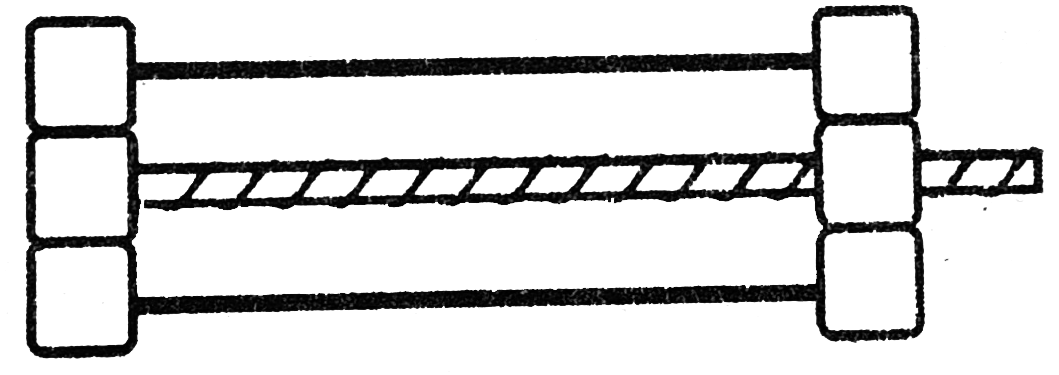
Young's modulus of steel Yb=2×1011N/m2
Young's modulus of Al,Yt=1011N/m2, coefficient of linear thermal expansion of Alalpha_(t)=2xx10^(-5)//^@C
The compressive stress in tube is
View SolutionA steel bolt of cross-sectional area Ab=5×10−5m2 is passed through a cylindrical tube made of aluminium. Cross-sectional area of the tube material is At=10−4m2 and its length isl=50cm. The bolt is just taut so that there is no stress in the bolt and temperature of the assembly increases through △θ=10∘C. Given, coefficient of linear thermal expansion of steel, αb=10−5/∘C.

Young's modulus of steel Yb=2×1011N/m2
Young's modulus of Al,Yt=1011N/m2, coefficient of linear thermal expansion of Alαt=2×10−5/∘C
The tensile stress in bolt is
View SolutionA steel bolt of cross-sectional area Ab=5×10−5m2 is passed through a cylindrical tube made of aluminium. Cross-sectional area of the tube material is At=10−4m2 and its length isl=50cm. The bolt is just taut so that there is no stress in the bolt and temperature of the assembly increases through △θ=10∘C. Given, coefficient of linear thermal expansion of steel, αb=10−5/∘C.

Young's modulus of steel Yb=2×1011N/m2
Young's modulus of Al,Yt=1011N/m2, coefficient of linear thermal expansion of Alαt=2×10−5/∘C
The compressive strain in tube is
View Solution
