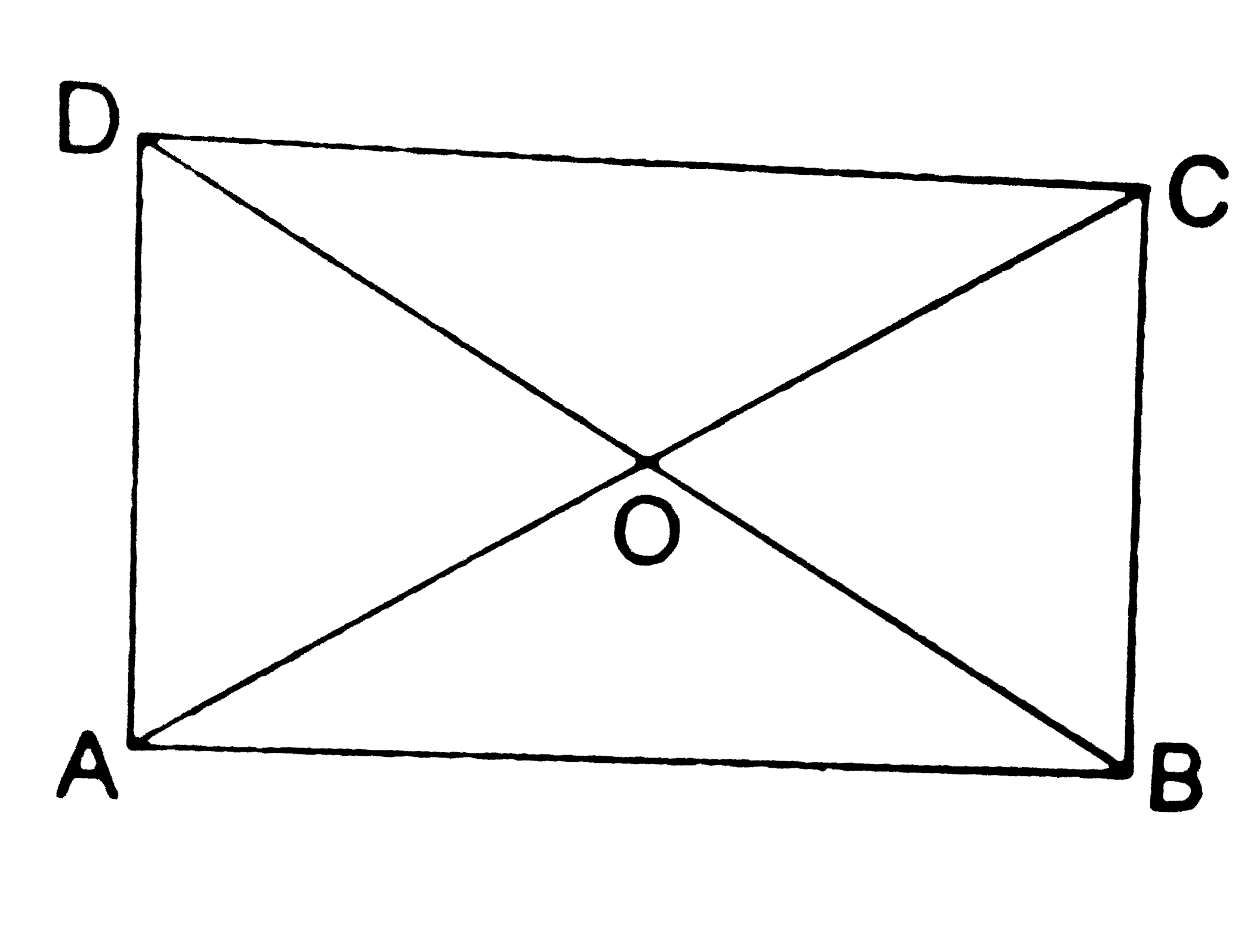
Prove that the centre of the circle circumscribing the cyclic rectangle ABCD is the point of intersection of its diagonals.
Let O be the point of intersection of the diagonals AC and BD fo rect. ABCD. Since the diagonals of a rectangle are equal and bisect each other, we have OA=OB=OC=OD
Hence, O is the centre of the circle through A,B,C,D.
Similar Questions
Knowledge Check
- Question 1 - Select One
A(4,8),B(5,5),C(2,4) and D (1,7) are the vertices of the parallelogram .
Find the coordinates of the point of intersection of its diagonals .A(6,12)B(12,6)C(3,6)D(6,3)
Prove that the line of centres of two interesting circles subtends equal angles at the two points of intersection.
View SolutionProve that the parallelogram circumscribing a circle is a rhombus.
View SolutionProve that the rectangle circumscribing a circle is a square
View SolutionProve that the parallelogram circumscribing a circle is a Rhombus.
View SolutionProve that the circle drawn with any side of a rhombus as a diameter, posses through the point of intersection of its diagonals.
View SolutionOne of the diameters of the circle circumscribing the rectangle ABCD is 4y=x+7. If AandB are the points (−3,4)and(5,4) and slope of the curve y=axb−x at point (1,1) be 2, then centre of circle is : (A) (a,b) (B) (b,a) (C) (−a,−b) (D) (a,−b)
View SolutionOne of the diameters of the circle circumscribing the rectangle ABCD is 4y=x+y. If AandB are the points (−3,4)and(5,4) respectively, find the area of the rectangle and equation of the circle.
View Solution

