ABCD is a rhombus such that ∠ACB=40∘. Find ∠ADB.
A
20∘
B
30∘
C
50∘
D
40∘
Video Solution
Text Solution
The correct Answer is:C
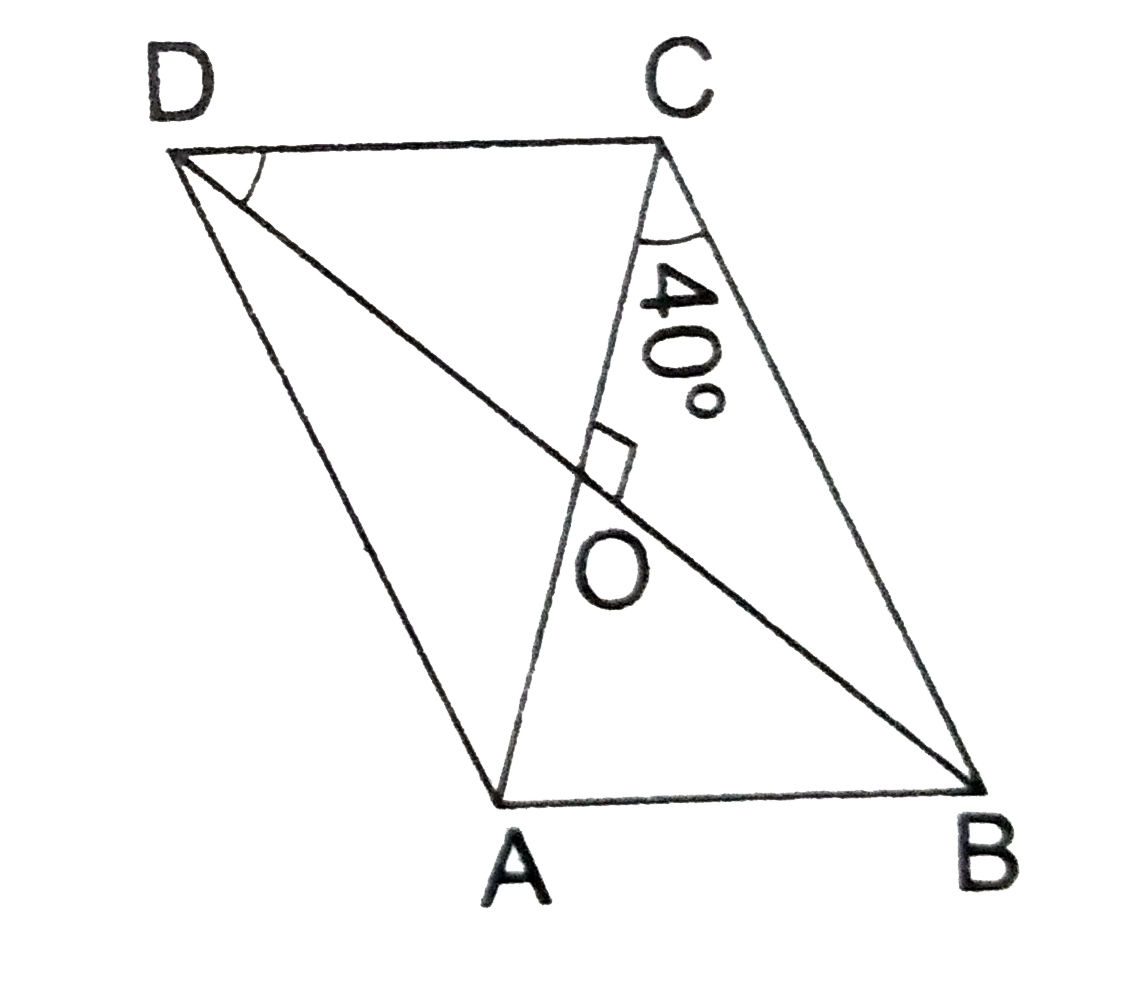
Let the diagonals AC and BD intersect at O.
Then , ∠BOC=90∘. [∴ diagonals of a rhombus intersect at right angles]
In △BOC, we have
∠OCB+∠BOC+∠CBO=180∘ (sum of ∠ of a △)
⇒40∘+90∘+∠CBO=180∘⇒∠CBO=50∘.
Now, ∠ADB=∠CBO=50∘ (alt. interior ∠).
|
Topper's Solved these Questions
- QUADRILATERALSBOOK - RS AGGARWALCHAPTER - QUADRILATERALSEXERCISE - EXAMPLE
32 Videos
- QUADRILATERALSBOOK - RS AGGARWALCHAPTER - QUADRILATERALSEXERCISE - Exercise 10 A
10 Videos
- PROBABILITYBOOK - RS AGGARWALCHAPTER - PROBABILITYEXERCISE - Multiple Choice Questions (Mcq)
16 Videos
- TRIANGLESBOOK - RS AGGARWALCHAPTER - TRIANGLESEXERCISE - MULTIPLE-CHOICE QUESTIONS (MCQ)
10 Videos
Similar Questions
Knowledge Check
ABCD is a rhombus such that ∠ACB=50∘. Then. ∠ADB= ?
A50∘B40∘C65∘D130∘ABCD is a rhombus such that ∠ACB=40∘, then ∠ADB is
A40∘B45∘C50∘D60∘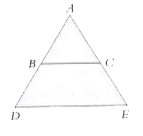
In the figure above BC∣∣DEand∠ABC=∠CED.∠A=∠ACB=30∘. Find ∠AA40∘B50∘C45∘D55∘
In the following figure, O is the centre of the circle. AB is an arc of the circle, such that ∠AOB=80∘.Find ∠ACB.
View SolutionIn a rhombus ABCD if ∠ACB=40∘, then fine ∠ADC.
View SolutionPQRS is rhombus with ∠QPS=50∘. Find ∠PQS.
View Solution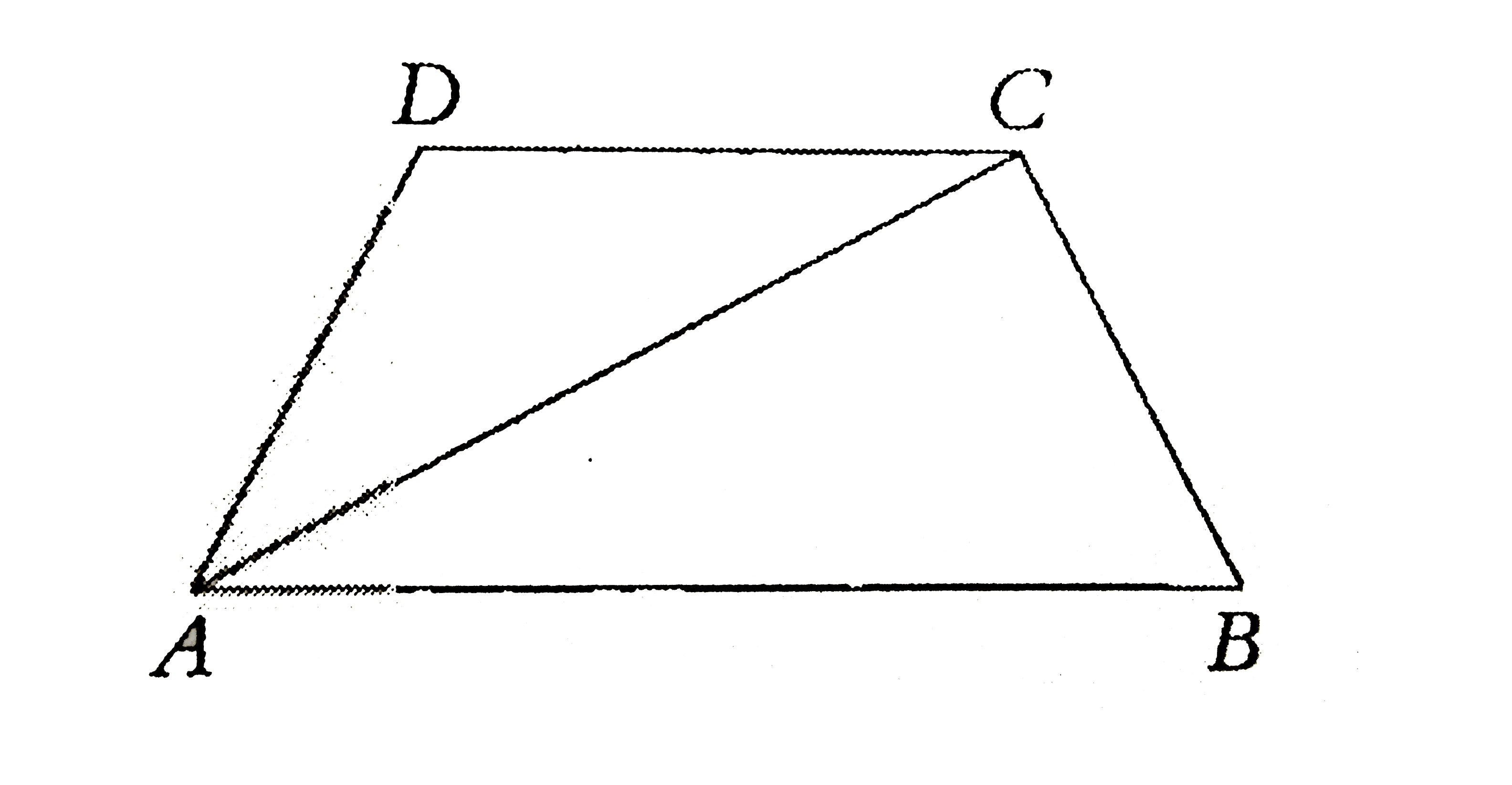
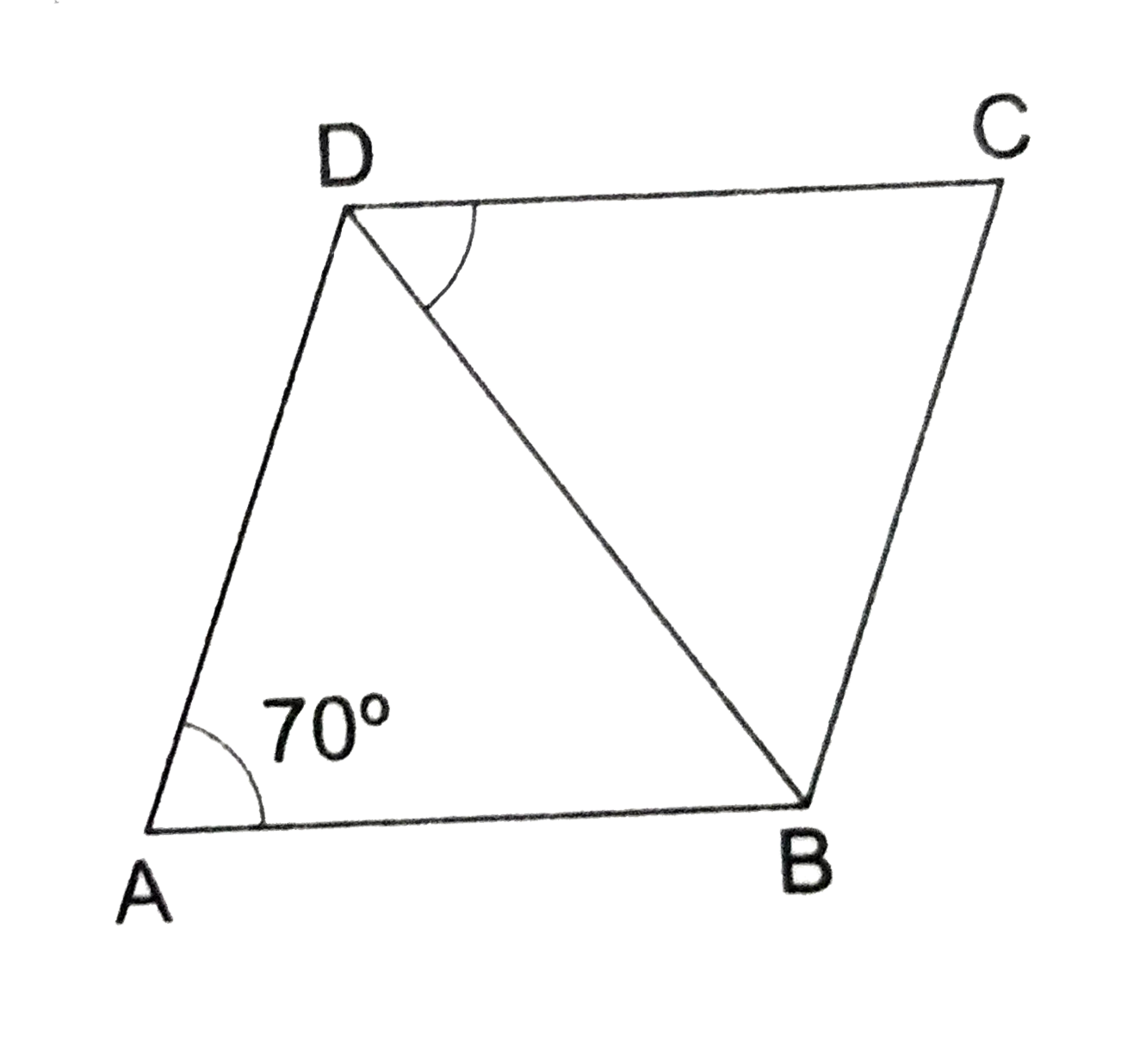
In the figure above ( not to scale ) , ABCD is a trapezium in which AB // DC. ∠ACB=70∘ and ∠ACD=30∘. Find ∠ABC.
View SolutionABCD is rhombus is which ∠B=120∘ and BD=5 cm. Find the perimeter of the rhombus ABCD.
View Solution