Question

Two dice are thrown simultaneously 500 times . Each time , the sum of the two numbers appearing on their tops is noted and recorded as given below :

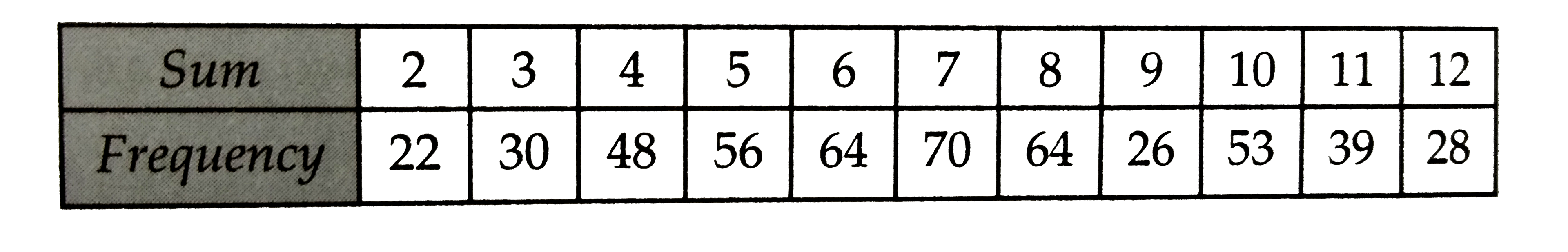
If the two dice are thrown once more , what is the probability of getting a sum
(i) 5 ? (ii) more than 9 ?
(iii) less than or equal to 6 ? (iv) between 6 and 10 ?

Explanation

Total number of times the two dice are thrown = 500 .
(i) Let `E_(1)` be the event of getting the sum 5 . Then ,
P(getting the sum 5)
`= P(E_(1))`
`= ("number of times the sum 5 is obtained")/("total number of times the two dice are thrown")`
= `(56)/(500) = (14)/(125)` .
(ii) Let `E_(2)` be the event of getting a sum more than 9 . Then ,
`E_(2)` = event of getting a sum 10 , 11 or 12 .
`therefore` P (getting a sum more than 9)
`= P (E_(2))`
`= ("number of times a sum 10 , 11 or 12 is obtained")/("total number of times the two dice are thrown")`
`= (53 + 29 + 28)/(500) = (110)/(500) = (11)/(50)`.
Let `E_(3)` be the event of getting a sum less than or equal to 6 .
Then , `E_(3)` = event of getting a sum 2 , 3 , 4 5 or 6 .
`therefore` P (getting a sum less than or equal to 6)
`= P(E_(3))`
`= ("number of times a sum , 2 , 3 , 4 , 5 or 6 is obtained")/("total number of times the two dice are thrown")`
`= (22 + 30 + 48 + 56 + 64)/(500) = (220)/(500) = (11)/(25)`.
(iv) Let `E_(4)` be the event of getting the sum between 6 and 10 .
Then , `E_(4)` = event of getting a sum 7 , 8 or 9 .
`therefore` P(getting the sum between 6 and 10)
`= P(E_(4))`
= `("number of times a sum 7 , 8 or 9 is obtained")/("total number of times the two dice are thrown")`
= `(70 + 64 + 26)/(500) = (160)/(500) = (8)/(25)`.