Taxi charges in a city consist of fixed charges and the remaining depending upon the distance travelled in kilometres. If a man travels 80 kg, he pays ₹ 1330, and travelling 90 km, he pays ₹ 1490. Find the fixed charges and rate per km.
The correct Answer is:fixed charges = ₹ 50, rate per km = ₹ 16
To solve the problem of taxi charges, we need to set up equations based on the information provided. Let's break it down step by step.
Step 1: Define the variables
Let:
- x = Fixed charges (in ₹)
- y = Rate per kilometer (in ₹)
Step 2: Set up the equations based on the given information
From the problem, we have two scenarios:
1. When a man travels 80 km, he pays ₹ 1330:
x+80y=1330(Equation 1)
2. When he travels 90 km, he pays ₹ 1490:
x+90y=1490(Equation 2)
Step 3: Solve the equations
We can solve these two equations simultaneously.
Subtract Equation 1 from Equation 2:
(x+90y)−(x+80y)=1490−1330
This simplifies to:
10y=160
Now, divide both sides by 10:
y=16
Step 4: Substitute y back to find x
Now that we have y, we can substitute it back into either Equation 1 or Equation 2 to find x. We'll use Equation 1:
x+80(16)=1330
Calculating 80×16:
x+1280=1330
Now, subtract 1280 from both sides:
x=1330−1280
x=50
Conclusion
Thus, the fixed charges x are ₹ 50, and the rate per kilometer y is ₹ 16.
Summary of the Solution
- Fixed charges (x) = ₹ 50
- Rate per kilometer (y) = ₹ 16
Let:
- x = Fixed charges (in ₹)
- y = Rate per kilometer (in ₹)
From the problem, we have two scenarios:
x+80y=1330(Equation 1)
x+90y=1490(Equation 2)
We can solve these two equations simultaneously.
(x+90y)−(x+80y)=1490−1330
This simplifies to:
10y=160
Now, divide both sides by 10:
y=16
Now that we have y, we can substitute it back into either Equation 1 or Equation 2 to find x. We'll use Equation 1:
x+80(16)=1330
Calculating 80×16:
x+1280=1330
Now, subtract 1280 from both sides:
x=1330−1280
x=50
Thus, the fixed charges x are ₹ 50, and the rate per kilometer y is ₹ 16.
- Fixed charges (x) = ₹ 50
- Rate per kilometer (y) = ₹ 16
Topper's Solved these Questions
- BOOK - RS AGGARWALCHAPTER - LINEAR EQUATIONS IN TWO VARIABLES EXERCISE - Exercise 3F21videos
LINEAR EQUATIONS IN TWO VARIABLES
- BOOK - RS AGGARWALCHAPTER - LINEAR EQUATIONS IN TWO VARIABLES EXERCISE - Multiple Choice Questions (Mcq)27videos
LINEAR EQUATIONS IN TWO VARIABLES
- BOOK - RS AGGARWALCHAPTER - LINEAR EQUATIONS IN TWO VARIABLES EXERCISE - Exercise 3D31videos
LINEAR EQUATIONS IN TWO VARIABLES
- BOOK - RS AGGARWALCHAPTER - HEIGHTS AND DISTANCESEXERCISE - Multiple Choice Questions (Mcq)25videos
HEIGHTS AND DISTANCES
- BOOK - RS AGGARWALCHAPTER - MEAN,MEDAN,MODE OF GROUPED,DATA CUMULATIVE FREQUENCY GRAPH AND OGIVE EXERCISE - Test Yourself18videos
MEAN,MEDAN,MODE OF GROUPED,DATA CUMULATIVE FREQUENCY GRAPH AND OGIVE
Similar Questions
Taxi charges in a city consist of fixed charges and the remaining depending upon the distance travelled in kilometres. If a person travels 60 km, he pays ₹ 960, and for travelling 80 km, he pays ₹ 1260. Find the fixed charges and the rate per kilometre.
View SolutionThe taxi charges in a city consist of a fixed charge together with the charge for the distance covered. For a distance of 20 km, the charge paid is 205 and for a distance of 25 km, the charge paid is 255. Find the fixed charges and the charge per km. How much does a person have to pay for covering a distance of 50 km? Sol. Let the fixed charges be x and charge per km be y.
View Solution
Knowledge Check
- Question 1 - Select One
The taxi charges in a city contain fixed charges and additional charge/km. The charge for a distance of 10 km is Rs 350 and for 25 km is Rs 800. The charge for a distance of 30 km is:
ARs 900BRs 950CRs 800DRs 750
[" (IV) The taxi charges in a city consist of a fixed charge together with the charge for the "],[" distance covered.For a distance of "10km" ,the charge paid is "₹105" and for a "],[" journey of "15km" ,the charge paid is "₹155" .What are the fixed charges and the "],[" charge per km? How much does a person have to pay for travelling a distance of "],[25km" ? "]
View SolutionThe car hire charges in a city comprise of a fixed charges together with the charge for the distance covered. For a journey of 12 km, the charge paid is Rs 89 and for a journey of 20 km, the charge paid is Rs 145. What will a person have to pay for travelling a distance of 30 km?
View SolutionThe taxi charges in a city contain fixed charges and additional charge per km. The fixed charge is for a distance of upto 5 km and additional charge/km there after. The charge for a distance of 10 km is ₹350 and for 25 km is ₹800. The charge for a distance of 30 km is :-
एक शहर में टैक्सी का एक निश्चित किराया है और प्रति किमी अतिरिक्त शुल्क है। निश्चित किराया 5 किमी की दूरी के लिए है तथा उसके बाद प्रति किमी के लिए अतिरिक्त किराया है। 10 किमी दूरी का किराया ₹350 है तथा 25 किमी दूरी का किराया ₹800 है तो 30 किमी की दूरी का किराया ज्ञात करें।View SolutionThe taxi charges in a city comprise of a fixed charge together with the charge for the distance covered. For a journey of 10 km the charge paid is Rs 75 and for a journey of 15 km the charge paid is Rs 110. What will a person have to pay for travelling a distance of 25 km?
View SolutionThe taxi charges in a city comprise of a fixed charge, together with the charge of the distance covered. For a journey of 16 km, the charges paid are Rs 156 and for a journey of 24 km, the charges paid are Rs 204. What will a person have to pay for travelling a distance of 30 km? (a) Rs 236 (b) Rs 240 (c) Rs 248 (d) Rs 252
View SolutionThe taxi charges in a city comprise of a fixed charge of Rs 100 for 5 kms and Rs 16 per km for every additional km. If the amount paid at the end of the trip was Rs 740, then find the distance travelled.
View SolutionIt is common that Governments revise travel fares from time to time based on various factors such as inflation ( a general increase in prices and fall in the purchasing value of money) on different types of vehicles like auto, Rickshaws, taxis, Radio cab etc. The auto charges in a city comprise of a fixed charge together with the charge for the distance covered. Study the following situations
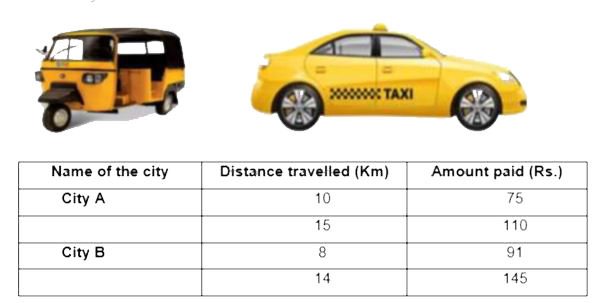
Situation 1: In city A, for a journey of 10 km, the charge paid is Rs 75 and for a journey of 15 km, the charge paid is Rs 110.
Situation 2: In a city B, for a journey of 8km, the charge paid is Rs91 and for a journey of 14km, the charge paid is Rs 145.
Refer to situation 1:
A person travels a distance of 50km. The amount he has to pay isView Solution

