A mild steel wire of length 1.0 m and cross sectional area 0.50×10−2cm2 is stretched, well within its elastic limit, horizontally between two pillars. A mass of 100 g is suspended from the mid-point of the wire. Calculate the depression at the midpoint.Solution in Gujrati
Let AB a mild steel wire of length = 1 m and its cross sectional area A=0.50×10−2cm2
Mass suspended m=100g=0.1kgandC is mid-point of wire AB
∴AC=CB=L=12andCD=x
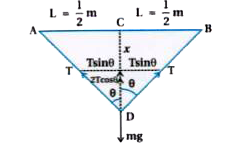
∴AD=√AC2+CD2andDB=√L2+x2=√L2+x2
Increas in length =AD+DB−2L
ΔL=√L2+x2+√L2+x2−2L
=2√L2+x2−2L
=2L[(1+x2L2)−1]
=2L[(1+x22L2+...)−1]
Only two terms taken from bionomial theorem.s expansion
=2L[1+x22L2−1]
=2L×x22L2
ΔL=x2L ...(1)
Longitudinal strain =ΔL2L=x22L2 ...(2)
If T be the tension in the wire, then in equilibrium
2Tcosθ=mg
∴T=mg2cosθ
=mg2xx√x2+L2
[∵From figure, cosθCDAD=x√x2+L2]=mg√x2+L22x
Ifx<<L⇒x neglected T=mgL2x ...(3)
∴Stressσ=TA=mgL2xA ...(4)
[∵ Equation (2)]
∴ Young modulus Y=StressStrain
=mgL2xA×2L2x2
Y=mgL3Ax3
∴x3=mgL3AY
∴x=L[mgAY]13
∴x=12[0.1×9.80.5×10−6×2×1011]13
∴x=12[0.98105]13
∴x12[9.8×10−6]13
logx=12[13log9.8×10−6]
=12{13[0.9912+¯¯¯¯¯¯¯¯¯¯¯6.0000]}
=12{13[¯6.9912]}=12[¯2.3304]
By antilog,
x=2.14×10−2m
thereforx=1.07×10−3m
∴x≈1cm=0.01m
Topper's Solved these Questions
- MACHANICAL PROPERTIES OF SOLIDS Book:KUMAR PRAKASHANChapter:MACHANICAL PROPERTIES OF SOLIDS Exercise:SECTION-C
Explore 66 Videos
- MACHANICAL PROPERTIES OF SOLIDS Book:KUMAR PRAKASHANChapter:MACHANICAL PROPERTIES OF SOLIDS Exercise:SECTION-D
Explore 29 Videos
- MACHANICAL PROPERTIES OF SOLIDS Book:KUMAR PRAKASHANChapter:MACHANICAL PROPERTIES OF SOLIDS Exercise:SECTION-A TRY YOURSELF (VSQs)
Explore 40 Videos
- LAW OF MOTIONBook:KUMAR PRAKASHANChapter:LAW OF MOTIONExercise:(QUESTION PAPER) SECTION-D
Explore 1 Video
- MECHANICAL PROPERTIES OF FLUIDS Book:KUMAR PRAKASHANChapter:MECHANICAL PROPERTIES OF FLUIDS Exercise:QUESTION PAPER (SECTION -C)
Explore 3 Videos
Similar Questions
What is elasticity ? What is plastic body and plasticity?
View SolutionModulus of rigidity of an ideal liquid is ......
View SolutionWhat are solid bodies composed ?
View SolutionA 70 kg man stands in contact against the inner wall of a hollow cylindrical drum of radius 3 m rotating about its vertical axis with 200 rev/min. The coefficient of friction between the wall and his clothing is 0.15. What is the minimum rotational speed of the cylinder to enable the man to remain stuck to the wall (without falling) when the floor is suddenly removed ?
View SolutionGlycerine flows steadily through a horizontal tube of length 1.5m and radius 1.0 cm . If the amount of glycerine collected per second at one is 4.0×10−3kgs−1 , what is the pressure difference between the two ends of the tube ? (Density of glycerine =1.3×10103kgm−3 and viscosity of glycerine =0.83 Pa s). [ you may also like to check if the assumption of laminar flow in the tube is correct]
View SolutionA mild steel wire of length 1.0 m and cross-sectional are 0.5 xx 10^(-20)cm^(2) is streached, well within its elastic limit, horizontally between two pillars. A mass of 100 g is suspended from the mid point of the wire, calculate the depression at the mid point. g = 10ms^(-2), Y=2 xx 10^(11)Nm^(-2 .
View SolutionA mild steel wire of length 1.0m and cross sectional area 5.0×10−2cm2 is stretched, within its elastic limit horizontally between two pillars. A mass of 100g is suspended form the midpont of the wire. Calculate the depression at the midpoint (Ysteel=200GPa)
View SolutionA mild steel wire of length 1.0m and cross sectional area 2L is strethched, within its elastic limit horizontally between two pillars(figure). A mass of m is suspended form the midpont of the wire. Strain in the wire is
View Solutionमृदु इस्पात के एक तार, जिसकी लंबाई 1.0 मीटर तथा अनुप्रस्थ परिच्छेद का क्षेत्रफल 0.50×10−2 सेमी 2 है, को दो खम्भों की बीच क्षैतिज दिशा में प्रत्यास्थ सीमा के अंदर ही तनित किया जाता है। तार के मध्य-बिन्दु से 100 ग्राम का एक द्रव्यमान लटका दिया जाता है। मध्य-बिन्दु पर अवनमन की गणना कीजिए।
View SolutionA mild steel wire of Length 2L and cross sectional Area A is stretched well within elastic limit, horizontally between two pillars. A man m is suspended from the mid point of the wire strain in the wire is
View SolutionA mild steel wire of length 1.0 m and cross sectional area 0.50 times 10^-2 (cm)^2 is stretched , well within its elastic limit, horizontally between two pillars. A mass of 100 g is suspended from the mid-point of the wire. Calculate the depression at the mid -point.
View SolutionA mild steel wire of length 1.0 m and cross - sectional area 0.50xx10^(-2)" cm"^(2) is stretched, well within its elastic limit, horizontally between two pillars. A mass of 100 g is suspended from the mid - point of the wire. Calculate the depression at the mid point.
View SolutionA mild steel wire of length 1.0 m and cross - sectional area 0.50xx10^(-2)" cm"^(2) is stretched, well within its elastic limit, horizontally between two pillars. A mass of 100 g is suspended from the mid - point of the wire. Calculate the depression at the mid point.
View SolutionA mild steel wire of length 1.0 m and cross - sectional area 0.50×10−2 cm2 is stretched, well within its elastic limit, horizontally between two pillars. A mass of 100 g is suspended from the mid - point of the wire. Calculate the depression at the mid point.
View SolutionA mild steel wire of length 1.0 m and cross - sectional area 0.50xx10^(-2)" cm"^(2) is stretched, well within its elastic limit, horizontally between two pillars. A mass of 100 g is suspended from the mid - point of the wire. Calculate the depression at the mid point.
View Solution
