Let A and B be two real matrices such that AB = BA and det . If is a cube root of unity then det is equal to _______ .
Text Solution
The correct Answer is:
|
Topper's Solved these Questions
- MATRICESBOOK - MCGROW HILL PUBLICATIONCHAPTER - MATRICESEXERCISE - Questions from Previous Years. AIEEE /JEE Main Papers
71 Videos
- MATRICESBOOK - MCGROW HILL PUBLICATIONCHAPTER - MATRICESEXERCISE - Questions from Previous Years. B -Architecture Entrance Examination Papers
22 Videos
- MATRICESBOOK - MCGROW HILL PUBLICATIONCHAPTER - MATRICESEXERCISE - EXERCISE ( LEVEL -2 ) ( single Correct Answer Type Questions)
17 Videos
- MATHEMATICAL REASONING BOOK - MCGROW HILL PUBLICATIONCHAPTER - MATHEMATICAL REASONING EXERCISE - QUESTIONS FROM PREVIOUS YEARS. B. ARCHITECTURE ENTRANCE EXAMINATION PAPERS
11 Videos
- PARABOLABOOK - MCGROW HILL PUBLICATIONCHAPTER - PARABOLAEXERCISE - QUESTIONS FROM PREVIOUS YEARS. B-ARCHITECTRE ENTRANCE EXAMINATION PAPERS
9 Videos
Similar Questions
Knowledge Check
If ω≠1 is a cube root of unity, then 1,ω,ω2
Aare vertices of an equilateral triangleBlie on a straight lineClie on a circle of radius √3/2DNone of theseIf A and B are two matrices such that AB=B and BA=A, then A2+B2 is equal to
AA2+B2BOCA2+2AB+B2DA+BLet A and B are square matrices of order 3 such that AB2=BAandBA2=AB. If (AB)2=A3Bn, then m is equal to
A3B4C5D7
If a, b, c are distanct integers and w≠1 is a cube root of unity, then
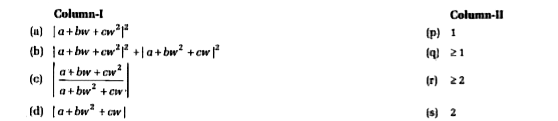
View SolutionLet A and B be two 3×3 real matrices such that
AB≠BA
AB−B2A2=I3
A3+B3=O3 .
then det (BA−A2B2). Then |12a +5i|= _______
View SolutionLet A and B be (3×3) matrices with det A = 4 and det B = 3
What is det (2AB) equal to ?
View SolutionLet A and B be (3×3) matrices with det A = 4 and det B = 3
What is det (3AB−1) equal to ?
View SolutionLet A and B be (3 × 3) matrices with det A = 4 and det B = 3.
What is det (2AB) equal to ?
View Solution
