Show that the reflection of the line px+qy+r=0 in the line x+y+1=0 is the line qx+py+(p+q−r)=0, where p≠−q.
x−y=p+q
x−y=p−q
x+y+1=0
x+y−1=0
The correct Answer is:C
Step by step video, text & image solution for Show that the reflection of the line px+qy+r=0 in the line x+y+1 =0 is the line qx+py+(p+q-r)=0, where p!= -q. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - MCGROW HILL PUBLICATIONCHAPTER - CARTESIAN SYSTEM OF RECTANGULAR COORDINATES AND STRAIGHT LINES EXERCISE - EXERCISE (LEVEL 2) SINGLE CORRECT ANSWER TYPE QUESTIONS21videos
CARTESIAN SYSTEM OF RECTANGULAR COORDINATES AND STRAIGHT LINES
- BOOK - MCGROW HILL PUBLICATIONCHAPTER - CARTESIAN SYSTEM OF RECTANGULAR COORDINATES AND STRAIGHT LINES EXERCISE - EXERCISE (NUMERICAL ANSWER TYPE QUESTIONS)21videos
CARTESIAN SYSTEM OF RECTANGULAR COORDINATES AND STRAIGHT LINES
- BOOK - MCGROW HILL PUBLICATIONCHAPTER - CARTESIAN SYSTEM OF RECTANGULAR COORDINATES AND STRAIGHT LINES EXERCISE - EXERCISE (CONCEPT - BASED) SINGLE CORRECT ANSWER TYPE QUESTIONS15videos
CARTESIAN SYSTEM OF RECTANGULAR COORDINATES AND STRAIGHT LINES
- BOOK - MCGROW HILL PUBLICATIONCHAPTER - AREA BY INTEGRATIONEXERCISE - Question from Previous Years. B-Architecture Entrance Examination Papers12videos
AREA BY INTEGRATION
- BOOK - MCGROW HILL PUBLICATIONCHAPTER - CIRCLES AND SYSTEMS OF CIRCLES EXERCISE - QUESTIONS FROM PREVIOUS YEARS. B-ARCHITECTURE ENTRANCE EXAMINATION PAPERS17videos
CIRCLES AND SYSTEMS OF CIRCLES
Similar Questions
Knowledge Check
- Question 1 - Select One
If r is the geometric mean of p and q, then the line px+qy+r=0
Ahas a fixed directionBpasses thorugh a fixed pointCforms with the axs a triangle of constant areaDsum of its intercepts on the axes is constant - Question 2 - Select One
The locus of the orthocentre of the triangle formed by the lines (1+p)x−py+p(1+p)=0
(1+q)x−qy+q(1+q)=0 and y=0 where p≠q isAa hyperbolaBa parabolaCan ellipseDa straight line - Question 3 - Select One
Shown below are the graphs of the lines y−2x=0,x+y=6andpx+qy=r
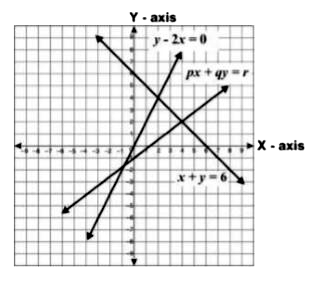
Which of these is the solution for the pair of equations x+y=6 and px+qy=rAx=2,y=4Bx=4, y=2Cx=3, y=2DWe cannot say for sure as the values of p and q are not known.
Let P be the foot of perpendicular drawn from a point Q on the line x + y +4 = 0 to the line 5x + y = 0. If P is the image of point R(12, 0) about the line x + y = 2, then coordinates of Q are
View SolutionThe locus of the orthocentre of the triangle formed by the lines (1+p)x−py+p(1+p)=0, (1+q)x−qy+q(1+q)=0 and y = 0, where p≠q, is (A) a hyperbola (B) a parabola (C) an ellipse (D) a straight line
View SolutionThe locus of the orthocentre of the triangle formed by the lines (1+p)x−py+p(1+p)=0,(1+q)x−qy+q(1+q)=0 and y = 0, where p≠⋅q, is (A) a hyperbola (B) a parabola (C) an ellipse (D) a straight line
View SolutionLet image of the line x−13=y−35=z−42 in the plane 2x−y+z+3=0 be L. A plane 7x+py+qz+r=0 is such that it contains the line L and perpendicular to the plane 2x−y+z+3=0 then p=1 (b) q=42 p+q+r=30 (d) p+q+r=0
View SolutionSolve the following pair of linear equations :
px+qy=p−q
qx−py=p+qView Solution

