If three vectors V1=αi+j+k,V2=i+βj−2k and V3=i+j are coplanar, and V1 and V3 are perpendicular, then the vector V1×V2 is:
−i+j+2k
i−j+2k
−i+j
2i−2j+k
The correct Answer is:B
To solve the problem, we need to follow these steps:
Step 1: Identify the vectors
We are given three vectors:
- V1=αi+j+k
- V2=i+βj−2k
- V3=i+j
Step 2: Use the coplanarity condition
The vectors V1,V2,V3 are coplanar if their scalar triple product is zero:
V1⋅(V2×V3)=0
This can be expressed as the determinant:
∣∣
∣∣α111β−2110∣∣
∣∣=0
Step 3: Calculate the determinant
Calculating the determinant:
=α∣∣∣β−210∣∣∣−1∣∣∣1−210∣∣∣+1∣∣∣1β11∣∣∣
Calculating each of these 2x2 determinants:
1. ∣∣∣β−210∣∣∣=0−(−2)=2
2. ∣∣∣1−210∣∣∣=0−(−2)=2
3. ∣∣∣1β11∣∣∣=1−β
Putting it all together:
α⋅2−1⋅2+(1−β)=0
This simplifies to:
2α−2+1−β=0(1)
Step 4: Use the perpendicularity condition
Vectors V1 and V3 are perpendicular, so:
V1⋅V3=0
Calculating the dot product:
(αi+j+k)⋅(i+j)=α⋅1+1⋅1+0=α+1=0
Thus, we have:
α+1=0⇒α=−1(2)
Step 5: Substitute α into equation (1)
Substituting α=−1 into equation (1):
2(−1)−2+1−β=0
This simplifies to:
−2−2+1−β=0⇒−3−β=0⇒β=−3
Step 6: Write the vectors with found values
Now substituting α and β back into the vectors:
- V1=−i+j+k
- V2=i−3j−2k
- V3=i+j
Step 7: Calculate V1×V2
Now we compute the cross product V1×V2:
∣∣
∣∣ijk−1111−3−2∣∣
∣∣
Calculating the determinant:
=i∣∣∣11−3−2∣∣∣−j∣∣∣−111−2∣∣∣+k∣∣∣−111−3∣∣∣
Calculating each of these 2x2 determinants:
1. ∣∣∣11−3−2∣∣∣=(1)(−2)−(1)(−3)=−2+3=1
2. ∣∣∣−111−2∣∣∣=(−1)(−2)−(1)(1)=2−1=1
3. ∣∣∣−111−3∣∣∣=(−1)(−3)−(1)(1)=3−1=2
Putting it all together:
V1×V2=i(1)−j(1)+k(2)=i−j+2k
Final Answer
Thus, the vector V1×V2 is:
i−j+2k
We are given three vectors:
- V1=αi+j+k
- V2=i+βj−2k
- V3=i+j
The vectors V1,V2,V3 are coplanar if their scalar triple product is zero:
V1⋅(V2×V3)=0
This can be expressed as the determinant:
∣∣
∣∣α111β−2110∣∣
∣∣=0
Calculating the determinant:
=α∣∣∣β−210∣∣∣−1∣∣∣1−210∣∣∣+1∣∣∣1β11∣∣∣
Calculating each of these 2x2 determinants:
1. ∣∣∣β−210∣∣∣=0−(−2)=2
2. ∣∣∣1−210∣∣∣=0−(−2)=2
3. ∣∣∣1β11∣∣∣=1−β
α⋅2−1⋅2+(1−β)=0
This simplifies to:
2α−2+1−β=0(1)
Vectors V1 and V3 are perpendicular, so:
V1⋅V3=0
Calculating the dot product:
(αi+j+k)⋅(i+j)=α⋅1+1⋅1+0=α+1=0
Thus, we have:
α+1=0⇒α=−1(2)
Substituting α=−1 into equation (1):
2(−1)−2+1−β=0
This simplifies to:
−2−2+1−β=0⇒−3−β=0⇒β=−3
Now substituting α and β back into the vectors:
- V1=−i+j+k
- V2=i−3j−2k
- V3=i+j
Now we compute the cross product V1×V2:
∣∣
∣∣ijk−1111−3−2∣∣
∣∣
=i∣∣∣11−3−2∣∣∣−j∣∣∣−111−2∣∣∣+k∣∣∣−111−3∣∣∣
Calculating each of these 2x2 determinants:
1. ∣∣∣11−3−2∣∣∣=(1)(−2)−(1)(−3)=−2+3=1
2. ∣∣∣−111−2∣∣∣=(−1)(−2)−(1)(1)=2−1=1
3. ∣∣∣−111−3∣∣∣=(−1)(−3)−(1)(1)=3−1=2
V1×V2=i(1)−j(1)+k(2)=i−j+2k
Thus, the vector V1×V2 is:
i−j+2k
Topper's Solved these Questions
- VECTOR ALGEBRABOOK - MCGROW HILL PUBLICATIONCHAPTER - VECTOR ALGEBRAEXERCISE - QUESTIONS FROM PREVIOUS YEARS. AIEEE/JEE MAIN PAPERS
65 Videos
- TRIGONOMETRICAL IDENTITIES AND EQUATIONS BOOK - MCGROW HILL PUBLICATIONCHAPTER - TRIGONOMETRICAL IDENTITIES AND EQUATIONS EXERCISE - Questions from Previous Years. B-Architecture Entrance Examination Papers
20 Videos
Similar Questions
Knowledge Check
The vector(s) which is/are coplanar with vectors ˆi+ˆj+2ˆkandˆi+2ˆj+ˆk are perpendicular to the vector ˆi+ˆj+ˆk is are
Aj−kB−i+jCi−jD−j+kA unit vector coplanar with i+j+2k and i+2j+k and perpendicular to i+j+k is.......
Ai+j√2B±−j+k√2C±j−k√2DnoneIf →v1+→v2 is perpendicular to →v1−→v2, then
A→v1 is perpendicular to →v2.B|→v1|=|→v2|C→v1 is a null vectorDThe angle between →v1 and →v2 can have any value
A unit vector coplanar with i+j+2k and i+2j+k and perpendicular to i+j+k is
View SolutionThree capacitors C1,C2 and C3 are connected as shown in, The potentials of P,Q, and R are V1,V2, and V3, respectively. Find the potential V0 at the functionO.
 .
.
View SolutionTwo batteries V1 and V2 are connected to three resistors as shown below. If V1=2Vand V2 = 0 V, the current I = 3 mA. If V1 = 0 V and V2 = 4V, the current I = 4mA. Now, if V1 = 10 V and V2 = 10 V, the current I will be-
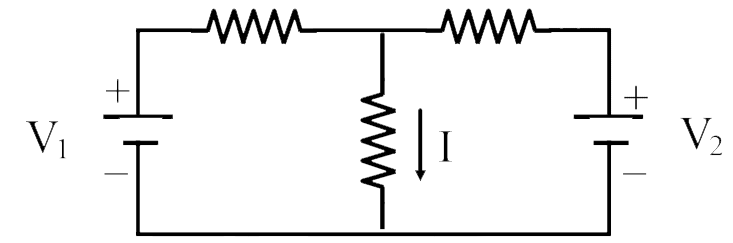
View SolutionA unit vector coplanar with i+j+2k and i+2j+k and perpendicular to i+j+k is.......
View SolutionA vector of magnitude √2 units and coplanar with vector 3i−j−kandi+j−2k and perpendicular to vector 2i+2j+k.....
View Solution
