For the reaction 2N2O5→4NO2+O2, rate and rate constant are 1.02×10−4Msec−1 and 3.4×10−5sec−1 respectively then concentration of N2O5 at that time will be (in moles /lit)Solution in Telugu
3
1.732
1
1.5
The correct Answer is:A
r=K.[N2O5]1,1.02×10−4=3.4×10−5×[N2O5]1,[N2O5]−1=1.02×10−43.4×10−5=1.02×103.4=3
Topper's Solved these Questions
- BOOK - AAKASH SERIESCHAPTER - CHEMICAL KINETICSEXERCISE - LEVEL-II(LECTURE SHEET EXERCISE-II LINKED COMPREHENSION TYPE QUESTIONS)9videos
CHEMICAL KINETICS
- BOOK - AAKASH SERIESCHAPTER - CHEMICAL KINETICSEXERCISE - LEVEL-II(LECTURE SHEET EXERCISE-III MATCH THE FOLLOWING QUESTIONS)3videos
CHEMICAL KINETICS
- BOOK - AAKASH SERIESCHAPTER - CHEMICAL KINETICSEXERCISE - LEVEL-1 (EXERCISE -II ORDER, MOLECULARITY, HALF LIFE:)34videos
CHEMICAL KINETICS
- BOOK - AAKASH SERIESCHAPTER - CHEMICAL KINETCSEXERCISE - EXERCISE - 3.245videos
CHEMICAL KINETCS
- BOOK - AAKASH SERIESCHAPTER - CHEMICAL THERMODYNAMICSEXERCISE - Additional Practice Exercise54videos
CHEMICAL THERMODYNAMICS
Similar Questions
A certain endothermic reaction: A→ Product, ΔH=+ve proceeds ina sequence of three elementary steps with the rate constant K1,K2 and K3 and each one having energy of activation E1,E2 and E3 respectively at 25∘C. The observed rate constannt for the reaction is equal to K3√K1K2.
A1,A2 and A3 are Arrhenius parameters respectively.
The observed energy of activation for the reaction is05:03View SolutionMatch the following columns
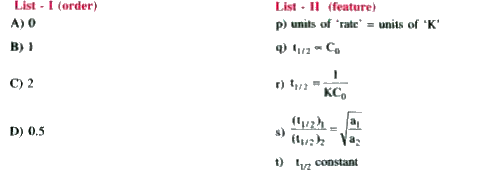 02:49View Solution
02:49View SolutionSucrose decompose in acid solution into glucose and fructose according to the first order rate law, with t12=3.00 hours. What fraction of sample of sucrose remains after 8 hours ?
02:05View SolutionDiscuss on the kinetic spontaneity of a chemical reaction. Is a slow reaction spontaneous? Why?
01:38View SolutionWhich among the following is not an exact differential?
01:10View SolutionFor the reactio 2N2O5→4NO2+O2
The concentration of N2O5 changes from 3.0 M to 2.75 M in 30 minute. Calculate rate of formation of NO201:40View SolutionThe decomposition of N2O5 takes place according to I order as: 2N2O5→4NO2+O2
Calculate:
(a) The rate constant, if instantaneous rate is 1.4×10−6mollitre−1sec−1 when concentration of N2O5 is 0.04M.
(b) The rate of reaction when concentration of N2O5 is 1.20M.
(c ) The concentration of N2O5 when the rate of reaction will be 2.45×10−5mollitre−1sec−101:44View SolutionThe rate constant for the reaction:
2N2O5→4NO2+O2 is 3.0×10−5sec−1. If the rate is 2.40×10−5Msec−1, then the concentration of N2O5 (in M) is:01:07View SolutionFor the reaction 2N2O5→4NO2+O2 rate of reaction and rate constant are 1.02×10−4 and 3.4×10−5sec−1 respectively. The concentration of N2O5 at that time will be
02:17View SolutionConsider the following reaction
2N2O5⇔4NO2+O2.
If rate and rate constant for above reaction are 2.40×10−5 mol L−1s−1 and 3×10−5s−1 respectively, then calculate the concentration of N2O5.03:22View SolutionFor the reaction, 2N2O5→4NO2+O2 rate and rate constant are 1.02×10−4Msec−1 and 3.4×10−5sec−1 respectively, the concentration of N2O5, at that time will be
02:11View SolutionThe rate constant for the reaction, 2N2O5→4NO2+O2 is 3.0×10−5s−1. If the rate is 2.40×10−5molL−1s−1, then the initial concentration of N2O5 (in molL−1) is
02:45View Solution2N_(2)O_(5)to4NO_(2)+O_(2) . The rate of reaction in terms of N_(2)O_(5) will be
01:40View SolutionThe rate constant for the reaction 2N_(2)O_(5)to4NO_(2)+O_(2), "is"" " 3.0xx10^(-5)S^(-1) . If the rate is 2.40xx10^(-5)"mol"L^(-1)s^(-1) then the concentration of N_(2)O_(5)("in mol" L^(-1)) is
05:02View SolutionFor the reaction 2N2O5→4NO2+O2 rate and rate constant are 1.22×10−4and3.4×10−5S−1 respectively then the concentration of N2O5 at that time will be
02:23View Solution

