In a gaseous phase reaction A2(g)→B(t)+1(1/2)C(g), the increase in pressure from 100 mm to 120 mm is noticed in 5 minute.The rate of disappearance of A2 in mm min−1 is Solution in Telugu
The correct Answer is:8
A2→B+12C
100 0 2
(100−x) x x2
60 40 20
⇒100−x+x+x2=120,rA2=d(PA2)dt=100−605=405=8
Topper's Solved these Questions
- BOOK - AAKASH SERIESCHAPTER - CHEMICAL KINETICSEXERCISE - PRACTICE SHEET-2 (SINGLE OR MORE THAN ONE OPTION QUESTIONS)16videos
CHEMICAL KINETICS
- BOOK - AAKASH SERIESCHAPTER - CHEMICAL KINETICSEXERCISE - PRACTICE SHEET-2 (LINKED COMPRHENSION TYPE QUESTIONS)6videos
CHEMICAL KINETICS
- BOOK - AAKASH SERIESCHAPTER - CHEMICAL KINETICSEXERCISE - PRACTICE SHEET-1 (MATCH THE FOLLOWING QUESTIONS)2videos
CHEMICAL KINETICS
- BOOK - AAKASH SERIESCHAPTER - CHEMICAL KINETCSEXERCISE - EXERCISE - 3.245videos
CHEMICAL KINETCS
- BOOK - AAKASH SERIESCHAPTER - CHEMICAL THERMODYNAMICSEXERCISE - Additional Practice Exercise54videos
CHEMICAL THERMODYNAMICS
Similar Questions
The rate constant (K) for the reactio. 2A+B→ Products was found to be
2.5×10−5litremol−1sec−1 after 15 sec, 2.60×10−5 litre mol−1sec−1 after 30 sec and 2.55×10−5 litre "mol"^(-1)"sec"^(-1) after 50 sec. The order of reaction is03:30View SolutionElemantary unimolecular reactions have first order rate laws, elementary bimolecular reactions have second order rate laws. A rate law is often derived from a proposed mechanism by imposing the state approximation or by assuming that there is a pre -equilibrium. A proposed mechanism must be consistent with the experiment rate law.
The decomposition of O3 obeys the mechanism give below
Step 1: O3⇔O2+(O) (both forward and backward reactions are fast)
Step 2: O3+(O)slow−−→O2+O2 (ignore) backward reaction), the rate of reaction is given by03:33View SolutionMatch the following columns
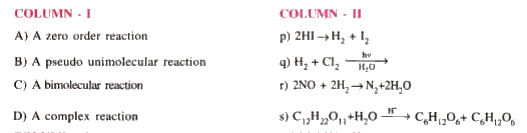 02:12View Solution
02:12View SolutionDiscuss on the kinetic spontaneity of a chemical reaction. Is a slow reaction spontaneous? Why?
02:12View SolutionWhich among the following is not an exact differential?
01:32View SolutionA gaseous reaction A_(2)(g) rarr B(g) + (1)/(2) C(g) shows increase in pressure form 100 mm to 120 mm in 5 min . What is the rate of disappearance of A_(2) ?
05:49View SolutionThe reaction M_(2)(g) rarrN(g)+(1)/(2)R(g) shows increase in pressure from 100 mm to 120 mm in 5 minutes. The rate of reaction is ------- mm min^(-1)
02:13View SolutionA gaseous A2(g)→B(g)+12C(g), shows increase in pressure from 100 mm to 120 mm in 5 min. The rate of disappearance of A2 is
02:13View SolutionA gaseous A_(2)(g) rarr B(g) +(1)/(2) C(g) , shows increase in pressure from 100 mm to 120 mm in 5 min. The rate of disappearance of A_(2) is
03:30View SolutionA gaseous reaction 2A(g) to B(g)+5C(g) shows increase in pressure from 80 mm of Hg to 100 mm of Hg in 5 minutes. The rate of disappearance of A is:
02:00View SolutionA(g)rarrB(g)+C(g) -(d[A])/(dt)=k[A] At the start, pressure is 100 mm and after 10 min, pressure is 120 mm. Hence, rate constant ("min"^(-1)) is :
05:15View SolutionIn gaseous state reaction, A_(2)(g)rarrB(g)+((1)/(2))C(g) . The increase in pressure from 100 mm to 120 mm is noticed in 5 minutes. The rate of disappearance of A_(2) "in mm min"^(-1) is :
02:14View SolutionIn gaseous state reaction, A2(g)→B(g)+(12)C(g). The increase in pressure from 100 mm to 120 mm is noticed in 5 minutes. The rate of disappearance of A2in mm min−1 is :
02:14View SolutionA gaseous reaction A2(g)→B(g)+12C(g) shows increase in pressure form 100mm to 120mm in 5min. What is the rate of disappearance of A2 ?
02:13View SolutionA2B is an ideal gas , which decompase according f to the equation : A2B→A2+12B2 . At start , the initial pressure is 100 mm Hg and after 5 minutes , the pressure is 120 mm Hg. What is the average rate of decomposition of A2B ? Assume T and V are constant .
02:26View Solution

