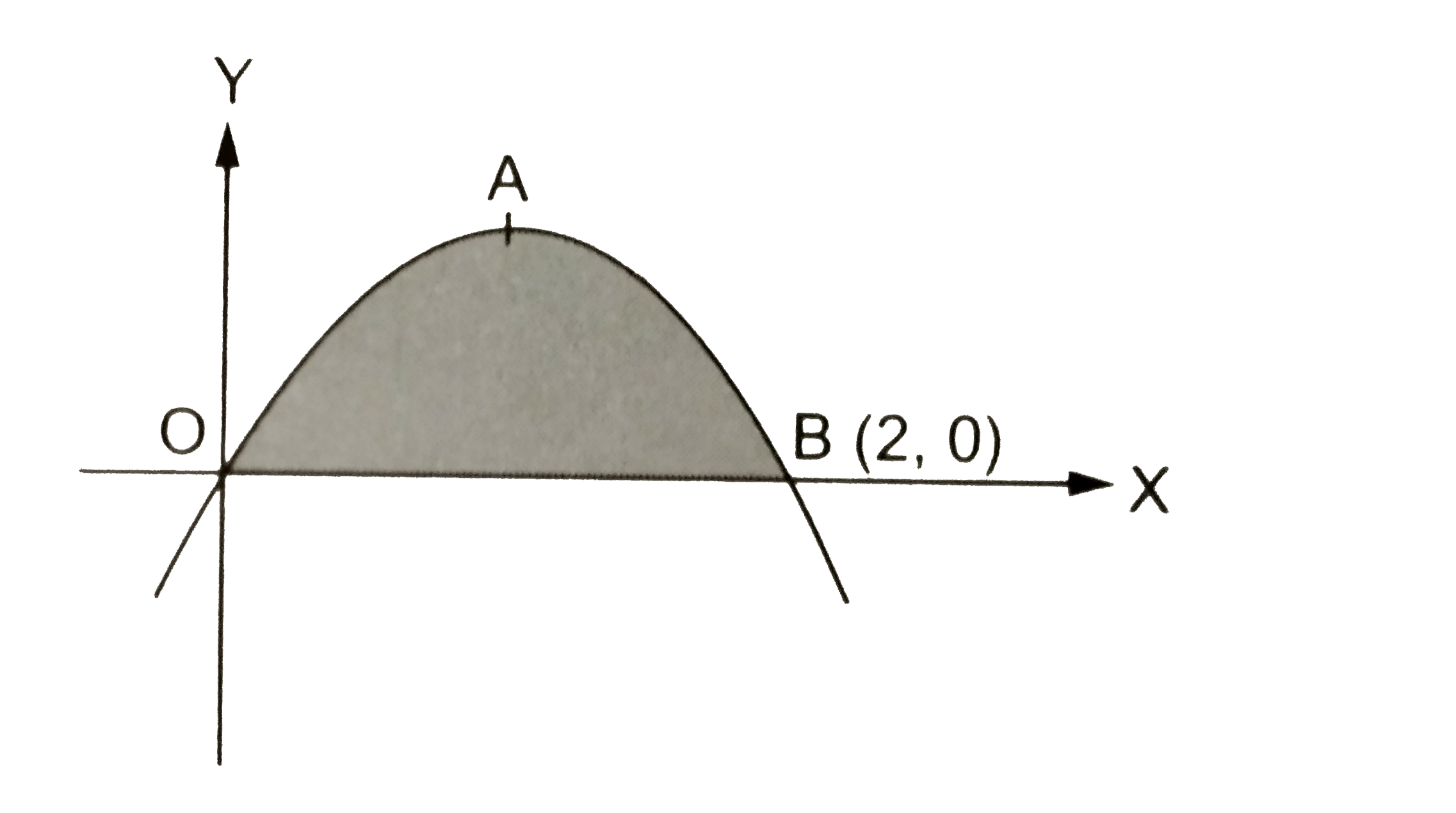
Find by integration the area of the region bounded by the curve $y = 2 x - x^{2}$ and the $x$ -axis.

Video Solution

Text Solution

Verified by Experts

The given curve is $y = 2 x - x^{2}$
Now $y = 2 x - x^{2} \Rightarrow (x^{2} - 2 x + 1) = (- y + 1)$
$\Rightarrow {(x - 1)}^{2} = - 1 (y - 1)$
$\Rightarrow X^{2} = - y$

where $(x - 1) = X and (y - 1) = y$
Clearly , $x^{2} = - y$ is a downward parabola with its vertex at $(X = 0, Y = 0) .$
Now , $X = 0, Y = 0 \Rightarrow x - 1 = 0 and y - 1 = 0$
$\Rightarrow x = 1 and y = 1$
Thus the vertex of the parabola is $A (1, 1)$
Also $y = 0 \Rightarrow 2 x - x^{2} = 0 \Rightarrow x (2 - x) = 0 \Rightarrow x = 0 or x = 2$
Thus, the curve the x-axist at $O (0, 0) and B (2, 0)$
The rough sketch of the curve can now be drawn as shown in the given figure
$∴$ required area $= 2 \int 0 y d x$
$= 2 \int 0 (2 x - x^{2}) d x = {[x^{2} - \frac{x^{3}}{3}]}_{0}^{2}$
$= (4 - \frac{8}{3}) = \frac{4}{3}$ sq units
Hence the required area is $\frac{4}{3}$ sq units .

The area of the region bounded by the curve $y = 2 x - x^{2}$ and x - axis is
A $\frac{2}{3}$ sq , units
B $\frac{4}{3}$ sq,units
C $\frac{5}{3}$ sq , units
D $\frac{8}{3}$ sq. units
The area of the region bounded by the curve $y = 2 x - x^{2}$ and X- axis is
A $\frac{2}{3}$ sq.units
B $\frac{4}{3}$ sq.units
C $\frac{5}{3}$ sq.units
D $\frac{8}{3}$ sq.units
Area of the region bounded by the curve $y = x^{2} - 5 x + 4$ and the X-axis is
A $\frac{3}{2}$
B $\frac{5}{2}$
C $\frac{7}{2}$
D $\frac{9}{2}$