If $λ_{1}, λ_{2} and λ_{3}$ are the wavelengths of the wave giving resonance with the fundamental, first and second overtones respectively of a closed orga pipe Then the ratio of wavelength $λ_{1}, λ_{2} and λ_{3}$ is

A

$1 : 3 : 5$

B

$1 : 2 : 3$

C

$5 : 3 : 1$

D

$1 : \frac{1}{3} : \frac{1}{5}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

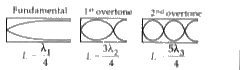
As is clear from figure
$L = \frac{λ_{1}}{4} or λ_{1} = 4 L, L = \frac{3 λ_{2}}{4} or λ_{2} = \frac{4 L}{3}$
$L = \frac{5 λ_{3}}{4} or λ_{3} = \frac{4 L}{5} ∴ λ_{1} : λ_{2} : λ_{3} = 1 : \frac{1}{3} : \frac{1}{5}$

|

Updated on:21/07/2023

Class 11 PHYSICS SIMPLE HARMONIC MOTION

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
If $λ_{1}, λ_{2}, λ_{3}$ are the wavelengths of the waves giving resonance in the fundamental, first and second overtone modes respecively in a open organ pipe, then the ratio of the wavelengths $λ_{1} : λ_{2} : λ_{3}$ , is :
A $1 : 2 : 3$
B $1 : 3 : 5$
C $1 : 1 / 2 : 1 / 3$
D $1 : 1 / 3 : 1 / 5$
Question 2 - Select One
If $λ_{1}, λ_{2}, λ_{3}$ are the wavelengths of the waves giving resonance in the fundamental, first and second overtone modes respecively in a open organ pipe, then the ratio of the wavelengths $λ_{1} : λ_{2} : λ_{3}$ , is :
A $1 : 2 : 3$
B $1 : 3 : 5$
C $1 : 1 / 2 : 1 / 3$
D $1 : 1 / 3 : 1 / 5$
Question 3 - Select One
If $λ_{1}, λ_{2} and λ_{3}$ are the wavelength of the waves giving resonance to the fundamental, first and second overtone modes respectively in a string fixed at both ends. The ratio of the wavelengths $λ_{1} : λ_{2} : λ_{3}$ is
A $1 : 2 : 3$
B $1 : 3 : 5$
C $1 : \frac{1}{2} : \frac{1}{3}$
D $1 : \frac{1}{3} : \frac{1}{5}$

MTG GUIDE-OSCILLATIONS AND WAVES -NEET CAFÉ TOPICWISE PRACTICE QUESTIONS

Home
Ask Doubt
Profile