A solenoid S1 is placed coaxially inside another solenoid S2 . The radii of the inner and outer solenoids are r1 and r2 respectively. If N1 and N2 are the number of turns of coil in solenoid S1 and S2 respectively and l is the length of solenoid S2 carrying current I , then calculate the mutual inductance between the two solenoids.
Magnetic field of the outer solenoid is B=μ0N2Il , when current I passes through it
The flux associated with the inner coil is ϕ1=N1Bπr21
⇒ϕ1=N1(μ0N2l)Iπr21
∴ Mutual inductance between the two solenoid is given by M=ϕ1I=(μ0N1N2l)πr21
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTION AND ALTERNATING CURRENTSBOOK - MTG GUIDECHAPTER - ELECTROMAGNETIC INDUCTION AND ALTERNATING CURRENTSEXERCISE - NEET CAFE TOPICWISE PRATICE QUESTIONS (MAGNETIC FLUX)
1 Videos
- ELECTROMAGNETIC INDUCTION AND ALTERNATING CURRENTSBOOK - MTG GUIDECHAPTER - ELECTROMAGNETIC INDUCTION AND ALTERNATING CURRENTSEXERCISE - NEET CAFE TOPICWISE PRATICE QUESTIONS (FARADAY.S LAW (INDUCED EMF AND CURRENT)
27 Videos
- DUAL NATURE OF MATTER AND RADIATION BOOK - MTG GUIDECHAPTER - DUAL NATURE OF MATTER AND RADIATION EXERCISE - AIPMT/ NEET MCQs
30 Videos
- ELECTROMAGNETIC WAVESBOOK - MTG GUIDECHAPTER - ELECTROMAGNETIC WAVESEXERCISE - AIPMT/NEET(MCQs)
15 Videos
Similar Questions
A solenoid S1 is placed inside another solenoid S2 as shown in the fig. The radii of the inner and the outer solenoids are r1 and r2 respectively and the numbers of turns per unit length are n1 and n2 respectively. Consider a length I of each solenoid. Calculate the mutual inductance between them.
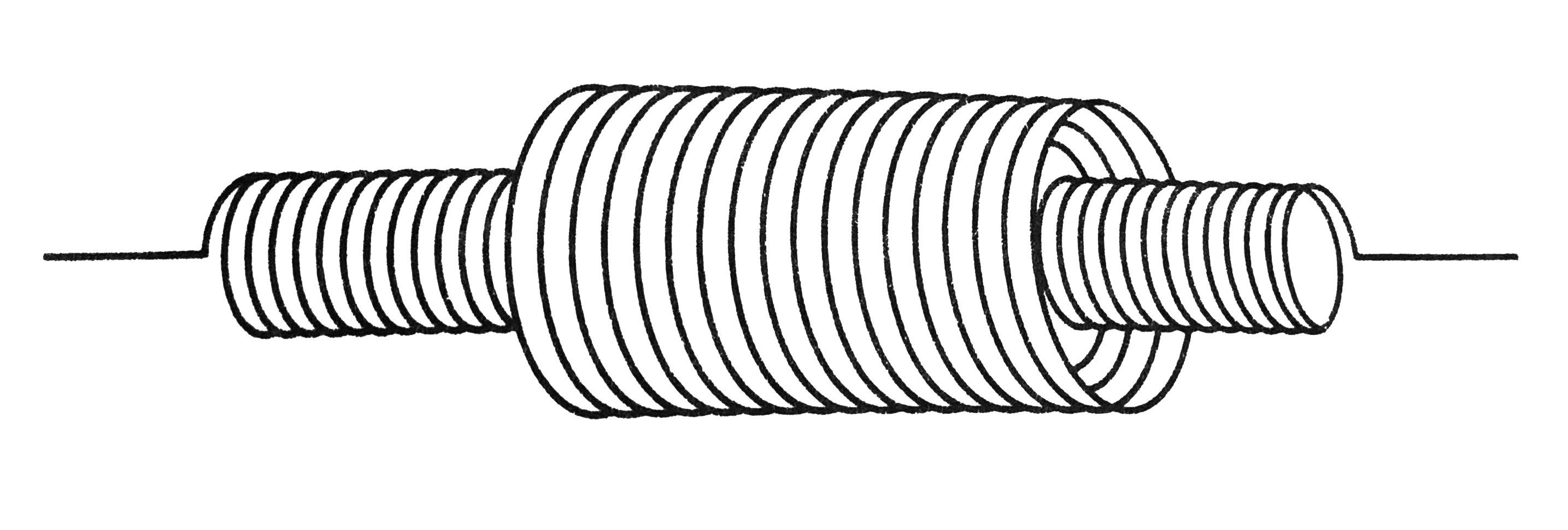
View SolutionThere are two long co-axial solenoids of same length l. The inner and other coils have redii r1 and r2 and number of turns per unti length n1 and n2 respectively .The reatio of mutual inductance to the self - inductance of the inner- coil is :
View Solution
Knowledge Check
A solenoid of inductance 2 H carries a current 1 A. what is the magnetic energy stored in a solenoid?
A2JB1JC4JD5JA long solenoid has 800 turns per metre length of solenoid. A current of 1.6 A flows through it. The magnetic induction at the end of the solenoid on its axis is
A16×10−4TB8×10−4TC32×10−4TD4×10−4TA long solenoid has 800 turns per metre length of solenoid A current of 1.6 A flow through it. The magnetic induction at the end of the solenoid on its axis is
A16×10−4 teslaB8×10−4 teslaC32×10−4 teslaD4×10−4 tesla
There are two long co-axial solenoids of same length l. The inner and other coils have redii r1 and r2 and number of turns per unti length n1 and n2 respectively .The reatio of mutual inductance to the self - inductance of the inner- coil is :
View SolutionA solenoid of turns carries current I while another -solenoid of same length and of 3 n turns pa.rries cur reot 4I. What is the ratio of the magnetic strengths of the two solenoids?
View SolutionAn e.m.f of 40 mV is induced in a solenoid, when the current in it changes at the rate of 2A/s. The self inductance of the solenoid is
View SolutionA solenoid is placed inside another solenoid, the length of both being equal carrying same magnitude of current. The other parameters like radius and number of turns are in the ratio 1:2 for the two solenoids. The mutual inductance on each other would be
View SolutionA solenoid is placed inside another solenoid, the length of both being equal carrying same magnitude of current. The parameters like radius and number of turns are in the ratio 1:2 for the two solenoids. The mutual inductance on each other would be
View Solution
