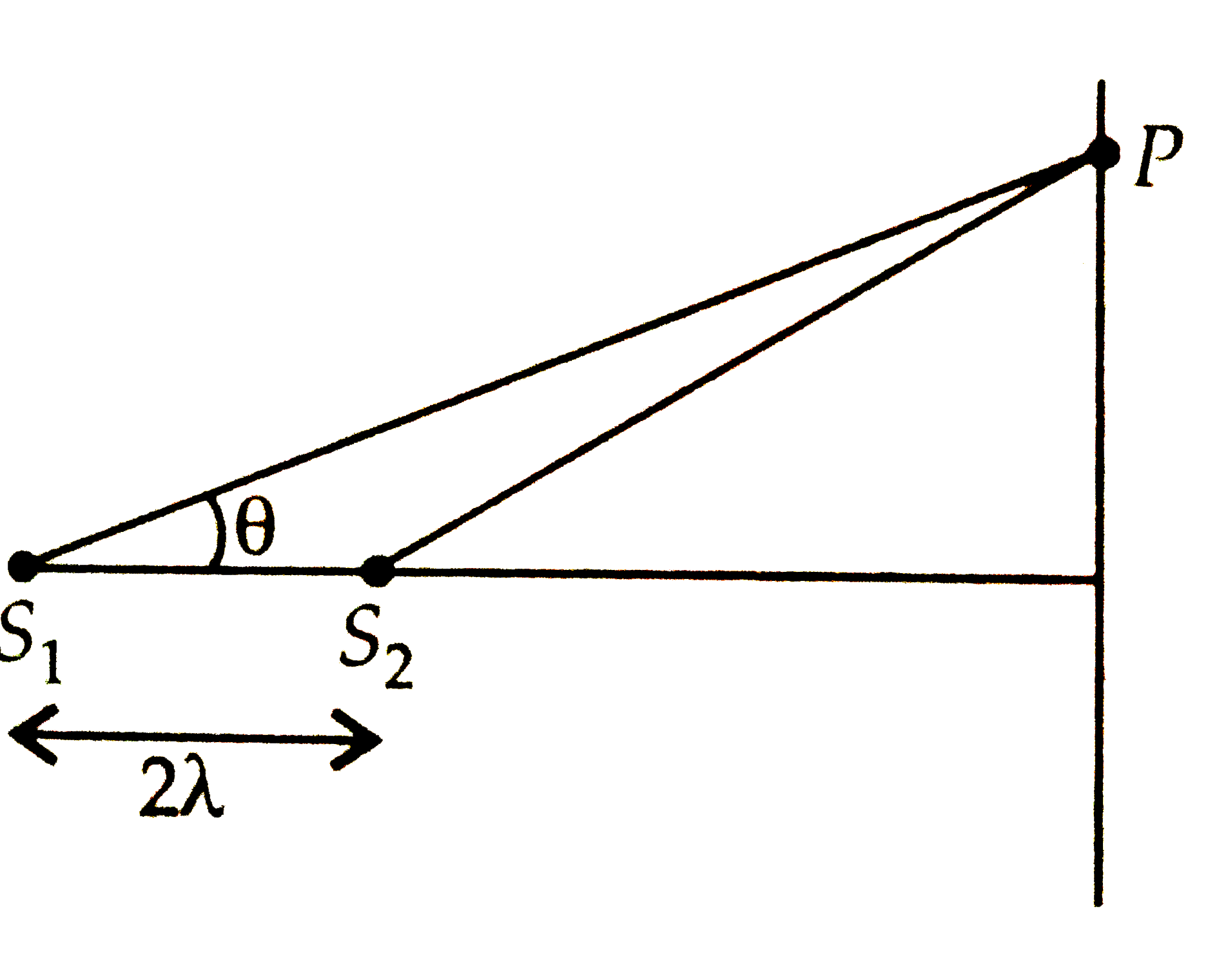
In Young's double slit experiment, the slits are horizontal. The intensity at a point P as shown in figure is $\frac{3}{4} I_{0}$ ,where $I_{0}$ is the maximum intensity.
Then the value of $θ$ is,
(Given the distance between the two slits $S_{1}$ and $S_{2}$ is $2 λ$ )

${cos}^{- 1} (\frac{1}{12})$

${sin}^{- 1} (\frac{1}{12})$

${tan}^{- 1} (\frac{1}{12})$

${sin}^{- 1} (\frac{3}{5})$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

Updated on:21/07/2023

In Young's double slit experiment intensity at a point is $(\frac{1}{4})$ of the maximum intersity. Angular position of this point is
A ${sin}^{- 1} (\frac{λ}{d})$
B ${sin}^{- 1} (\frac{λ}{2 d})$
C ${sin}^{- 1} (\frac{λ}{3 d})$
D ${sin}^{- 1} (\frac{λ}{4 d})$
In a Young's double slit experiment the amplitudes of the two waves incident on the two slits are $A$ and $2 A$ . If $I_{0}$ is the maximum intensity, then the intensity at a spot on the screen where the phase difference between the two interfering waves is $ϕ$ .
A $I_{0} {cos}^{2} (ϕ / 2)$
B $\frac{I_{0}}{3} {sin}^{2} (ϕ / 2)$
C $(\frac{I_{0}}{9} (5 + 4 cos (ϕ))$
D $\frac{I_{0}}{9} (5 + 8 cos (ϕ))$
In Young's double-slit experiment, the intensity at a point P on the screen is half the maximum intensity in the interference pattern. If the wavelength of light used is $λ$ and d is the distance between the slits, the angular separation between point P and the center of the screen is
A ${sin}^{- 1} (\frac{λ}{d})$
B ${sin}^{- 1} (\frac{λ}{2 d})$
C ${sin}^{- 1} (\frac{λ}{3 d})$
D ${sin}^{- 1} (\frac{λ}{4 d})$