A mixture consists of two radioactive materials A1 and A2 with half-lives of 20s and 10s respectively. Initially the mixture has 40g of A1 and 160g of a2. The amount the two in the mixture will become equal after
60 s
80 s
20 s
40 s
Let after t s amount of the A1andA2 will become equal in the mixture.
As N=N0(12)n where n is the number of half - lives
For A1,N1=N01(12)t/20
For A2,N2=N02(12)t/10
According to question, N1=N2, so, 402t/20=1602t/10
2t/10P=4(2t/20)or2t/10=222t/20
2t/10=2(t20+2)
t10=t20+2ort10−120=2ort20=2ort=40s
Topper's Solved these Questions
Similar Questions
In a radioactive decay processthe activity is defined as A = - (dN)/dtwhereN(t)istheνmberofradioactiveνc≤iattimet.Tworadioactivesources,S_1andS_2havesameactivityattimet=0.Atalatertime,theactivitiesofS_1andS_2areA_1andA_2,respectively.WhenS_1andS_2havejustcomp≤tedtheir3rdand7thhalf−lives,respectively,theratioA_1/A_2 is.
View Solution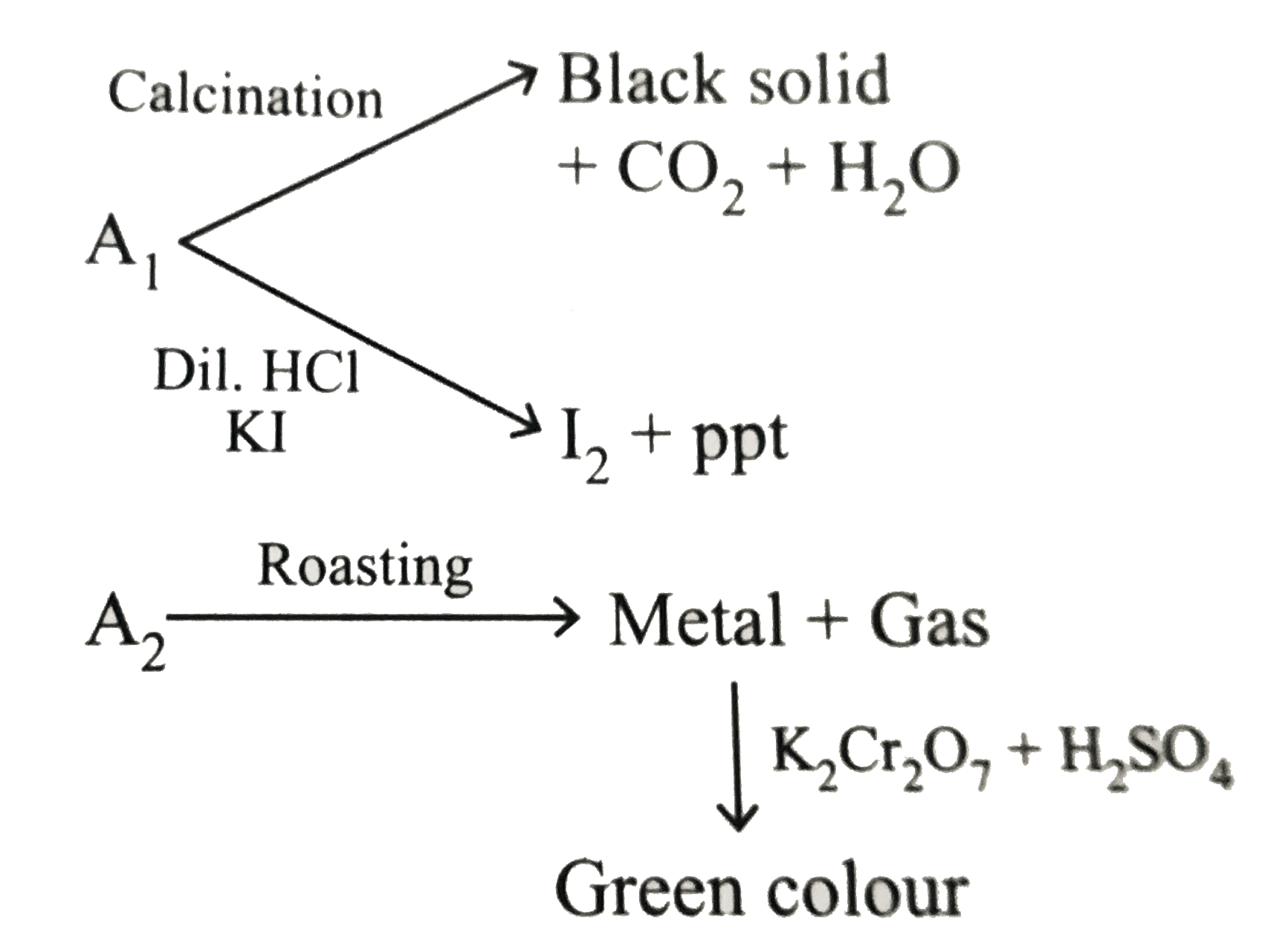
A1 and A2 are two ores of metal M.A1 on calcination gives black precipitate, CO2 and water. Identify A1 and A2.View Solution
Knowledge Check
- Question 1 - Select One
Atomic masses of two heavy atoms are A1 and A2. Ratio of their respective nuclear densities will be approximately
A(a) A1A2B(b) (A1A2)13C(c) (A2A1)13D(d) 1 - Question 2 - Select One
The decay constant of a radioactive isotope is λ. If A1 and A2 are its activities at time t1 and t2 respectively, then the number of nuclei which have decayed the time (t1−t2)
AA1t1−A2t2BA1−A2C(A1−A2)/λDλ(A1−A2) - Question 3 - Select One
The ratio of nuclear density of two nuclei having mass number A1=12 and A2=14 is
A6:7B7:6C1:1D1:2
The pKa1 and pKa2 of an amino acid are 2.3 and 9.7 respectively. The isoelectric point of the amino acid is:
View SolutionTwo concentric conducting spherical shells of radii a1anda2(a2>a1) are charged to potentials ϕ1 and ϕ2, respectively. Find the charge on the inner shell.
View SolutionTwo wires of different materials of resistivity p1andp2 , length l1andl2, and area of cross-section A1andA2 respectively are connected in parallel. The ratio of current density in the two wires,j1j2 , is given by:
View SolutionThe activation energies of two reactions are Ea1 and Ea2 with Ea1>Ea2. If the temperature of the reacting systems is increased from T to T', which of the following is correct?
View SolutionThe activation energies of two reactions are Ea1 and Ea2 with Ea1>Ea2. If the temperature of the reacting systems is increased from T to T', which of the following is correct?
View Solution

