A magnetic field
always exerts a force on a charged par ticle
never exerts a force on a charged particle
exerts a force, if the charged particle is moving along the magnetic field lines
exerts a force, if the charged particle is moving across the magnetic field lines
The correct Answer is:D
To solve the question regarding the behavior of a charged particle in a magnetic field, we need to analyze the options provided based on the principles of electromagnetism.
1. Understanding the Magnetic Force on a Charged Particle:
The force F on a charged particle moving in a magnetic field is given by the formula:
F=Q⋅V⋅B⋅sin(θ)
where:
- F is the magnetic force,
- Q is the charge of the particle,
- V is the velocity of the particle,
- B is the magnetic field strength,
- θ is the angle between the velocity vector and the magnetic field direction.
2. Analyzing Each Option:
- Option 1: "A magnetic field always exerts a force on a charged particle."
- This is incorrect because if the charged particle is at rest (V=0) or moving parallel to the magnetic field lines (θ=0 or 180∘), then F=0.
- Option 2: "A magnetic field never exerts a force on a charged particle."
- This is also incorrect because a magnetic field can exert a force on a charged particle when it is moving at an angle to the magnetic field lines (0<θ<180∘).
- Option 3: "A magnetic field exerts a force on a charged particle if it is moving along the magnetic field lines."
- This is incorrect as well. If the charged particle is moving along the magnetic field lines (θ=0), the force F will be zero since sin(0)=0.
- Option 4: "A magnetic field exerts a force if the charged particle is moving across the magnetic field."
- This is correct. When the charged particle moves across the magnetic field lines, θ is not 0 or 180∘, thus sin(θ) is non-zero, and a force will be exerted on the particle.
3. Conclusion:
Based on the analysis of the options, the correct answer is:
Option 4: A magnetic field exerts a force if the charged particle is moving across the magnetic field.
The force F on a charged particle moving in a magnetic field is given by the formula:
F=Q⋅V⋅B⋅sin(θ)
where:
- F is the magnetic force,
- Q is the charge of the particle,
- V is the velocity of the particle,
- B is the magnetic field strength,
- θ is the angle between the velocity vector and the magnetic field direction.
- Option 1: "A magnetic field always exerts a force on a charged particle."
- This is incorrect because if the charged particle is at rest (V=0) or moving parallel to the magnetic field lines (θ=0 or 180∘), then F=0.
- This is also incorrect because a magnetic field can exert a force on a charged particle when it is moving at an angle to the magnetic field lines (0<θ<180∘).
- This is incorrect as well. If the charged particle is moving along the magnetic field lines (θ=0), the force F will be zero since sin(0)=0.
- This is correct. When the charged particle moves across the magnetic field lines, θ is not 0 or 180∘, thus sin(θ) is non-zero, and a force will be exerted on the particle.
Based on the analysis of the options, the correct answer is:
Option 4: A magnetic field exerts a force if the charged particle is moving across the magnetic field.
Topper's Solved these Questions
- HEATING AND MAGNETIC EFFECTS OF CURRENT BOOK - MCGROW HILL PUBLICATIONCHAPTER - HEATING AND MAGNETIC EFFECTS OF CURRENT EXERCISE - HIGHER ORDER THINKING QUESTIONS
34 Videos
- HEATBOOK - MCGROW HILL PUBLICATIONCHAPTER - HEATEXERCISE - HIGHER ORDER THINKING QUESTIONS
15 Videos
- LAWS OF MOTION, FRICTION AND ARCHIMEDES' PRINCIPLEBOOK - MCGROW HILL PUBLICATIONCHAPTER - LAWS OF MOTION, FRICTION AND ARCHIMEDES' PRINCIPLEEXERCISE - HIGHER ORDER THINKING QUESTIONS
34 Videos
Similar Questions
Knowledge Check
Magnetic field lines are closer where the magnetic field is
AstrongBweakCsometimes strong and sometimes weakDnone of theseMagnetic field lines are shown closer together, where the magnetic field is
AstrongerBweakCconstantDNone of theseA charged particle enters a uniform magnetic field perpendicular to it. The magnetic field
Aincreases the K.E. of the particleBdecreases the K.E. of the particleCchanges the direction of motion of the particleDdoes both (a) and ( c)
What are magnetic field lines? How is the direction of magnetic field at a point determined? Mention two important properties of magnetic field lines.
View SolutionMagnet (pole strength, magnetic dipole and magnetic dipole moment)|Magnetic field and strength of magnetic field |Due to a single pole, due to a bar magnet |Magnet in an external uniform magnetic field
View SolutionMagnetic axis |magnetic length |magnetic field lines and its properties | magnetic field intensity
View SolutionA magnetic of magnetic moment placed along the X-axis in an magnet field .Find the torque acting on the magnetic field
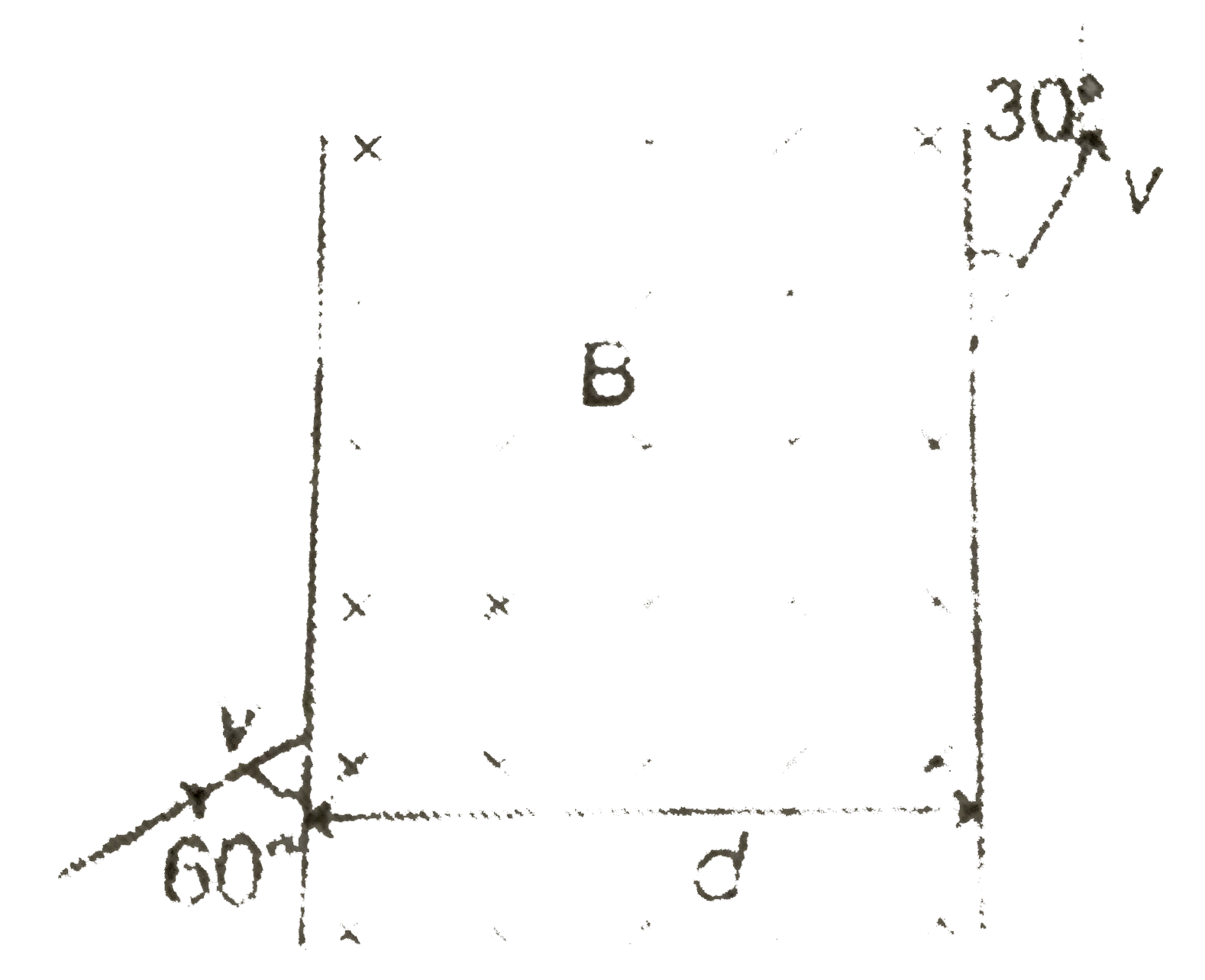
View SolutionA charge particle of charge 'q' and mass 'm' enters in a given magnetic field 'B' and perpendicular to the magnetic field as shown in the figure. It enters at an angle of 60∘ with the boundary surface of magnetic field and comes out at an angle of 30∘ with the boundary surface of the magnetic field as shown in the figure. Find width 'd' in which magnetic field exist.
View Solution
