PA and PB are the vapour pressure of pure liquid components. A and B, respectively of an ideal binary solution. If xA represents the mole fraction of component A, the total pressure of the solution will be Solution in Telugu
PB+xA(PB−PA)
PA+xA(PA−PB)
PA+xA(PB−PA)
PB+xA(PA−PB)
The correct Answer is:D
Step by step video, text & image solution for P_A and P_B are the vapour pressure of pure liquid components. A and B, respectively of an ideal binary solution. If x_A represents the mole fraction of component A, the total pressure of the solution will be by Chemistry experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - AAKASH SERIESCHAPTER - SOLUTIONS EXERCISE - Objective Exercise -450videos
SOLUTIONS
- BOOK - AAKASH SERIESCHAPTER - SOLUTIONS EXERCISE - Objective Exercise -2110videos
SOLUTIONS
- BOOK - AAKASH SERIESCHAPTER - SOLIDS STATEEXERCISE - PRACTICE EXERCISE63videos
SOLIDS STATE
- BOOK - AAKASH SERIESCHAPTER - STATES OF MATTER EXERCISE - ADDITIONAL PRACTICE EXERCISE -PRACTICE SHEET (ADVANCED) (LINKED COMPREHENSION TYPE QUESTIONS) (PASSAGE- IV)2videos
STATES OF MATTER
Similar Questions
Assertion : Molarity of a solution decreases with an increase of temperature
Reason : As temperature increases volume of the solution increases.01:36View SolutionThe number of Glucose molecules present in 10 ml of decimolar solution is
View SolutionWhich of the following is considered as true amorphous solid
02:14View SolutionAt a particular temperature and pressure for a real gas Van der Waal's equation can be written as:
(P+aV2m)(Vm−b)=RT
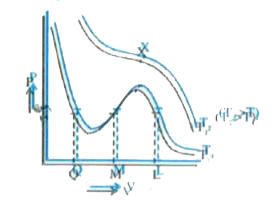
where Vm is molar volume of gas. This is cubic equation in the variable Vm and therefore for any single value of P & T there should be 3 values of Vm. Which are shown in graph as Q, M and L.
As temperature is made to increase at a certain higher temperature the three values of Vm becomes identical. The temperature, pressure & molar volume at point X are called Tc, Pc & Vc for real gas. The compressibility factor in terms of Pc, Vc and T is called Zc.
The expression of Van dcr Waal's constant 'a' can be given as02:49View SolutionMole fraction of component A in vapour phase is χ1 and that of component A in liquid mixture is χ2, then (p∘A)= vapour pressure of pure A, p∘B = vapour pressure of pure B), the total vapour pressure of liquid mixture is
02:49View SolutionFor a binary liquid solution of A and B. P_A^0 =pure vapour pressure of A . P_B^0 =pure vapour pressure of B. X_A =mole fraction of A in liquid phase. Y_A =mole fraction of A in vapour phase. {:("Column I","Column II"),((A)P_A^0gtP_B^0"[Ideal liquid solution]",(p)X_A=Y_A),((B)"Azeotropic mixture",(q)X_AltY_A),(( C)"Equimolar ideal mixture of A & B with "P_A^0ltP_B^0,(r)X_BltY_B),((D)"Equimolar ideal mixture of A & B with "P_A^0=P_B^0,(s)Y_BgtY_A),(,(t)X_B=Y_B):}
View SolutionIf x_(1) and x_(2) represent the mole fraction of a component A in the vapour phase and liquid mixture respectively and p_(A)^(@) and p_(B)^(@) represent vapours pressures of pure A and pure B. then total vapour pressure of the liquid mixture is
02:32View SolutionP_1 and P_2 are the vapour pressures of pure liquid components , 1 and 2 respectively of an ideal binary solution if x_1 represents the mole Traction of component 1, and the total pressure of the solutions formed by 1 and 2 will be
06:17View SolutionP1 and P2 are the vapour pressures of pure liquid components , 1 and 2 respectively of an ideal binary solution if x1 represents the mole Traction of component 1, and the total pressure of the solutions formed by 1 and 2 will be
01:55View SolutionP_(1) and P_(2) are the vapour pressures of pure liquid components,1 and 2 respectively of an ideal binary solution if x_(1) represents the mole fraction of component 1, the total pressure of the solution formed by 1 and 2 will be…..........
01:55View SolutionIf x1 and x2 represent the mole fraction of a component A in the vapour phase and liquid mixture respectively and p0A and p0B represent vapours pressures of pure A and pure B, then total vapour pressure of the liquid mixture is
06:59View SolutionWith the help of Raoult's law show that total vapour pressure of a binary liquid solution varies linearly with the mole fraction of a component.
01:53View SolutionThe mole fraction of component A in vapour phase is χ1 and mole fraction of component A in liquid mixture is χ2(P∘A=vapour pressure of pure A,P∘B=vapour pressure of pure B).Then total vapour pressure of the liquid mixture is
05:12View SolutionMole fraction of component A in vapour phase is χ1 and that of component A in liquid mixture is χ2, then (p∘A)= vapour pressure of pure A, p∘B = vapour pressure of pure B), the total vapour pressure of liquid mixture is
03:01View SolutionThe total vapour pressure of a binary solution is given by P=(100xA+260xB)mmHg
where xA and xB are the mole fraction of components A and B. This indicates that the :05:37View SolutionThe vapour pressures of pure liquids A and B respectively are 600 torr and 500 torr. In a binary solution of A and B , mole fraction of A is 0.25 then total pressure of the solution (in torr) will be
View Solution

