Match the solid in Column - I with its characteristic in Column - II.
COLUMN - ICOLUMN - II(Solid)(Characteristic)A) NaClp) Body centred cubicB) CsClq) Packing fractionC) Nar) Packing fraction =2π3(r3++r3−)(r++r−)3D) TiCls) Packing fraction √32π(r3++r3−)(r++r−)3Solution in Telugu
The correct Answer is:A−r;mB−p,s;C−pq;D−ps
For NaCl=4×43π(r+−r−)323×(r+−r−)3=23π(r+−r−)3(r+−r−)3=43π(r+−r−)(23)3(r++r−)3=√32π(r+−r−)3(r+−r−)3
Na−BCC, P.F = 0.68, TiCl BCC P.F=√3π2(r+−r−)3(r+−r−)3
Topper's Solved these Questions
- BOOK - AAKASH SERIESCHAPTER - SOLID STATEEXERCISE - LEVEL - II LECTURE SHEET (EXERCISE - IV)4videos
SOLID STATE
- BOOK - AAKASH SERIESCHAPTER - SOLID STATEEXERCISE - PRACTICE SHEET - 130videos
SOLID STATE
- BOOK - AAKASH SERIESCHAPTER - SOLID STATEEXERCISE - LEVEL - II LECTURE SHEET (EXERCISE - II)6videos
SOLID STATE
- BOOK - AAKASH SERIESCHAPTER - REVISION EXERCISE EXERCISE - COMPLEX COMPOUNDS47videos
REVISION EXERCISE
- BOOK - AAKASH SERIESCHAPTER - SOLIDS STATEEXERCISE - PRACTICE EXERCISE63videos
SOLIDS STATE
Similar Questions
How many effective Cl− ions are present in the rock salt NaCl if ions along one axis joining opposite faces are removed?
02:55View SolutionA match box exhibits
10:14View Solution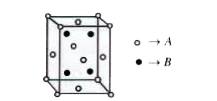
If the molar mass AB is 100gmol−1 and 'a' is edge length then the density of the crystal will be06:32View SolutionIn which of the following complexes the metal ion is in zero oxidation state ?
View SolutionWhich of the following is considered as true amorphous solid
02:28View SolutionIf r_(1),r_(2),r_(3) are the radii of the escribed circlesof a Delta ABC and ifr is the radius of its incircle then r_(1)r_(2)r_(3)-r(r_(1)R_(2)+r_(2)r_(3)+r_(3)r_(1))=
10:10View Solution(r_(3)+r_(1))(r_(3)+r_(2))sin C=2r_(3)sqrt(r_(2)r_(3)+r_(3)r_(1)+r_(1)r_(2))
06:22View Solution(r2+r3)√rr2r2r3=
08:11View SolutionStatement I In a ΔABC,ifa<b<c and ri si inradius and r1,r2+r2r3 are the exradii opposite to angle A,B,C respectively, then r<r1<r2<r3.
Statement II For, ΔABCr1r2+r2r3+r3r1=r1r2r3r01:33View Solutionshow that r2r3+r3r1+r1r2=s2
View SolutionProve that r1(r2+r3)√r1r2+r2r3+r3r1=a
05:30View SolutionStatement-1: ln !ABC,r1+r2+r3−r=4R
Statement-2: In !ABC,r1r2+r2r3+r3r1=!2View SolutionProve the questions
a(rr1+r2r3)=b(rr2+r3r1)=c(rr3+r1r2)02:07View SolutionIf r1,r2,r3,r are in usual notation in ΔABC then r1r2r3−r(r1r2+r2r3+r3r1)=
05:17View SolutionIn a ΔABC, if 2r2r3r2−r1=r3−r1 then
r1(r2+r3)√r1r2+r2r3+r3r1=10:26View Solution

