Two bars of masses m, and m, connected by an light spring (intially at its natural length) rests on a horizontal plane. The coefficient of friction between the bars and the surface is μ. The mnimum costant force that bar to be applied in the horizontal direction (along the length of spring) to the bar of mass m1 in order to shift the other bar of mass m2 Acceleration due to gravity is g) will beSolution in Telugu
μ(m1+m2)g
μ(m1+m22)g
μ(m12+m2)g
μ(m1+m2)g2
The correct Answer is:B
Step by step video, text & image solution for Two bars of masses m, and m, connected by an light spring (intially at its natural length) rests on a horizontal plane. The coefficient of friction between the bars and the surface is mu. The mnimum costant force that bar to be applied in the horizontal direction (along the length of spring) to the bar of mass m_(1) in order to shift the other bar of mass m_(2) Acceleration due to gravity is g) will be by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- FRICTION BOOK - AAKASH SERIESCHAPTER - FRICTION EXERCISE - PRACTICE SHEET (EXERCISE-I) (LEVEL-II) (INTEGER TYPE QUESTIONS)
4 Videos
- FRICTION BOOK - AAKASH SERIESCHAPTER - FRICTION EXERCISE - PRACTICE SHEET EXERCISE-II)(LEVEL-I) (STRAIGHT OBJECTIVE TYPE QUESTIONS)
3 Videos
- FRICTION BOOK - AAKASH SERIESCHAPTER - FRICTION EXERCISE - PRACTICE SHEET (EXERCISE-I) (LEVEL-I) (STRAIGHT OBJECTIVE TYPE QUESTIONS)
12 Videos
- FLUID MECHANICSBOOK - AAKASH SERIESCHAPTER - FLUID MECHANICSEXERCISE - Questions for Descriptive Answers
14 Videos
- GAUSS.S LAW BOOK - AAKASH SERIESCHAPTER - GAUSS.S LAW EXERCISE - PROBLEMS (LEVEL - II)
12 Videos
Similar Questions
Half of the chain is lying on a table. The rest of the chain hangs in equilibrium from both of the ends of the table. Extra length of the hanging chain at one end is 1/8 th of the total length of the chain than that hanging at the other end. The coefficient of friction between table and chain is 25×10−4 the value of x is
View SolutionA horizontal force of 10 N is necessary to just hold a block stationary against a wall. The coefficient of friction between the block and the wall is 0.2. The weight, of the block is:

View SolutionA block of mass 5kg is lying on a rough horizontal surface. The coefficient of static and kinetic friction are 0.3 and 0.1 and g=10ms−2 The frictional force on the block is
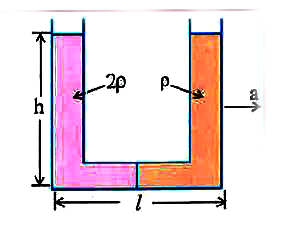
View SolutionA U-tube of base length I filled with same volume of two liquids of densities ρ and 2ρ is moving with an acceleration a on the horizontal plane. If the height difference between the two surfaces (open to atmosphere)becomes zero,then find height h
View SolutionA charge q is placed at the centre of the open end of a cyclindrical vessel. Find the flux of the electric through the surface of the vessel.
View SolutionTwo block of masses m_(1) and m_(2) connected by a light spring rest on a horixontal plane. The cofficient of friction between the block and the surface is equal to mu . What minimum constant force has to be applied in the horizontal direction to the block of mass m_(1) in order to shift the other block?
View SolutionTwo bars of masses m_1 and m_2 connected by a weightless spring of stiffness k , rest on a smooth horizontal plane. Bar 2 is shifted by a small distance x_0 to the left and released. The veloicyt of the centre of mass of the system when bar 1 breaks off the wall is
View SolutionA plank of mass m1 with a bar of mass m2 placed on it lies on a smooth horizontal plane. A horizontal force growing with time t as F′=at (a is constant) is applied to the bar. Find how the accelerations of the plank w1 and of the bar w2 depend on t, if the coefficient of friction between the plank and the bar is equal to k. Draw the approximate plots of these dependences.
View SolutionTwo bars of masses m1 and m2 connected by a non-deformed light spring rest on a horizontal plane. The coefficient of friction between the bars and the surface is equal to k. What minimum constant force has to be applied in the horizontal direction to the bar of mass m1 in order to shift the other bar?
View SolutionTwo bars connected by a weightless spring of stiffness ϰ and length (in the non-deformed state) l_0 rest on a horizontal plane. A constant horizontal force F starts acting on one of the bars as shown in figure. Find the maximum and minimum distances between the bars during the subsequent motion of the system, if the masses of the bars are: (a) equal: (b) equal to m_1 and m_2 , and the force F is applied to the bar of mass m_2 .
View SolutionTwo bars of masses m_(1) and m_(2) connected by a non - deformed light spring rest on a horizontal plane. The coefficient of friction between the bars and the surface is equal to mu . What minimum constant force has to be appled in the horizontal direction to the bar of mass m_(1) in order to shift the other bar?
View SolutionA bar of mass m is suspended horizontally on two vertical springs of spring constant k and 3k . The bar bounces up and down while remaining horizontal. Find the time period of oscillation of the bar neglect mass of springs and friction everywhere
View SolutionA bar of mass m_(1) is placed on a plank of mass m_(2) which rests on a smooth horizontal plane . The coefficient of friction between the surfaces of bar and plank is k . The plank is subjected to a horizontal force F depending on time t as F = at , where a is a constant . The moment of time t_(0) at which the plank starts sliding is :
View SolutionTwo blocks of masses M1 and M2 connected by a light spring rest on a horizontal plane . The coefficient of friction between the blocks and the surface is equal to μ . The minimum constant force that has to be applied in the horizontal direction to the block of mass M1 in order to shift the other block is (M1+M2α)μg , then α is :
View SolutionTwo bars of masses m_(1) and m_(2) , connected by light underformed horizontal spring are lying on a uniformly rough horizontal surface, having coefficient of friction mu . The minimum constant force that has to be applied horizontal to the bar m_(1) along the length of the spring, in order to just shift the other bar m_(2) is
View Solution
