दिए गए इलेक्ट्रॉन संक्रमणों के लिए λ1,λ2 एवं λ3 में सम्बन्ध प्राप्त कीजिए।
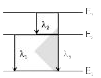
दी हुई स्थिति के लिए E3−E1=(E3−E2)+(E2−E1)
⇒hcλ3=hcλ2+hcλ1⇒1λ3=1λ2+1λ1 इसलिए λ3=λ2λ1λ2+λ1
Topper's Solved these Questions
- परमाणु संरचना तथा X -किरणे BOOK - ALLEN HINDICHAPTER - परमाणु संरचना तथा X -किरणे EXERCISE - BEGINNER.S BOX -1
6 Videos
- परमाणु संरचना तथा X -किरणे BOOK - ALLEN HINDICHAPTER - परमाणु संरचना तथा X -किरणे EXERCISE - BEGINNER.S BOX -2
7 Videos
- पदार्थो के गुण एवं तरल यांत्रिकी BOOK - ALLEN HINDICHAPTER - पदार्थो के गुण एवं तरल यांत्रिकी EXERCISE - EXERCISE-IV (Assertion & Reason)
34 Videos
- प्रकाश विद्युत प्रभाव तथा द्रव्य तरंगे BOOK - ALLEN HINDICHAPTER - प्रकाश विद्युत प्रभाव तथा द्रव्य तरंगे EXERCISE - EXERCISE - IV (Assertion & Reason)
16 Videos
Similar Questions
जब हाइड्रोजन परमाणु को मूल अवस्था में 975Å तरंगदैर्ध्य के विकिरण द्वारा उत्तेजित किया जाता है ।अधिकतम एवं न्यूनतम तरंगदैर्ध्य की गणना कीजिये
View Solutionयदि Mo (Z = 42) से प्राप्त Kα विकिरण की तरंगदैर्ध्य 0.71Å है Cu (Z = 29) के संगत विकिरण की तरंगदैर्ध्य की गणना कीजिये।
View Solutionकथन :- प्रत्यास्थ प्रत्यानयन बल संरक्षी बल हो सकते हैं।
कारण :- भार बढ़ाते हुए व घटाते हुए समान प्रतिबल पर उत्पन्न विकृति के मान क्रमशः भिन्न-भिन्न होते हैं
View Solutionकथन : प्रकाश विद्युत प्रभाव प्रकाश के तरंग स्वरूप को दर्शाता है|
कारण : प्रकाश - इलेक्ट्रोनो की संख्या प्रकाश-आवृत्ति के समानुपाती होती है|
View SolutionIf a1=a,a2=b,an+2=an+1+an2,n≥0 then limn→∞an is a+λ1bλ2,(λ1,λ2εI) where λ1+λ2 is _______
View SolutionDue to transitions among its first three energy levels, hydrogenic atom emits radiation at three discrete wavelengths λ1,λ2, and λ3(λ1<λ2<λ3). Then
View SolutionIf λ1,λ2andλ3 are the wavelengths of the wave giving resonance with the fundamental, first and second overtones respectively of a closed organ pipe Then the ratio of wavelength λ1,λ2andλ3 is
View SolutionThe matrix A=⎡⎢ ⎢⎣λ21λ1λ2λ1λ3λ2λ1λ22λ2λ3λ3λ1λ3λ2λ23⎤⎥ ⎥⎦ is idempotent if λ21+λ22+λ23=k where λ1,λ2,λ3 are non-zero real numbers. Then the value of (10+k)2 is . . .
View SolutionIf λ1andλ2 are the wavelengths of the first members of the Lyman and Paschen series respectively, then λ1:λ2 is
View SolutionFor Balmer series, wavelength of first line is lamda_(1) and for Brackett series, wavelength of first line is lamda_(2) then (lamda_(1))/(lamda_(2))
View SolutionIf |ai|<1λi≥0 for i=1,2,3,.......nandλ1+λ2+.......+λn=1 then the value of |λ1a1+λ2a2+.......+λnan| is :
View SolutionIf λ1 and λ2 are the two valyes of λ such that the α and β of quadratic equation λ(x2−x)+x−5=0 satisfy αβ+βα+45=0 then λ1λ22+λ2λ21 is equal to
View SolutionIf |a_(k)|lt1,lamda_(k)ge0 , Where k=1,2, . . ,n And lamda_(1)+lamda_(2)+ . . +lamda_n=1 , Then |lamda_(1)a_(1)+lamda_(2)a_(2)+ . . .+lamda_(n)a_(n)| The value of
View SolutionIf lambda_(1), lamda_(2)and lamda_(3) are the wavelengths of the wave giving resonance with the fundamental, first and second overtones respectively of a closed organ pipe Then the ratio of wavelength lambda_(1), lamda_(2)and lamda_(3) is
View SolutionIf α,β are the roots of the equation λ(x2−x)+x+5=0 and if λ1andλ2 are two values of λ obtained from αβ+βα=4, then λ1λ22+λ2λ21 equals.
View Solution
