If we multiply or divide both sides of a linear equation with a non-zero number, then the solution of the linear equation.
changes
remains the same
Only changes in case of multiplication
Only changes in case of division
To solve the question, we will demonstrate the property of linear equations that states if we multiply or divide both sides of a linear equation by a non-zero number, the solution remains unchanged.
1. Start with a Linear Equation:
Let's consider the linear equation:
x+y=7
Here, we have a solution for this equation, which is (5,2) meaning x=5 and y=2.
2. Verify the Solution:
Substitute x and y into the equation:
5+2=7
This is true, confirming that (5,2) is indeed a solution.
3. Multiply Both Sides by a Non-Zero Number:
Now, let's multiply the entire equation by 2 (a non-zero number):
2(x+y)=2⋅7
Simplifying this gives:
2x+2y=14
4. Substitute the Solution into the New Equation:
Substitute x=5 and y=2 into the new equation:
2(5)+2(2)=14
Calculating this gives:
10+4=14
This is also true, confirming that the solution remains valid.
5. Conclusion:
Since we have shown that multiplying both sides of the equation by a non-zero number does not change the solution, we conclude that the solution of the linear equation remains the same.
Final Answer:
The solution of the linear equation remains unchanged when we multiply or divide both sides by a non-zero number.
---
Let's consider the linear equation:
x+y=7
Here, we have a solution for this equation, which is (5,2) meaning x=5 and y=2.
Substitute x and y into the equation:
5+2=7
This is true, confirming that (5,2) is indeed a solution.
Now, let's multiply the entire equation by 2 (a non-zero number):
2(x+y)=2⋅7
Simplifying this gives:
2x+2y=14
Substitute x=5 and y=2 into the new equation:
2(5)+2(2)=14
Calculating this gives:
10+4=14
This is also true, confirming that the solution remains valid.
Since we have shown that multiplying both sides of the equation by a non-zero number does not change the solution, we conclude that the solution of the linear equation remains the same.
The solution of the linear equation remains unchanged when we multiply or divide both sides by a non-zero number.
Topper's Solved these Questions
- LINEAR EQUATION IN TWO VARIABLESBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - LINEAR EQUATION IN TWO VARIABLESEXERCISE - Exercise 4.2
1 Videos
- LINEAR EQUATION IN TWO VARIABLESBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - LINEAR EQUATION IN TWO VARIABLESEXERCISE - Exercise 4.3
11 Videos
- INTRODUCTION TO EUCLID GEOMETRYBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - INTRODUCTION TO EUCLID GEOMETRYEXERCISE - Exercise 5.4 Long Answer Type Questions
5 Videos
- LINES AND ANGLES BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - LINES AND ANGLES EXERCISE - MULTIPLE CHOICE QUESTIONS
34 Videos
Similar Questions
Knowledge Check
If
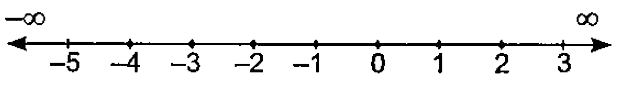 is the solution set of a linear in equation in x, then the solution set of x in set-builder form is:A{x:−4≤x≤2,x∈R}B{x:−5<x<3,x∈R}C{x:−4≤x≤2,x∈N}D{x:−5<x<3,x∈I}
is the solution set of a linear in equation in x, then the solution set of x in set-builder form is:A{x:−4≤x≤2,x∈R}B{x:−5<x<3,x∈R}C{x:−4≤x≤2,x∈N}D{x:−5<x<3,x∈I}On multiplying {−5} on both sides of a linear inequation, the linear inequation become ......
A−2x+7≥−4x+9B2x−7≥4x−9C−2x+7≤4x−9D2x−7≤4x−9
Simultaneously linear equation
View SolutionLinear equation in two variable
View SolutionForm a linear equation and a word problem with solution 12.
View SolutionFormulate a linear equation and a word problem, each with solution 18.
View SolutionShow that the wave funcation y=eb(x−vt) is a solution of the linear wave equation.
View SolutionFind the number of non-zero integral solutions of the equation |1−i|x=2x.
View Solution
