For all sets A, B and C,A−(B−C)=(A−B)−C.
Video Solution
Text Solution
See the Venn diagrams given below. Where shaded portions are representing A−(B−C) and (A−B)−C respectively.
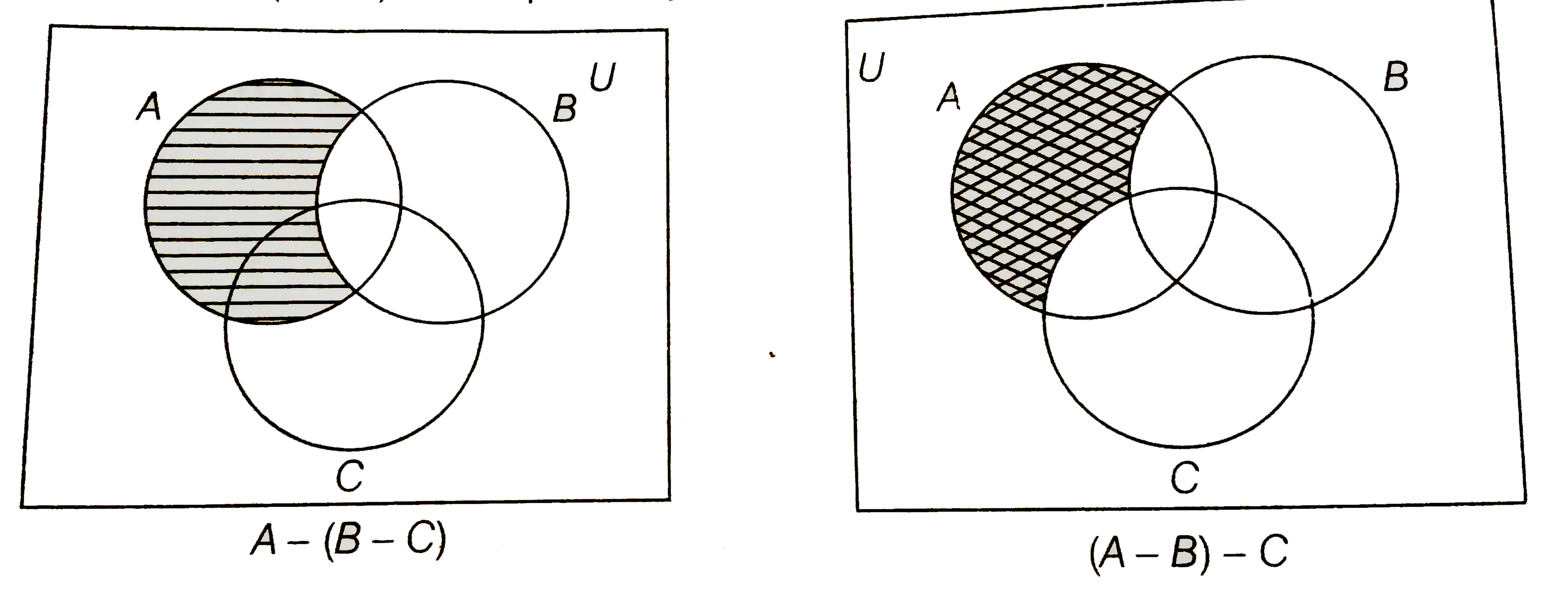
Clearly A−(B−C)≠(A−B)−C.
Hence, given statement is false.
|
Topper's Solved these Questions
- SETSBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - SETSEXERCISE - LONG ANSWERS TYPE QUESTIONS
6 Videos
- SETSBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - SETSEXERCISE - OBJECTIVE TYPE QUESTIONS
14 Videos
- SEQUENCE AND SERIESBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - SEQUENCE AND SERIESEXERCISE - Match the comumms
2 Videos
- STATISTICSBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - STATISTICSEXERCISE - FILLERS
7 Videos
Similar Questions
For all sets A,B and C, if A⊂B, then A∪C⊂B∪C.
View SolutionFor all sets A,B and C, if A⊂B, then A∩C⊂B∩C.
View SolutionStatement-1 If U is universal set and B = U - A, then n(B) = n(U) - n(A).
Statement-2 For any three arbitrary sets A, B and C, if C = A - B, then n(C ) = n(A) - n(B).
View SolutionFor any three sets A, B, and C A∩(B−C)=(A∩B)−(A∩C)
View SolutionFor all sets A, B and C, if A⊂C and B⊂C, then A∪B⊂C.
View SolutionFor all sets A,B and C show that (A−B)∩(A−C)=A−(B∪C)
View SolutionFor three sets A,B,C,A−(B∪C)=?
View SolutionFor sets A, B,C , show that (A−B)∪(A−C)=A−(B∪C)
View SolutionIf A, B and C be sets. Then, show that A∩(B∪C)=(A∩B)∪(A∩C).
View SolutionFor sets A,B, and C using Venn diagram , check if A-(B-C) = (A-B-C)
View Solution
