Question

Let $S=$
set of point inside the sqare, $T=$
set of points inside the triangles and $C=$
the set of point inside the circle, if the triangle and circle intersect
each other are contained in the square, then (a) $S\cap T\cap C=\varphi$(b)$S\cup T\cup C=C$(c) $S\cup T\cup C=S$$\left(d\right)S\cup T=S\cap C$

Options

A. `S nn T nn C = phi`
B. `S uu T uu C = C`
C. `S uu T uu C = S`
D. `S uu T = S nn C`

Answer

C

Explanation

The given sets can be represented in Venn diagram as shown below

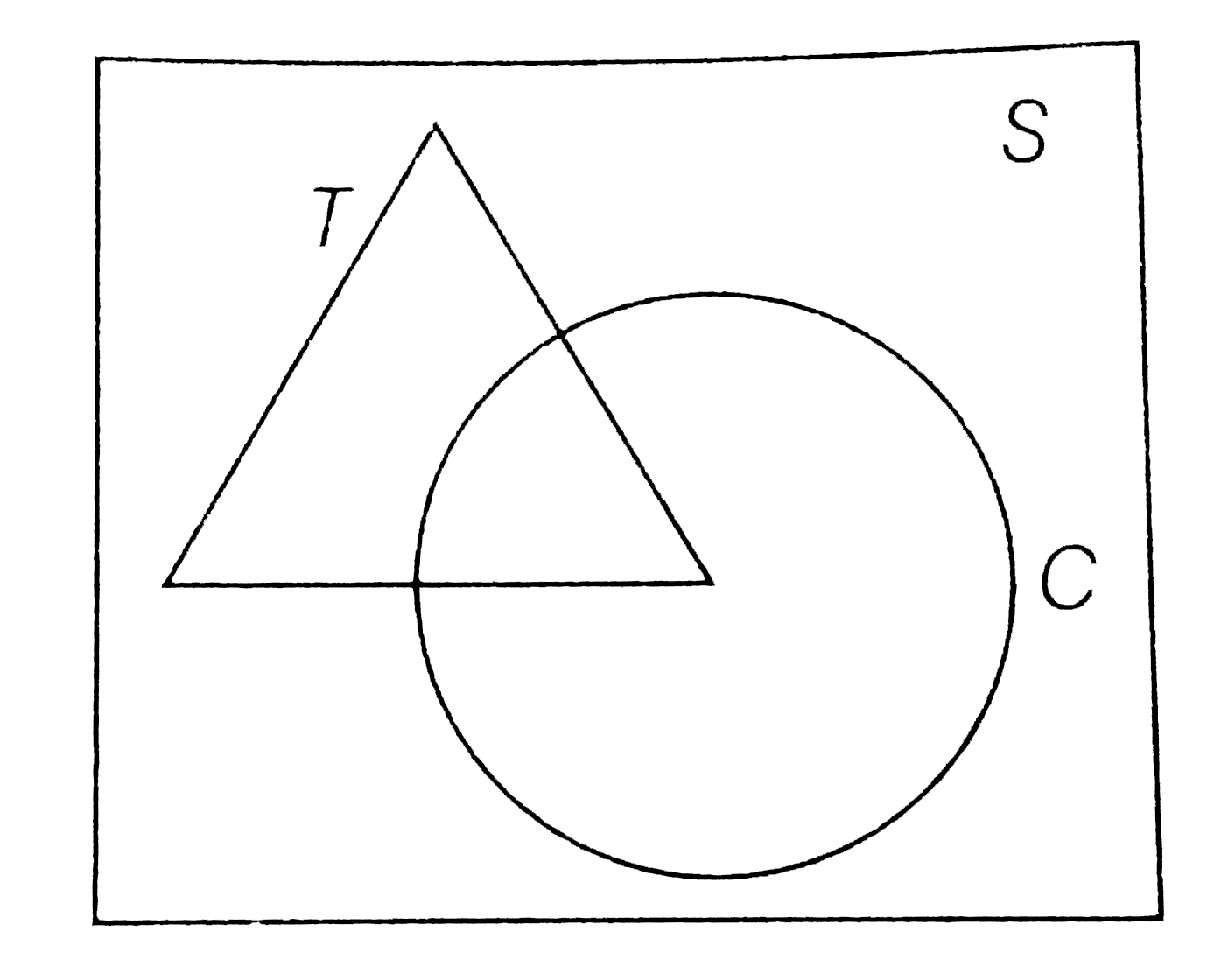
It is clear from the diagram that, `S uu T uu C = S`.