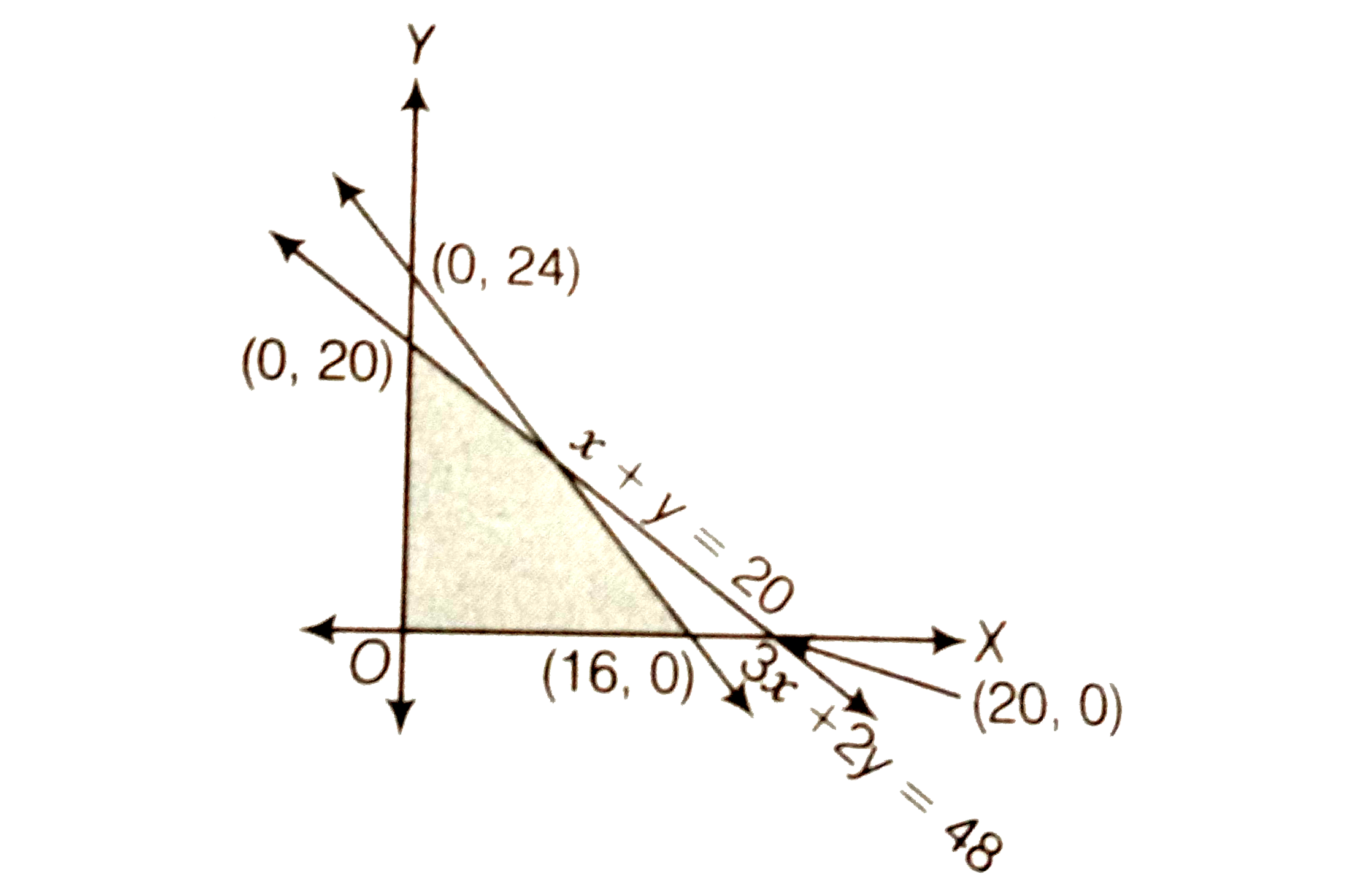
Find the linear inequalities for which the shaded region in the given figure is the solution set.
Video Solution
Text Solution
Consider the line 3x+2y=48, we observe that the shaded and the origin are on the same side of the line 3x+2y=48and(0,0) satisfy the linear constraint 3x+2y≤48. So, we must have one inequation as 3x+2y≤48.
Now, condider the line x+ y = 20 . We find that the shaded region and the origin are on the same side of the line x + y = 20 and (0, 0) satisfy the constraints x+y≤20. So, the second inequation is x+y≤20.
We also notice that the shaded region is above X- axis and is on the right side of y-axis, so we must have x≥0,y≥0.
Thus, the linear inequations corresponding to the given solution set are 3x+2y≤48,x+y≤20andx≥0,y≥0.
|
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - LINEAR INEQUALITIESEXERCISE - Objective Type Questions14videos
LINEAR INEQUALITIES
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - LINEAR INEQUALITIESEXERCISE - Objective Type Questions14videos
LINEAR INEQUALITIES
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - LIMITS AND DERIVATIVES EXERCISE - FILLERS4videos
LIMITS AND DERIVATIVES
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - MATHEMATICAL REASONING EXERCISE - OBJECTIVE TYPE QUESTIONS21videos
MATHEMATICAL REASONING
Similar Questions
Knowledge Check
- Question 1 - Select One
What is the area of shaded region in the figure?
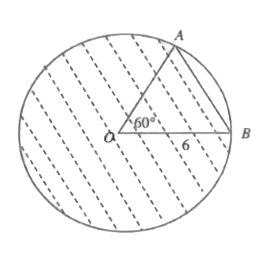 A10π+27√3B10π+274√3C30π+27√3D30π+9√3
A10π+27√3B10π+274√3C30π+27√3D30π+9√3
Find the linear inequations for which the shaded area in fig. 15.41 is the solution set. Draw the diagram of the solution set of the linear inequations.
View SolutionFind the linear equations for which the shaded area in Fig: 15.39 is the solution set.
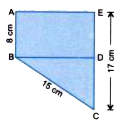
View SolutionFind the area of the shaded region in the adjoing figure :
 View Solution
View SolutionFind the area of the shaded region in the adjoing figure :
 View Solution
View SolutionFind the area of the shaded region in the adjoing figure :
 View Solution
View SolutionFind the area of the shaded region in the adjoing figure :
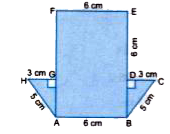 View Solution
View SolutionFind the area of the shaded region in the adjoing figure :
 View Solution
View Solution

