Using the digits 1, 2, 3, 4, 5, 6,7, a number of 4 different digits is formed. Find

Text Solution

Verified by Experts

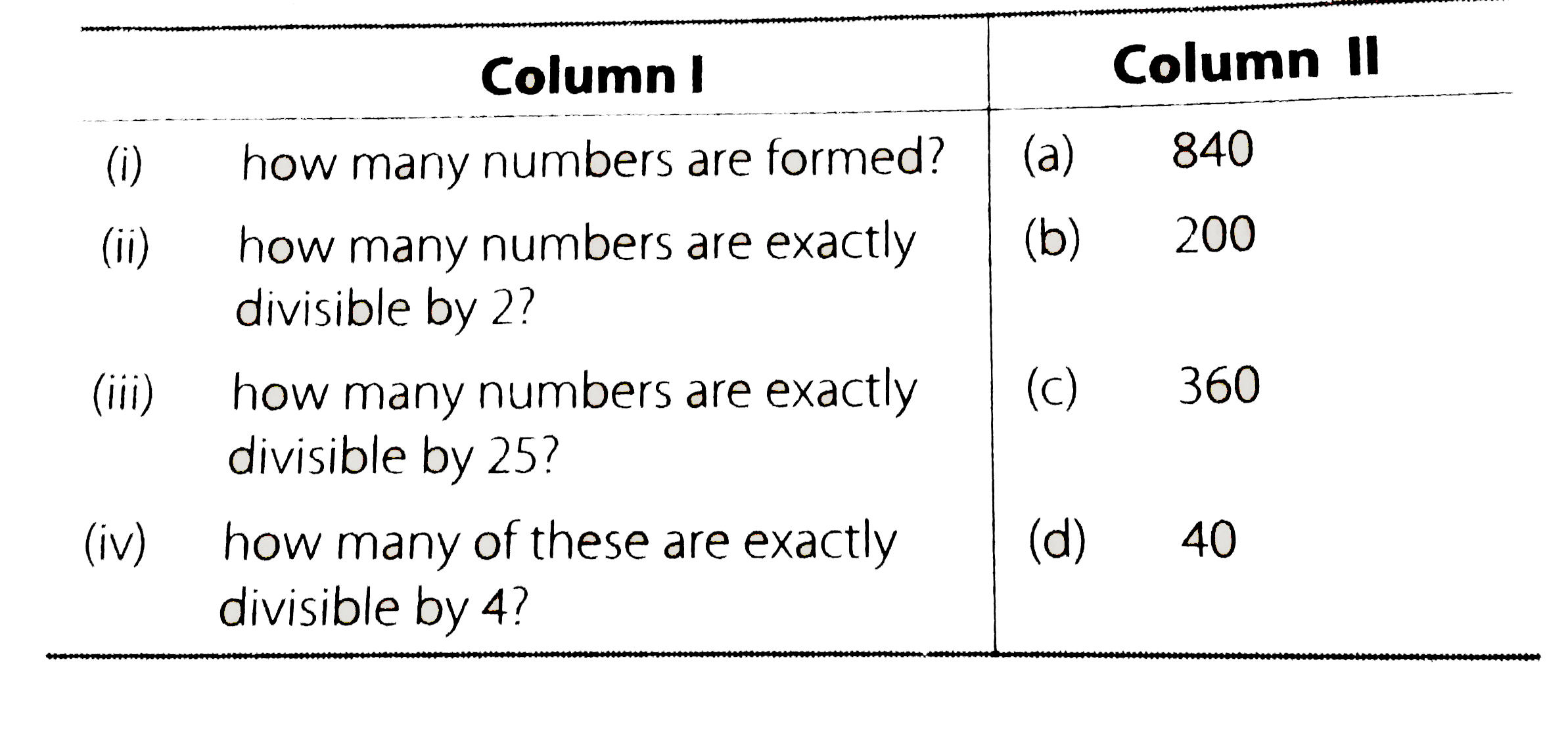
(i) Total number of 4 digit formed with digit 1, 2, 3, 4, 5, 6, 7
$= 7 \times 6 \times 5 \times 4 = 840$
(ii) When a number is divisible by 2. At its unit place only even numbers occurs.
Total numbers $= 4 \times 5 \times 6 \times 3 = 360$
(iii) Total numbers which are divisible by 25 = 40
(iv) A number is divisible 4, If its last two digit is divisible by 4.
$∴$ Total such numbers = 200

Updated on:21/07/2023

A four digit number of distinct digits is formed by using the digits 2, 3, 4, 5, 6, 7, 8. The number of such numbers which are divisible by 25, is
A60
B40
C20
D15
The number of four-digit numbers that can be formed by using the digits $1, 2, 3, 4, 5, 6, 7, 8$ and $9$ such that the least digit used is $4$ , when repetition of digits is allowed is
A $617$
B $671$
C $716$
D $761$
Find the number of 6-digit numbers that can be found using the digits 1, 2, 3, 4, 5, 6 once such that the 6-digit number is divisible by its unit digit. (The unit digit is not 1).
AA) 620
BB) 456
CC) 520
DD) 528