A cone of radius 8 cm and height 12 cm is divided into two parts by a plane through the mid-point of its axis parallel to its base Find the ratio of the volumes of two parts
Let ORN be the cone then , given radius of the base of the bases of the coner .
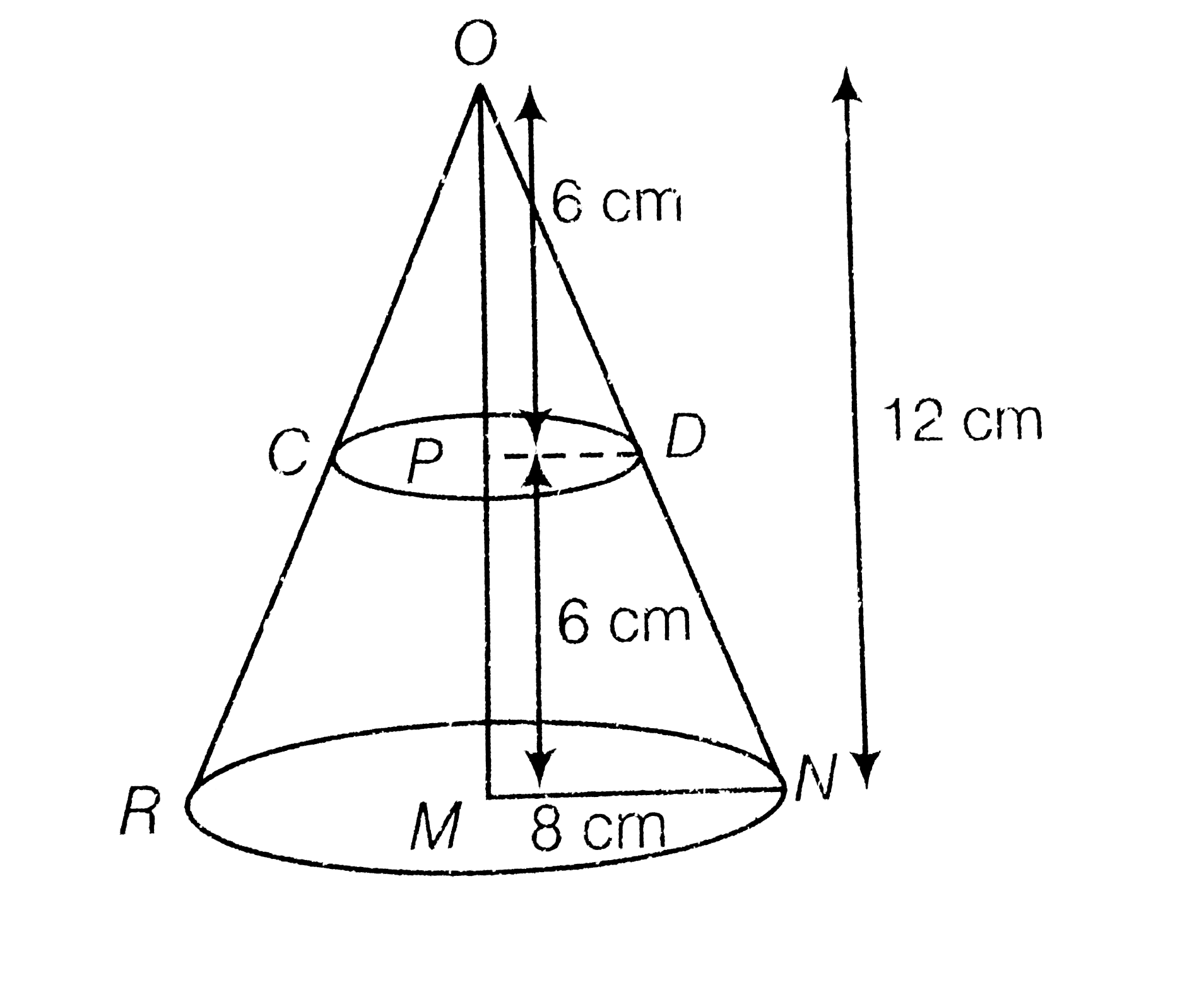
and height of the cone, (h) OM = 12 cm
Let P be the mid-point of OM, then
Now,
The plane along CD divides the cone into two parts , namely
(i) a smalle cone of radius 4 cm and height 6 cm and (ii) frustum of a cone for which
Radius of the top the frustum ,
Radius of the bottom,
and height of the frustum, h = 6 cm
Volume of smaller cone
and volume of the frustum of cone
Required ratio = volume of furstum : Volume of cone
Topper's Solved these Questions
- SURFACE AREAS AND VOLUMESBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - SURFACE AREAS AND VOLUMESEXERCISE - LONG ANSWER TYPE QUESTIONS
20 Videos
- SURFACE AREAS AND VOLUMESBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - SURFACE AREAS AND VOLUMESEXERCISE - VERY SHORT ANSWER TYPE QUESTIONS
8 Videos
- STATISTICS AND PROBABILITYBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - STATISTICS AND PROBABILITYEXERCISE - LONG ASWERS QUESTIONS
14 Videos
- TRIANGLESBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - TRIANGLESEXERCISE - Long Answer Type Questions
18 Videos
Similar Questions
A cone of radius 4 cm is divided into two parts by drawing a plane through the mid-point of its axis and parallel to its base. Compare the volumes of the two parts.
View SolutionIf a cone of radius 10cm is divided into two parts by drawing a plane through the mid-point of its axis, parallel to its base. Compare the volumes of the two parts.
View SolutionA solid cone of base radius 10 cm is cut into two parts through the mid-point of its height, by a plane parallel to its base. Find the ratio in the volumes of two parts of the cone.
View SolutionIf a cone is cut into two parts by a horizontal plane passing through the mid-point of its axis, the ratio of the volumes of upper and lower part is (a) 1:2 (b) 2:1 (c) 1:7 (d) 1:8
View SolutionA solid right circular cone is cut into two parts at the middle of its height by a plane parallel to its base. The ratio of the volume of the smaller cone to the whole cone is:
View SolutionA solid right circular cone is cut into two parts at the middle of its height by a plane parallel to its base. The ratio of the volume of the smaller cone to the whole cone is: 1:2 (b) 1:4 (c) 1:6 (d) 1:8
View SolutionA solid right circular cone is cut into two parts at the middle of its height by a plane parallel to its base. The ratio of the volume of the smaller cone to the whole cone is: 1:2 (b) 1:4 (c) 1:6 (d) 1:8
View SolutionIf a cone is cut into two parts by a horizontal plane passing through the mid-point of its axis, the ratio of the volumes f upper and lower part is (a) 1:2 (b) 2:1 (c) 1:7 (d) 1:8
View SolutionA metallic right circular cone 20 cm high and whose vertical angle is 60∘is cut into two parts at the middle of its height by a plane parallel to its base. If the frustum so obtained be drawn into a wire of diameter 116cm find the length of the wire.
View SolutionA solid metallic right circular cone 20 cm high with vertical angle 60o is cut into two parts at the middle point of its height by a plane parallel to the base. If the frustum, so obtained, be drawn into a wire of diameter 116 cm , find the length of the wire.
View Solution
