A rectangular water tank of base 11m×6m contains water upto a height of 5 m. If the water in the tank is transferred to a cylindrical tank of radius 3.5 m, find the height of the water level in the tank.
To solve the problem step by step, we will calculate the volume of water in the rectangular tank and then use that volume to find the height of the water in the cylindrical tank.
Step 1: Calculate the volume of water in the rectangular tank.
The volume V of a rectangular tank can be calculated using the formula:
V=length×width×height
Given:
- Length = 11 m
- Width = 6 m
- Height = 5 m
Substituting the values:
V=11m×6m×5m=330m3
Step 2: Set up the volume equation for the cylindrical tank.
The volume of a cylinder is given by the formula:
V=πr2h
Where:
- r is the radius of the cylinder
- h is the height of the water in the cylindrical tank
Given:
- Radius r=3.5m
We can express the volume of the cylindrical tank as:
V=π(3.5)2h
Step 3: Equate the volumes of the rectangular tank and the cylindrical tank.
Since the volume of water remains constant when transferred from the rectangular tank to the cylindrical tank:
330=π(3.5)2h
Step 4: Substitute the value of π.
Using π≈227:
330=227(3.5)2h
Step 5: Calculate (3.5)2.
(3.5)2=12.25
So the equation becomes:
330=227×12.25×h
Step 6: Simplify the equation.
330=22×12.257h
Calculating 22×12.257:
22×12.25=270.5
So,
330=270.57h
Step 7: Solve for h.
Multiply both sides by 7 to eliminate the fraction:
330×7=270.5h
2310=270.5h
Now, divide both sides by 270.5:
h=2310270.5≈8.57m
Step 8: Round the answer.
Rounding to one decimal place, we find:
h≈8.6m
Final Answer:
The height of the water level in the cylindrical tank is approximately 8.6 m.
The volume V of a rectangular tank can be calculated using the formula:
V=length×width×height
Given:
- Length = 11 m
- Width = 6 m
- Height = 5 m
V=11m×6m×5m=330m3
The volume of a cylinder is given by the formula:
V=πr2h
Where:
- r is the radius of the cylinder
- h is the height of the water in the cylindrical tank
- Radius r=3.5m
V=π(3.5)2h
Since the volume of water remains constant when transferred from the rectangular tank to the cylindrical tank:
330=π(3.5)2h
Using π≈227:
330=227(3.5)2h
(3.5)2=12.25
So the equation becomes:
330=227×12.25×h
330=22×12.257h
Calculating 22×12.257:
22×12.25=270.5
So,
330=270.57h
Multiply both sides by 7 to eliminate the fraction:
330×7=270.5h
2310=270.5h
Now, divide both sides by 270.5:
h=2310270.5≈8.57m
Rounding to one decimal place, we find:
h≈8.6m
The height of the water level in the cylindrical tank is approximately 8.6 m.
Topper's Solved these Questions
- SURFACE AREAS AND VOLUMESBook:NCERT EXEMPLAR ENGLISHChapter:SURFACE AREAS AND VOLUMESExercise:SHORT ANSWER TYOE QUESTIONS
Explore 14 Videos
- STATISTICS AND PROBABILITYBook:NCERT EXEMPLAR ENGLISHChapter:STATISTICS AND PROBABILITYExercise:LONG ASWERS QUESTIONS
Explore 14 Videos
- TRIANGLESBook:NCERT EXEMPLAR ENGLISHChapter:TRIANGLESExercise:Long Answer Type Questions
Explore 18 Videos
Similar Questions
A cylindrical bucket holds 44.372 litre of water. The water is emptied into a rectangular tank 66 cm long and 28 cm wide. Find the height of the water level in the tank.
View SolutionA cylindrical bucket 28 cm in diameter and 72 cm high is full of water. The water is emptied into a rectangular tank 66 cm long and 28 cm wide. Find the height of the water level in the tank.
View Solution
Knowledge Check
A closed rectangular tank contains a certain amount of water. When the tank is placed on its 3 ft. by 4 ft. side, the height of the water in the tank is 5 ft. when the tank is placed on another side of dimension 4 ft. by 5 ft., what is the height, in feet, of the surface of the water above the ground?
A2B3C4D5
A cylinderical bucket 28 cm in diameter and 72 cm high is full of water . The water is emptied in to a rectangualr tank 66 cm long and 28 cm wide .Find the height of water level in the tank.
View SolutionA conical flask is full of water. The flask has base-radius r and height h . The water is poured into a cylindrical flask of base-radius mr . Find the height of water in the cylindrical flask.
View SolutionA rectangular tank 15 m long and 11 m broad is required to receive entire liquid contents from a full cylindrical tank of internal diameter 21 m and length 5 m. Find the least height of the tank that will serve the purpose.
View SolutionA rectangular water reservoir contains 105 m3 of water. Find the depth of the water in the reservoir if its base measures 12m by 3.5m.
View SolutionA rectangular water reservoir contains 42000 litres of water. Find the depth of the water in the reservoir if its base measures 6m by 3.5m.
View SolutionA rectangular tank of base area 100 m2 has a height of 2 m. Calculate the thrust at the bottom of the tank, if it is filled upto the brim with water of density 103 kg m−3.
View SolutionAn open rectangular tank 5m×4m×3m high containing water upto a height of 2m is accelerated horizontally along the longer side .
(a) Determine the maximum acceleration that can be given without spilling the water .
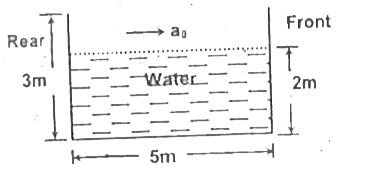
View Solution