Question

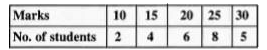
The mean deviation of the data is ….. When measured from the meadian

Answer

N/a

Explanation

To solve the question regarding the mean deviation of the data when measured from the median, we can follow these steps:

Step 1: Understand Mean Deviation
Mean deviation is a measure of dispersion that indicates how much the values in a dataset deviate from a central point (mean, median, or mode). It is calculated as the average of the absolute deviations from the central point.

Step 2: Identify the Central Point
In this case, we are interested in the mean deviation when measured from the median. The median is the middle value of a dataset when it is ordered.

Step 3: Calculate Absolute Deviations
To find the mean deviation from the median, we need to calculate the absolute deviations of each data point from the median. This means for each data point \( x_i \), we compute \( |x_i - \text{median}| \).

Step 4: Sum the Absolute Deviations
Next, we sum all the absolute deviations calculated in the previous step:
\( \text{Sum of Absolute Deviations} = \sum |x_i - \text{median}| \)

Step 5: Calculate Mean Deviation
Finally, to find the mean deviation, we divide the sum of the absolute deviations by the number of data points \( n \):
\( \text{Mean Deviation} = \frac{\sum |x_i - \text{median}|}{n} \)

Conclusion
The mean deviation of the data is least when measured from the median compared to when it is measured from the mean or mode. This is a statistical property that indicates the median provides a better central tendency measure for minimizing deviations in a dataset.