A discrete random variable X has the probability distribution as given below
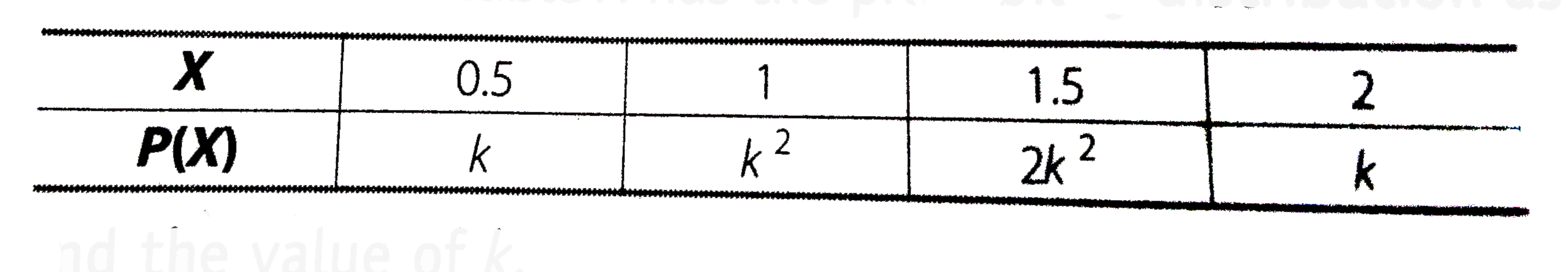
(i) Find the value of k.
(ii) Determine the mean of the distribution.
We have,

(i) We know that, n∑i=1Pi=1, where Pi≥0
⇒P1+P2+P3+P4=1
⇒k+k2+2k2+k=1
⇒3k2+2k−1=0
⇒3k2+3k−k−1=0
⇒(3k−1)(k+1)=0
⇒k=1/3⇒k=−1
Since, k is ≥0⇒k=1/3
(ii) Mean of the distribution (μ)=E(X)=n∑i=1ixiPi
=0.5(k)+1(k2+1.5(2k2)+2(k)=4k2+2.5k
=4⋅19+2.5⋅13
=4+7.59=2318
Topper's Solved these Questions
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - PROBABILITYEXERCISE - Long Answer Type Questions15videos
PROBABILITY
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - PROBABILITYEXERCISE - Objective Type Questions38videos
PROBABILITY
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - MATRICESEXERCISE - Solved example101videos
MATRICES
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - RELATIONS AND FUNCTIONSEXERCISE - Fillers15videos
RELATIONS AND FUNCTIONS
Similar Questions
A random variable X has the following distribution
 View Solution
View SolutionA discrete random variable X has the probability distribution given below: X:0.511.52 P(X):kk22k2k Find the value of k. (ii) Determine the mean of the distribution.
View SolutionA random variable X has the following probability distribution :

determine the value of a
View SolutionFind the value of x, if the mean of the following distribution is 18.
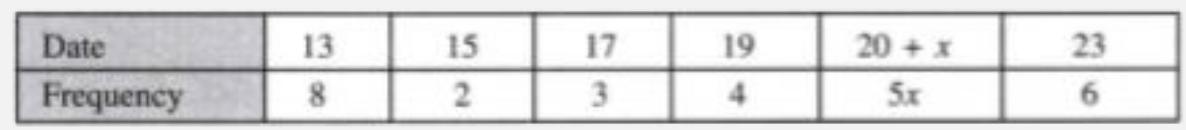 View Solution
View SolutionA random variable 'X' has the following probability distribution :

The values of k isView Solution
is the probability distribution of a random variable X. Find the variance of X.View Solution
is the probability distribution of a random variable X. Find the variance of X.View SolutionFind the probability distribution of the maximum of two scores obtained when a die is thrown twice. Determine also the mean of the distribution.
View SolutionFind the probability distribution of the maximum of two scores obtained when a die is thrown twice. Determine also the mean of the distribution.
View SolutionA random variable X has the following distribution .

The value of k and P(X<4) are equal toView Solution

