Suppose 5% of men and 0.25% of women have grey hair. A grey haired person is selected at random. What is the probability of this person being male? Assume that there are equal number of males and females.
To solve the problem, we will use the concept of conditional probability. We need to find the probability that a randomly selected grey-haired person is male.
1. Define the probabilities:
- Let P(M) be the probability of selecting a male.
- Let P(W) be the probability of selecting a female.
- Since there are equal numbers of males and females, we have:
P(M)=P(W)=12
2. Determine the probabilities of having grey hair:
- The probability that a male has grey hair, P(G|M), is given as 5%, which can be expressed as:
P(G|M)=5100=0.05
- The probability that a female has grey hair, P(G|W), is given as 0.25%, which can be expressed as:
P(G|W)=0.25100=0.0025
3. Calculate the total probability of selecting a grey-haired person, P(G):
- Using the law of total probability:
P(G)=P(G|M)⋅P(M)+P(G|W)⋅P(W)
- Substituting the values:
P(G)=(0.05⋅12)+(0.0025⋅12)
P(G)=0.025+0.00125=0.02625
4. Calculate the probability that a grey-haired person is male, P(M|G):
- We use Bayes' theorem:
P(M|G)=P(G|M)⋅P(M)P(G)
- Substituting the values:
P(M|G)=0.05⋅120.02625
P(M|G)=0.0250.02625
5. Simplify the fraction:
- To simplify 0.0250.02625:
P(M|G)=2526.25=25×10026.25×100=25002625
- Dividing both numerator and denominator by 25:
P(M|G)=100105=2021
Final Answer:
The probability that a randomly selected grey-haired person is male is:
2021
- Let P(M) be the probability of selecting a male.
- Let P(W) be the probability of selecting a female.
- Since there are equal numbers of males and females, we have:
P(M)=P(W)=12
- The probability that a male has grey hair, P(G|M), is given as 5%, which can be expressed as:
P(G|M)=5100=0.05
- The probability that a female has grey hair, P(G|W), is given as 0.25%, which can be expressed as:
P(G|W)=0.25100=0.0025
- Using the law of total probability:
P(G)=P(G|M)⋅P(M)+P(G|W)⋅P(W)
- Substituting the values:
P(G)=(0.05⋅12)+(0.0025⋅12)
P(G)=0.025+0.00125=0.02625
- We use Bayes' theorem:
P(M|G)=P(G|M)⋅P(M)P(G)
- Substituting the values:
P(M|G)=0.05⋅120.02625
P(M|G)=0.0250.02625
- To simplify 0.0250.02625:
P(M|G)=2526.25=25×10026.25×100=25002625
- Dividing both numerator and denominator by 25:
P(M|G)=100105=2021
The probability that a randomly selected grey-haired person is male is:
2021
Topper's Solved these Questions
Similar Questions
Suppose that 5% of men and 0.25% of women have grey hair. A grey haired person is selected at random. What is the probability of these person being male?
Assume that there are equal number of males and females.
View SolutionSuppose 5 men out of 100 and 25 women out of 1000 are god orators. An orator is chosen at random. Find the probability that a male person is selected. Assume that there are equal number of men and women.
View Solution
Knowledge Check

The table above shows the distribution of age and gender for 25 people who entered a contest. If the contest winner will be selected at random, what is them probability that the winner will be either a female under age 40 or a male age 40 or older?A425B1025C1125D1625
John invites 12 friends to a dinner party, half of whom are men. Exactly one man and one woman are bringing desserts. If one person from this group is selected at random, what is the probability that it is a man who is not bringing a dessert OR that it is a woman?
View SolutionA committee of two persons is selected from two men and two women. What is the probability that the committee will have i. no man? Ii. one man? iii. two men?
View SolutionIn a group there are 2 men and 3 women ,3 persons are selected at random from the group . Find the probability that 1 man and 2 women or 2 men and 1 women are selected .
View SolutionA committee of two persons is selected from two men and two women. What is the probability that the committee will have i. no man? Ii. one man? iii. two men?
View SolutionThe number of committees of 5 persons consisting of at least one female number, that can be formed from 6 males and 4 females, is
View SolutionFrom the employees of a company, 5 persons are selected to represent them in the managing committee of the company. Particulars of five persons are as follows:
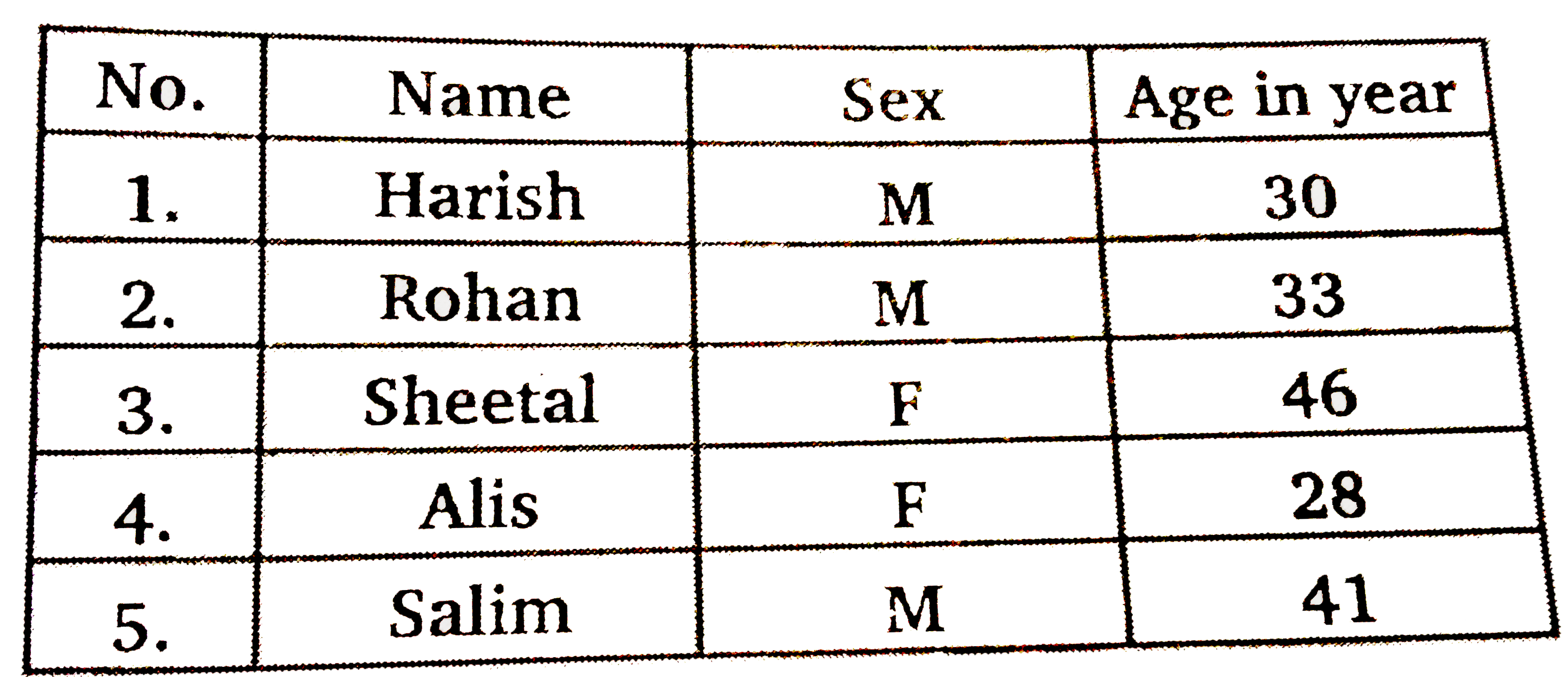
A person is selected at random from this group to act as a spokeperson. What is the probability that the spokesperson wil be either male or over 35 years?
View SolutionA committee of three persons is to be randomly selected from a group of three men and two women and the chair person will be randomly selected from 2 woman and 1 men for the committee. The probability that the committee will have exactly two woman and one man and that the chair person will be a woman, is
View Solution
