The rate of a reaction depends upon the temperature and is quantitatively expressed as
k=Ae−EaRT
i) If a graph is plotted between log k and 1/T, write the expression for the slope of the reaction?
ii) If at under different conditions Ea1andEa2 are the activation energy of two reactions. If Ea1=40J/mol andEa2=80J/ mol. Which of the two has a larger value of the rate constant?
To solve the question step by step, we will break it down into two parts as stated in the question.
Part i: Finding the slope of the graph between log k and 1/T
1. Start with the Arrhenius equation:
k=Ae−EaRT
where k is the rate constant, A is the pre-exponential factor, Ea is the activation energy, R is the universal gas constant, and T is the temperature in Kelvin.
2. Take the logarithm of both sides:
logk=logA−Ea2.303RT
Here, we use the property of logarithms that states log(ex)=x⋅log(e) and log(e)≈0.4343.
3. Rearranging the equation:
logk=logA−Ea2.303R⋅1T
This equation can be compared to the linear equation y=mx+c, where:
- y=logk
- x=1T
- m=−Ea2.303R (slope)
- c=logA (y-intercept)
4. Identify the slope:
The slope of the graph plotted between logk and 1T is:
slope=−Ea2.303R
Part ii: Comparing the rate constants for two reactions
1. Given activation energies:
- Ea1=40J/mol
- Ea2=80J/mol
2. Understanding the relationship between activation energy and rate constant:
The rate constant k is inversely proportional to the activation energy Ea. This means:
k∝1Ea
Therefore, a lower activation energy results in a higher rate constant.
3. Comparing the two activation energies:
Since Ea1<Ea2:
- k1 (for Ea1) will be greater than k2 (for Ea2):
k1>k2
Final Answers:
i) The expression for the slope of the graph between log k and 1/T is:
slope=−Ea2.303R
ii) The reaction with Ea1=40J/mol has a larger value of the rate constant compared to the reaction with Ea2=80J/mol.
k=Ae−EaRT
where k is the rate constant, A is the pre-exponential factor, Ea is the activation energy, R is the universal gas constant, and T is the temperature in Kelvin.
logk=logA−Ea2.303RT
Here, we use the property of logarithms that states log(ex)=x⋅log(e) and log(e)≈0.4343.
logk=logA−Ea2.303R⋅1T
This equation can be compared to the linear equation y=mx+c, where:
- y=logk
- x=1T
- m=−Ea2.303R (slope)
- c=logA (y-intercept)
The slope of the graph plotted between logk and 1T is:
slope=−Ea2.303R
- Ea1=40J/mol
- Ea2=80J/mol
The rate constant k is inversely proportional to the activation energy Ea. This means:
k∝1Ea
Therefore, a lower activation energy results in a higher rate constant.
Since Ea1<Ea2:
- k1 (for Ea1) will be greater than k2 (for Ea2):
k1>k2
i) The expression for the slope of the graph between log k and 1/T is:
slope=−Ea2.303R
Topper's Solved these Questions
- SAMPLE PAPER 2019BOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - SAMPLE PAPER 2019EXERCISE - SECTION: C
13 Videos
- SAMPLE PAPER 2019BOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - SAMPLE PAPER 2019EXERCISE - SECTION: D
1 Video
- SAMPLE PAPER 2019BOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - SAMPLE PAPER 2019EXERCISE - SECTION: D
1 Video
- EXAM REVISION SERIESBOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - EXAM REVISION SERIESEXERCISE - All Questions
28 Videos
- XII BOARDSBOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - XII BOARDSEXERCISE - SET - II
12 Videos
Similar Questions
The temperature dependence of the rate constant k is expressed as k=Ae−Ea/RT
When a plot between logk and 1/T is plotted we get the graph as shown.
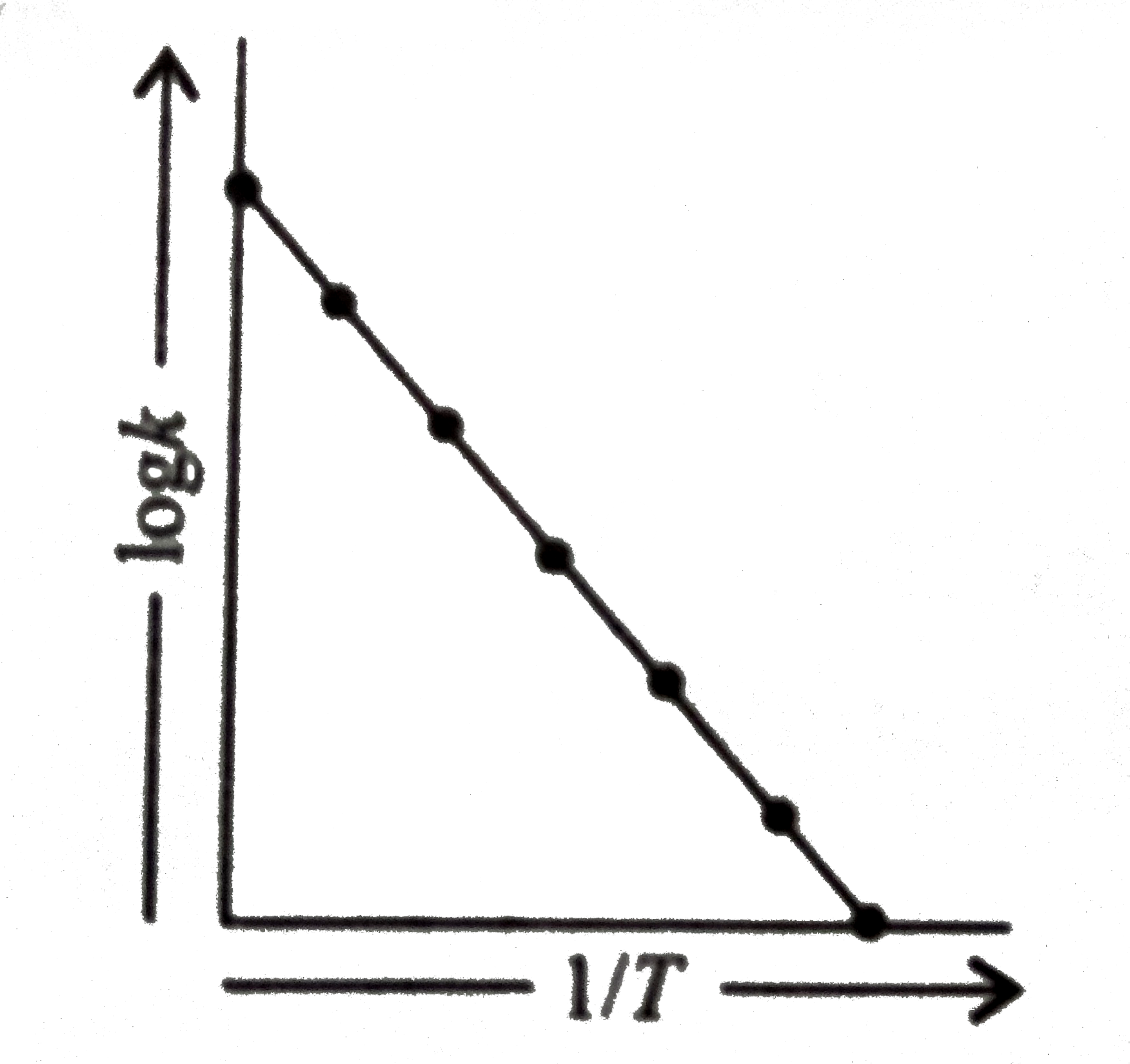
What is the value of slopw in the graph?
View SolutionThe slope of the line in the graph of log k (k = rate constant) versus 1Tis - 5841. Calculate the activation energy of the reaction.
View SolutionThe slope of the line in the graph of log k (k = rate constant) versus 1Tis - 5841. Calculate the activation energy of the reaction.
View SolutionThe slope of the line in the graph of log k (k = rate constant) versus 1Tis - 5841. Calculate the activation energy of the reaction.
View SolutionRate of a reaction can be expressed by Arrhenius equation as:
k=Ae−Ea/RT
In this equation, Ea represents:
View SolutionRate constant for a chemical reaction taking place at 500K is expressed as K=A.e−1000 The activation energy of the reaction is :
View SolutionFor a reaction, the rate constant is expressed as k=Ae−40000/T. The energy of the activation is
View SolutionArrhenius equation k=Ae−Ea/RT If the activation energy of the reaction is found to be equal to RT, then [given : 1e=0.3679]
View SolutionThe rate constant (K') of one reaction is double of the rate constant (K") of another reaction. Then the relationship between the corresponding activation energies of the two reactions (E′aandE''a) will be
View SolutionThe activation energies of two reactions are Ea1 and Ea2 with Ea1>Ea2. If the temperature of the reacting systems is increased from T to T', which of the following is correct?
View Solution