An alternating voltage given by V=140sin314t is connected across a pure resistor of 50 ohm. Find (i) the frequency of the source. (ii) the rms current thought the resistor.
To solve the problem step by step, we will find the frequency of the source and the root mean square (rms) current through the resistor.
Step 1: Identify the given voltage equation
The alternating voltage is given by:
V(t)=140sin(314t)
Step 2: Compare with the standard form
The standard form of an alternating voltage is:
V(t)=V0sin(ωt)
where V0 is the peak voltage and ω is the angular frequency.
From the given equation, we can identify:
- V0=140 volts (peak voltage)
- ω=314 radians/second (angular frequency)
Step 3: Calculate the frequency of the source
The relationship between angular frequency ω and frequency f is given by:
ω=2πf
To find the frequency f, we rearrange the equation:
f=ω2π
Substituting the value of ω:
f=3142π
Calculating f:
f≈3146.28≈50 Hz
Step 4: Calculate the rms current through the resistor
The rms current Irms through a resistor can be calculated using the formula:
Irms=VrmsR
Where Vrms is the root mean square voltage, which is related to the peak voltage V0 by:
Vrms=V0√2
Substituting the value of V0:
Vrms=140√2≈1401.41≈99.01 volts
Now, using the resistance R=50 ohms:
Irms=VrmsR=99.0150≈1.98 A
Step 5: Final results
Thus, the frequency of the source is:
f=50 Hz
And the rms current through the resistor is:
Irms≈1.98 A
Summary of Answers
(i) The frequency of the source is 50 Hz.
(ii) The rms current through the resistor is approximately 1.98 A.
---
The alternating voltage is given by:
V(t)=140sin(314t)
The standard form of an alternating voltage is:
V(t)=V0sin(ωt)
where V0 is the peak voltage and ω is the angular frequency.
- V0=140 volts (peak voltage)
- ω=314 radians/second (angular frequency)
The relationship between angular frequency ω and frequency f is given by:
ω=2πf
f=ω2π
f=3142π
f≈3146.28≈50 Hz
The rms current Irms through a resistor can be calculated using the formula:
Irms=VrmsR
Vrms=V0√2
Vrms=140√2≈1401.41≈99.01 volts
Irms=VrmsR=99.0150≈1.98 A
Thus, the frequency of the source is:
f=50 Hz
And the rms current through the resistor is:
Irms≈1.98 A
(i) The frequency of the source is 50 Hz.
(ii) The rms current through the resistor is approximately 1.98 A.
Topper's Solved these Questions
- XII BOARDSBook:XII BOARD PREVIOUS YEAR PAPER ENGLISHChapter:XII BOARDSExercise:PHYSICS (Theory) [SET - II]
Explore 6 Videos
- XII BOARDSBook:XII BOARD PREVIOUS YEAR PAPER ENGLISHChapter:XII BOARDSExercise:PHYSICS (Theory) [SET - III]
Explore 9 Videos
- XII BOARDSBook:XII BOARD PREVIOUS YEAR PAPER ENGLISHChapter:XII BOARDSExercise:[Set-III]
Explore 3 Videos
- SAMPLE PAPER 2019Book:XII BOARD PREVIOUS YEAR PAPER ENGLISHChapter:SAMPLE PAPER 2019Exercise:SECTION D
Explore 6 Videos
Similar Questions
An alternating voltage given by V=70sin100πt is connected across a pure resistor of 25Ω. Find
(i) the frequency of the source.
(ii) the rms current through the resistor.
View SolutionAn alternating voltage given by
E=280sin50πt is connected across a pure resistor of 40 ohm. Find frequency of source and rms current through the bulb.
View Solution
Knowledge Check
A alternating voltage given by V=140sin314t is connected across a pure resistor of 50 ohm. Find the rms current through the resistor.
A1.98AB5.63AC3.536AD49.39A
When a 12V battery is connected across an unknown resistor, there is a current of 2.5mA in the circuit. Find the value of the resistance of the resistor.
View SolutionIn the circuit shown in fig the voltage across the 2 Ω resistor is 12V. What is the emf of the battery and the current thorugh the 6 Ω resistor?

View SolutionIn the circuit shown in fig the voltage across the 6Ω resistor is 12V. What is the emf of the battery and the current thorugh the 6Oemga resistor?
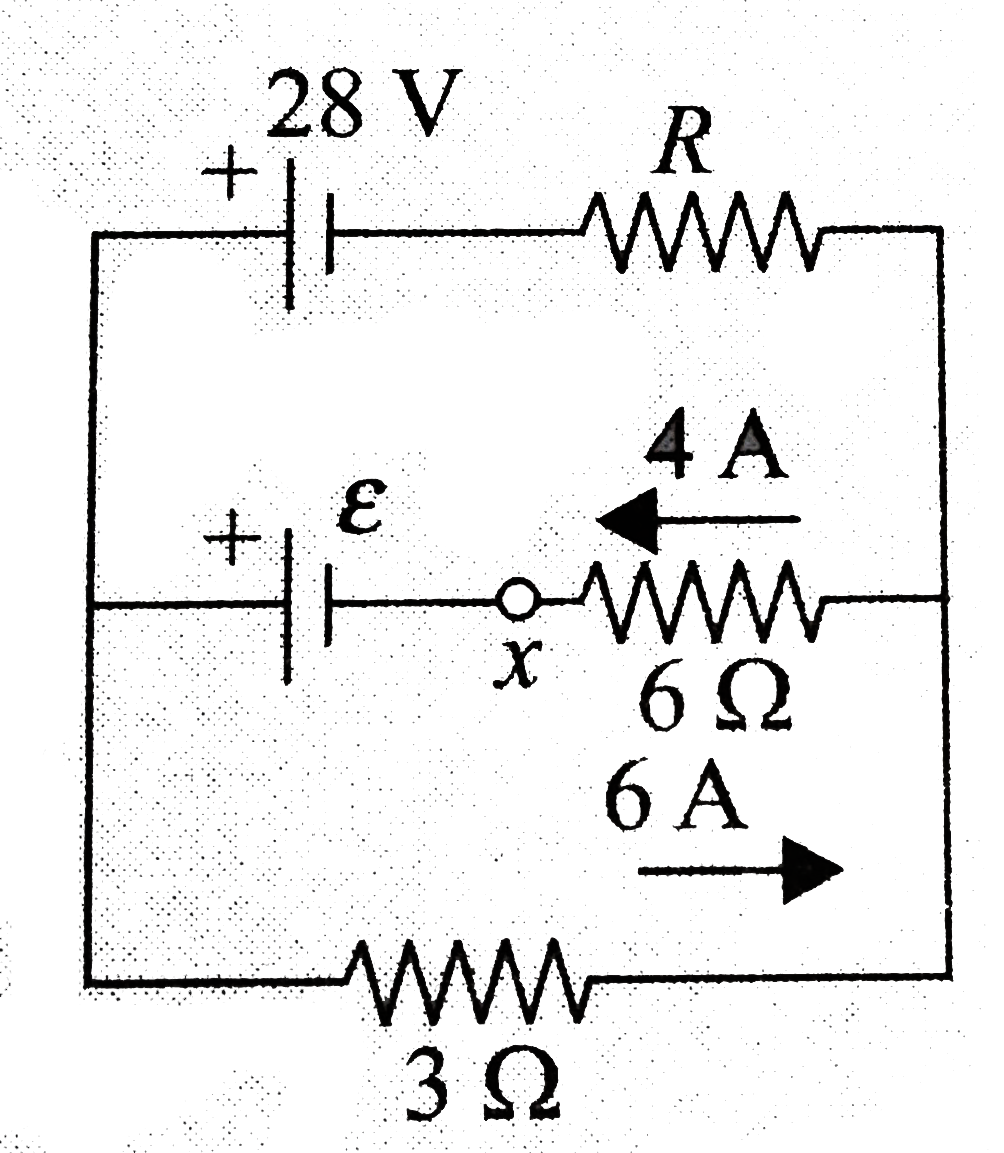
View SolutionIf an alternating voltage is represented as
E=141sin(628t), then the rms value of the voltage and the frequency are respectively
View SolutionAn emf E=100sin314tV is applied across a pure capacitor of 637(μ)F. Find
(a) the instantaneous current I
(b) the instantaneous power p.
View SolutionThree resistors are connected to a 12 V battery as shown in the figure given below :
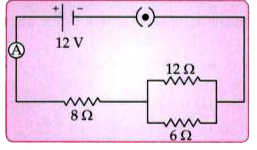
What is the current through the 8 ohm resistor ?
View SolutionThree resistors are connected to a 12 V battery as shown in the figure given below :
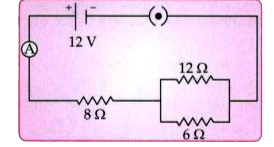
What is the current through the 6 ohm resistor ?
View Solution
