Using Huygen's construction, draw a figure showing the propagation of a plane wave reflecting at the interface of the two media. Show that the angle of incidene is equal to the angle of reflection.
Step-by-Step Solution:
1. Understanding Huygens' Principle:
Huygens' principle states that every point on a wavefront can be considered as a source of secondary wavelets that spread out in all directions with the same speed as the wave. The new wavefront at a later time is the envelope of these secondary wavelets.
Hint: Remember that Huygens' principle helps us visualize how waves propagate through space.
2. Setting Up the Problem:
Consider a plane wavefront AB incident on a reflecting surface at point B. Draw the normal line to the surface at point B. Let point C be another point on the surface.
Hint: Visualize the wavefront as a straight line approaching the surface, and mark the normal to help define angles.
3. Identifying the Incident Wavefront:
The incident wavefront AB reaches point B and starts to reflect. Draw the incident ray from point A to point B, and label the angle of incidence as ∠ABC.
Hint: The angle of incidence is measured between the incident ray and the normal at the point of incidence.
4. Secondary Wavelets:
According to Huygens' principle, as the wavefront touches point B, secondary wavelets begin to emanate from point B. These wavelets spread out in a hemisphere.
Hint: Picture the wavefront as a series of ripples emanating from the point of contact.
5. Constructing the Reflected Wavefront:
After some time, the secondary wavelets from point B will have spread out to form a new wavefront. Draw a tangent to the wavelets at point D, which represents the reflected wavefront.
Hint: The reflected wavefront will be tangent to the secondary wavelets and will be at the same distance from the normal as the incident wavefront.
6. Using Triangles to Show Equality of Angles:
In triangles ABC and DCB, we note:
- ∠BAC and ∠CDB are both right angles (90 degrees).
- The side BC is common to both triangles.
- The lengths AC and BD are equal (since they are both distances from the wavefront to the surface).
Hint: Use the properties of congruent triangles to establish relationships between angles.
7. Proving the Angles are Equal:
Since triangles ABC and DCB are congruent (by the criteria of right angle, common side, and equal sides), we conclude that:
∠ABC=∠DCB
Here, ∠ABC is the angle of incidence, and ∠DCB is the angle of reflection.
Hint: Congruent triangles imply that corresponding angles are equal, which is key to proving the law of reflection.
8. Conclusion:
We have shown that the angle of incidence is equal to the angle of reflection, thus proving the law of reflection using Huygens' construction.
Hint: Reflect on how this principle applies not only to light but also to other types of waves.
Huygens' principle states that every point on a wavefront can be considered as a source of secondary wavelets that spread out in all directions with the same speed as the wave. The new wavefront at a later time is the envelope of these secondary wavelets.
Consider a plane wavefront AB incident on a reflecting surface at point B. Draw the normal line to the surface at point B. Let point C be another point on the surface.
The incident wavefront AB reaches point B and starts to reflect. Draw the incident ray from point A to point B, and label the angle of incidence as ∠ABC.
According to Huygens' principle, as the wavefront touches point B, secondary wavelets begin to emanate from point B. These wavelets spread out in a hemisphere.
After some time, the secondary wavelets from point B will have spread out to form a new wavefront. Draw a tangent to the wavelets at point D, which represents the reflected wavefront.
In triangles ABC and DCB, we note:
- ∠BAC and ∠CDB are both right angles (90 degrees).
- The side BC is common to both triangles.
- The lengths AC and BD are equal (since they are both distances from the wavefront to the surface).
Since triangles ABC and DCB are congruent (by the criteria of right angle, common side, and equal sides), we conclude that:
∠ABC=∠DCB
Here, ∠ABC is the angle of incidence, and ∠DCB is the angle of reflection.
We have shown that the angle of incidence is equal to the angle of reflection, thus proving the law of reflection using Huygens' construction.
Similar Questions
Using Huygen's construction, draw a figure showing the propagation of a plane wave reflecting at the interface of the two media. Show that the angle of incidence is equal to the angle of reflection.
View SolutionHow is a wavefront defined? Using Huygen's construction , draw a figure showing the propagation of a plane wave refracting at a plane surface separating two media. Hence verify Snell's law of refraction.
View Solution
Knowledge Check
The angle of incidence on a plane mirror is 30∘. The angle of reflection will be:
A30∘B60∘C15∘D0∘
When a light ray is reflected from a wall, the angle of incidence is not equal to the angle of reflection.
View SolutionIn the given figure,the angle of reflection is

View SolutionDraw a diagram showing the reflection of a light ray from a plane mirror. Label on it the incident ray, the reflected ray, the normal, the angle of incidence i and the angle of reflection r.
View SolutionWhen light wave suffers reflection at the interface from air to glass, the change in phase of the reflected wave is equal to
View SolutionDraw a diagram to show the reflection of a ray of light by a plane mirror. In the diagram, label the incident ray, the reflected ray, the normal, the angle of incidence and the angle of reflection.
View Solution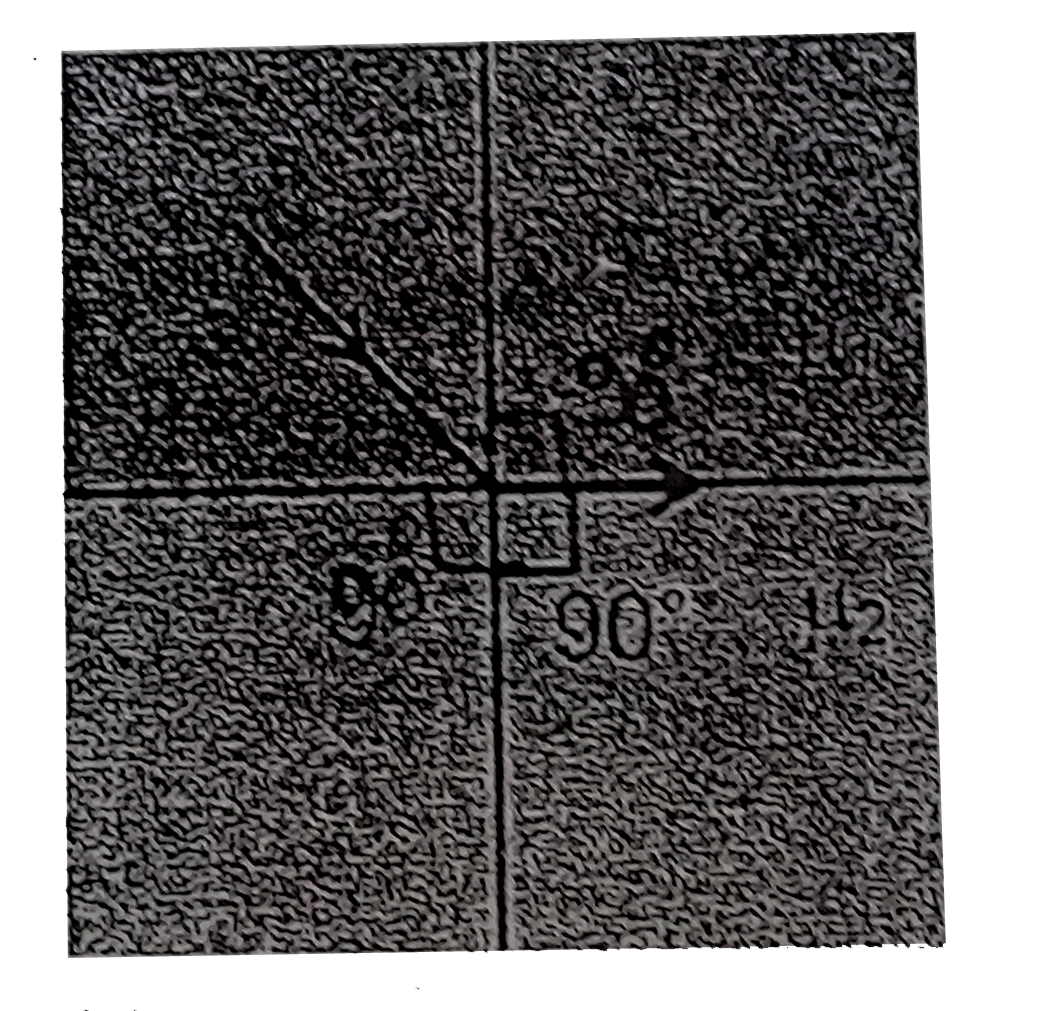
A ray is incident on interface of two media at critical angle as shown in the figure.
View SolutionIn the following figures, draw the normal at the point of incidence and then find out its angle of incidence and angle of reflection
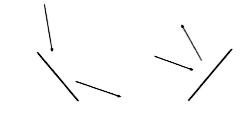
View Solution