Two Polaroids, P1andP2 are set-up so that their pass-axis are crossed with respect to each other. A third Polaroids, P3 is now introduced between these two so that its pass-axis makes and angle θ with the pass-axis of P1.
A beam of unpolarised light, of intensity I, is incident on P1. If the intensity of light, that gets transmitted through this combination of three Polaroids is I' find the ratio (I'I) when θ equals.
(i)30∘ (ii)45∘
To solve the problem step by step, we will analyze the transmission of light through the three polaroids using Malus's Law.
1. Understanding the Setup:
- We have three polaroids: P1, P2, and P3.
- The pass-axis of P1 and P2 are crossed (90 degrees apart).
- The pass-axis of P3 makes an angle θ with the pass-axis of P1.
2. Incident Light:
- A beam of unpolarized light with intensity I is incident on P1.
3. Intensity after P1:
- When unpolarized light passes through the first polaroid P1, the intensity is reduced to half:
I1=I2
4. Intensity after P3:
- The intensity after passing through P3 (which is at an angle θ to P1) is given by Malus's Law:
I2=I1⋅cos2(θ)=I2⋅cos2(θ)
5. Intensity after P2:
- The angle between P3 and P2 is 90∘−θ. Thus, the intensity after passing through P2 is:
I′=I2⋅cos2(90∘−θ)=I2⋅sin2(θ)
- Substituting I2:
I′=(I2⋅cos2(θ))⋅sin2(θ)=I2⋅cos2(θ)⋅sin2(θ)
6. Final Expression for Ratio:
- The ratio of transmitted intensity I′ to the incident intensity I is:
I′I=12⋅cos2(θ)⋅sin2(θ)
7. Calculating for Specific Angles:
- For θ=30∘:
I′I=12⋅cos2(30∘)⋅sin2(30∘=12⋅(√32)2⋅(12)2
=12⋅34⋅14=332
- For θ=45∘:
I′I=12⋅cos2(45∘)⋅sin2(45∘)=12⋅(1√2)2⋅(1√2)2
=12⋅12⋅12=18
Final Answers:
- For θ=30∘: I′I=332
- For θ=45∘: I′I=18
- We have three polaroids: P1, P2, and P3.
- The pass-axis of P1 and P2 are crossed (90 degrees apart).
- The pass-axis of P3 makes an angle θ with the pass-axis of P1.
- A beam of unpolarized light with intensity I is incident on P1.
- When unpolarized light passes through the first polaroid P1, the intensity is reduced to half:
I1=I2
- The intensity after passing through P3 (which is at an angle θ to P1) is given by Malus's Law:
I2=I1⋅cos2(θ)=I2⋅cos2(θ)
- The angle between P3 and P2 is 90∘−θ. Thus, the intensity after passing through P2 is:
I′=I2⋅cos2(90∘−θ)=I2⋅sin2(θ)
- Substituting I2:
I′=(I2⋅cos2(θ))⋅sin2(θ)=I2⋅cos2(θ)⋅sin2(θ)
- The ratio of transmitted intensity I′ to the incident intensity I is:
I′I=12⋅cos2(θ)⋅sin2(θ)
- For θ=30∘:
I′I=12⋅cos2(30∘)⋅sin2(30∘=12⋅(√32)2⋅(12)2
=12⋅34⋅14=332
I′I=12⋅cos2(45∘)⋅sin2(45∘)=12⋅(1√2)2⋅(1√2)2
=12⋅12⋅12=18
- For θ=30∘: I′I=332
- For θ=45∘: I′I=18
Topper's Solved these Questions
- XII BOARDSBOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - XII BOARDSEXERCISE - SECTIONS-E
4 Videos
- XII BOARDSBOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - XII BOARDSEXERCISE - SECTIONS-D
4 Videos
- XII BOARDSBOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - XII BOARDSEXERCISE - SECTIONS-B
4 Videos
- SAMPLE PAPER 2019BOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - SAMPLE PAPER 2019EXERCISE - SECTION D
6 Videos
Similar Questions
Two polaroids are placed such that their planes are parallel to each other with their access of transmission at 30°. If an unpolarised light of intensity I0 is incident on first polaroid polarizer then intensity of light that will be transmitted through the second polaroid is
View SolutionUnpolarized light with intensity I0 is incident on combination of two polarizers as shown . The intensity of the light after passage through both the polaroids will be.
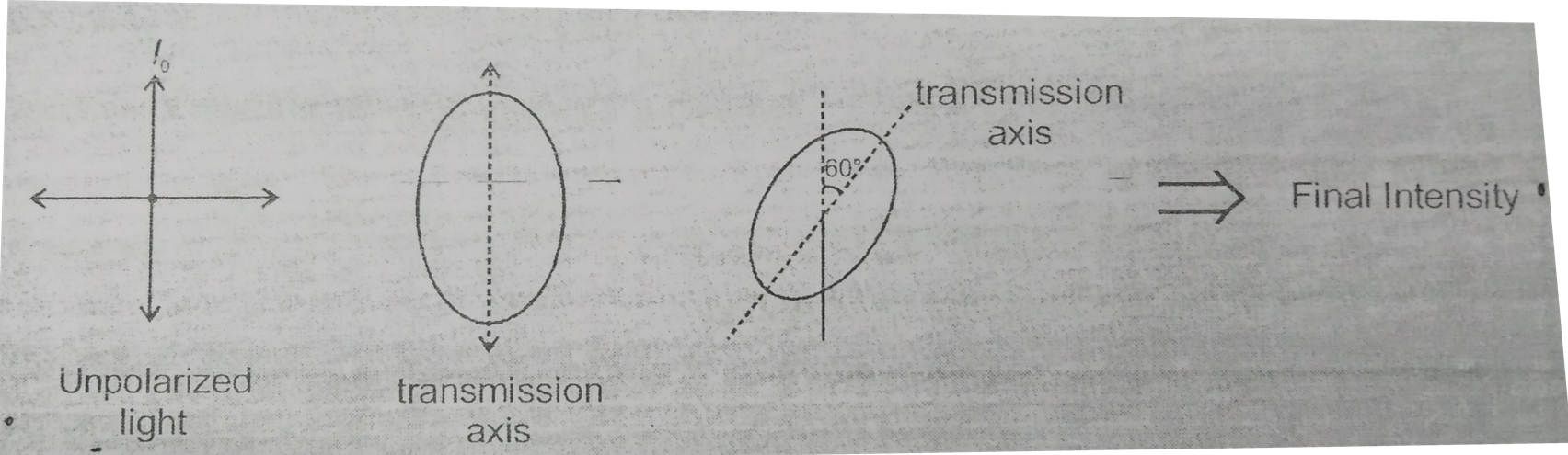
View SolutionTwo Polaroids P1 and P2 are placed with their axis perpendicular to eachother. Unpolarised light I0 is nicident on P1. A third polaroid P3 is kept in between P1 and P2 such that its axis makes an angle 45∘ with that of P1. The intensity of transmitted light through P2 is
View SolutionTwo Polaroids P1 and P2 are placed with their axis perpendicular to eachother. Unpolarised light I0 is nicident on P1. A third polaroid P3 is kept in between P1 and P2 such that its axis makes an angle 45∘ with that of P1. The intensity of transmitted light through P2 is
View SolutionA system of three polarizers P1,P2,P3 is set up such that pass axis of P3 is crossed with respect to that of P1. The pass axis of P2 is inclined at 60∘ to the pass axis of P3. When a beam of unpolarized light of intensity I0 is incident on P1, the intensity of light transmitted by the three polarizes is I. The ratio (Io/I) equals (nearly) : a) 5.33 b) 16.00 c) 10.67 d) 1.80
View SolutionAn unpolarized light with intensity 2I0 is passed through a polaroid. The resultant intensity of the transmitted light will be
View SolutionTwo polaroids P1 and P2 are placed with their axis perpendicular to each other. Unpolarised light I0 is incident of P1. A third polaroid P3 is kept in between P1 and P2 such that its axis makes an angle 45∘ with that of P1. The intensity of transmitted light through P2 is :
View SolutionUnpolarised light of intensity I is passed through a polaroid. What is the intensity of emerging polaroised light ?
View SolutionTwo polaroids are crossed. If now one of them is rotated through 30∘ and unpolarised light of intensity l0 is incident on the first polaroid, then the intensity of transmitted light will be
View SolutionA polaroid is placed at 45∘ to an incoming light of intensity I0 Now, the intensity of light passing through polaroid after polarization would be
View Solution