Species - Area Relationship
Step-by-Step Solution for Species-Area Relationship
1. Definition of Species-Area Relationship:
The species-area relationship describes how the number of species (species richness) increases with the area being studied. This concept was first proposed by Alexander von Humboldt.
2. Graph Representation:
- The relationship is often represented graphically, with the area (A) plotted on the x-axis and species richness (S) on the y-axis.
- The graph typically shows a curve that indicates that as area increases, the number of species also increases.
3. Mathematical Representation:
- The relationship can be expressed mathematically as:
S=CAZ
where:
- S = species richness
- C = y-intercept (a constant)
- A = area
- Z = slope of the line
4. Log-Log Transformation:
- To linearize the relationship, we can take the logarithm of both sides:
logS=logC+ZlogA
- This transformation allows for easier analysis and interpretation of the data.
5. Understanding the Variables:
- Species Richness (S): The number of different species present in a given area.
- Area (A): The size of the area being studied.
- Constant (C): Represents the number of species in a unit area.
- Slope (Z): Indicates how steeply species richness increases with area. A slope between 0.1 to 0.2 is typical for entire continents.
6. Implications of the Relationship:
- As the explored area increases, the likelihood of encountering new species also increases due to more diverse habitats and ecological niches.
- This relationship is crucial for biodiversity conservation, as it highlights the importance of protecting larger areas to maintain species richness.
The species-area relationship describes how the number of species (species richness) increases with the area being studied. This concept was first proposed by Alexander von Humboldt.
- The relationship is often represented graphically, with the area (A) plotted on the x-axis and species richness (S) on the y-axis.
- The graph typically shows a curve that indicates that as area increases, the number of species also increases.
- The relationship can be expressed mathematically as:
S=CAZ
where:
- S = species richness
- C = y-intercept (a constant)
- A = area
- Z = slope of the line
- To linearize the relationship, we can take the logarithm of both sides:
logS=logC+ZlogA
- This transformation allows for easier analysis and interpretation of the data.
- Species Richness (S): The number of different species present in a given area.
- Area (A): The size of the area being studied.
- Constant (C): Represents the number of species in a unit area.
- Slope (Z): Indicates how steeply species richness increases with area. A slope between 0.1 to 0.2 is typical for entire continents.
- As the explored area increases, the likelihood of encountering new species also increases due to more diverse habitats and ecological niches.
- This relationship is crucial for biodiversity conservation, as it highlights the importance of protecting larger areas to maintain species richness.
Topper's Solved these Questions
Similar Questions
Alexander von Humboldt is associated with
(a.) species - area relationship
(b.) log S = log C + z logA
(C.) Within a region , species richness increases with the increasing limited explored area.
(d.) Increased diversity contributes to higher productivity.
(e.) Species diversity increases with the loss of keystone species.
View SolutionMatch the items in Column-I with those in Column-II and choose the correct option from the codes given below:
Column-IColumn-II(a)Ecosystem Experiments (i)Norman Myers(b)Species-Area relationships(ii)Alexander von Humboldt(c)Biodiversity hotspots(iii)Paul Ehrlich(d)Rivet Popper Hypothesis(iv)David Tilman
View Solution
Knowledge Check
On a logarithmic scale, the species area relationship is a straight line described by the equation log S = log C + Z log A.
What does S, C, Z and A represent in the given equation? Select the correct answer from the codes given below.
Species richness=1Slope of the line=2Y-intercept=3Area=4
a) 1 2 3 4
C S Z A
b) 1 2 3 4
S Z C A
c) 1 2 3 4
Z S C A
d) 1 2 3 4
A C S ZAa) 1234CSZABb) 1234SZCACc) 1234ZSCADd) 1234ACSZIntroduction of alien species into new area poses a threat to extinction of indigenous species due to
(a) their high nutrient requirement
(b) their symbiotic relationship
(c) absence of their natural predators
(d) more intraspecific competition.Atheir high nutrient requirementBtheir symbiotic relationshipCabsence of their natural predatorsDmore intraspecific competition.
Match column I with column II and select the correct option from the given codes.
Column IColumn IIA) Rivet Popper hypothesis(i)Paul EhrlichB) Long-term ecosystem <br> experiments using outdoor plots(ii)David TilmanC) Species-area relationships(iii)Alexander von <br> Humboldt
(a) A-(iii), B-(i), C-(ii)
(b) A-(i), (ii), C-(iii)
(c) A-(i), B-(iii), C-(ii)
(d) A-(ii), B-(iii), C-(i)
View SolutionFill in the blanks with suitable words in following statements.
(i) Colombia located near the equator has about…….. Species of birds.
(ii) More than 1,25,000 species of………. have been reported in Aamzonian rain forest.
(iii) Favourable and less seasonal enviroments has helped.............organism to gain more niche specialisation.
(iv) Value of slope of regression (Z) of species-area relationship lies in the range of............molluscs in New York state.
View SolutionThe following graph shows the species area relationship. Answers the Questions as directed.
(a) Name the naturalist who studied the kind of relationship shown in the graph.
Write the observations made by him.
(b) Write the situations as discovered by the ecologists when the value of 'Z' (slope of the line) lies between
(i) 0.1 and 0.2, (ii) 0.6 and 1.2
What does 'Z' stand for ?
(c ) When would the slope of the line 'b' become steeper ?
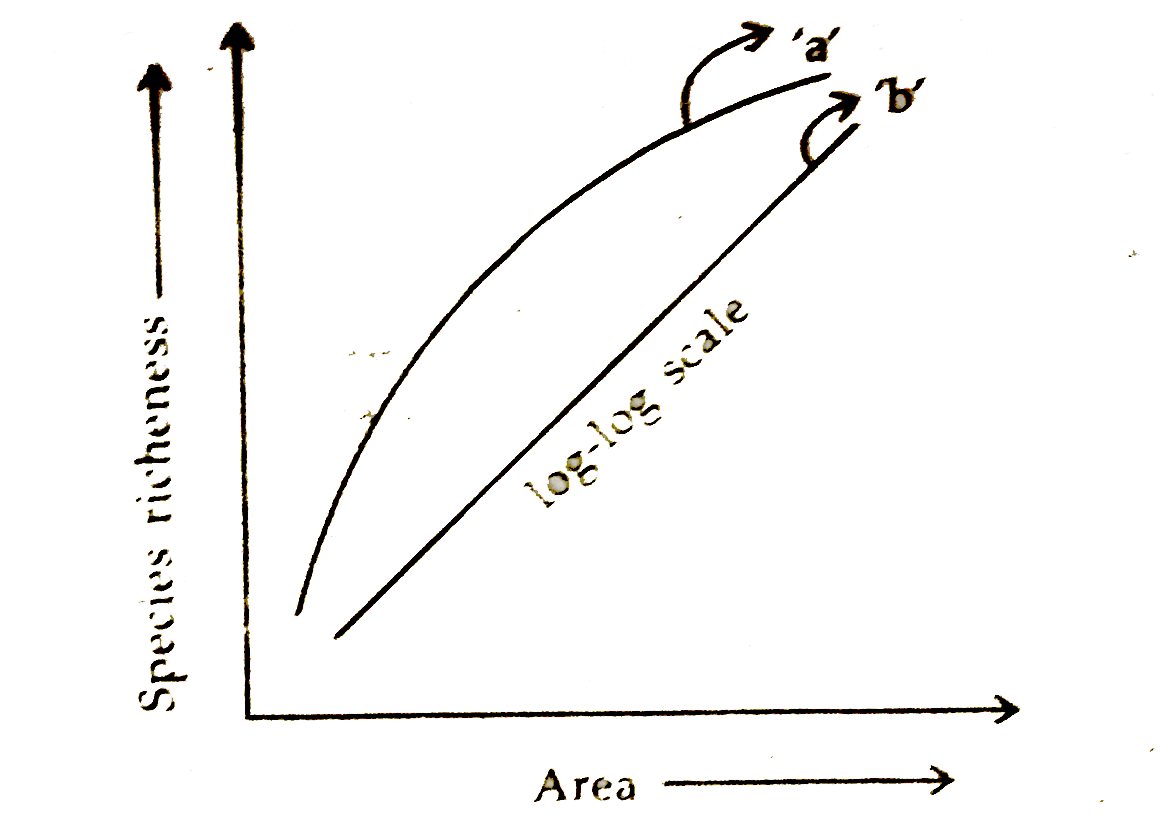
View SolutionThe following graph shows the species area relationship. Answers the Questions as directed.
(a) Name the naturalist who studied the kind of relationship shown in the graph.
Write the observations made by him.
(b) Write the situations as discovered by the ecologists when the value of 'Z' (slope of the line) lies between
(i) 0.1 and 0.2, (ii) 0.6 and 1.2
What does 'Z' stand for ?
(c ) When would the slope of the line 'b' become steeper ?

View SolutionWhich one of the following plants shows a very close relationship with a species of moth, where none of the two can complete its life cycle without the other
View SolutionWhich one of the following plants shows a very close relationship with a species of moth, where none of the two can complete its life cycle without the other
View Solution