If (3,−6) is the mid-poiint of the line segment joing (0,0)and(x,y), then the point (x,y) is
(−3,6)
(6,6)
(6,−12)
(32,−3)
To solve the problem, we need to find the coordinates of the point (x,y) given that (3,−6) is the midpoint of the line segment joining the points (0,0) and (x,y).
1. Understand the Midpoint Formula:
The midpoint M of a line segment joining two points (x1,y1) and (x2,y2) is given by:
M=(x1+x22,y1+y22)
2. Identify the Points:
Here, we have:
- Point P=(0,0) (which we can denote as (x1,y1))
- Point Q=(x,y) (which we can denote as (x2,y2))
- Midpoint M=(3,−6)
3. Set Up the Equations:
According to the midpoint formula, we can set up the following equations:
x1+x22=3(1)
y1+y22=−6(2)
4. Substitute Known Values:
Substitute x1=0 and y1=0 into the equations:
- From equation (1):
0+x2=3
- From equation (2):
0+y2=−6
5. Solve for x:
Multiply both sides of equation (1) by 2:
0+x=6⟹x=6
6. Solve for y:
Multiply both sides of equation (2) by 2:
0+y=−12⟹y=−12
7. Final Coordinates:
Therefore, the coordinates of the point (x,y) are:
(x,y)=(6,−12)
Conclusion:
The point (x,y) is (6,−12).
The midpoint M of a line segment joining two points (x1,y1) and (x2,y2) is given by:
M=(x1+x22,y1+y22)
Here, we have:
- Point P=(0,0) (which we can denote as (x1,y1))
- Point Q=(x,y) (which we can denote as (x2,y2))
- Midpoint M=(3,−6)
According to the midpoint formula, we can set up the following equations:
x1+x22=3(1)
y1+y22=−6(2)
Substitute x1=0 and y1=0 into the equations:
- From equation (1):
0+x2=3
- From equation (2):
0+y2=−6
Multiply both sides of equation (1) by 2:
0+x=6⟹x=6
Multiply both sides of equation (2) by 2:
0+y=−12⟹y=−12
Therefore, the coordinates of the point (x,y) are:
(x,y)=(6,−12)
The point (x,y) is (6,−12).
Topper's Solved these Questions
- CBSE BOARDS 2020BOOK - X BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - CBSE BOARDS 2020EXERCISE - SECTION B
22 Videos
- CBSE BOARDS 2020BOOK - X BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - CBSE BOARDS 2020EXERCISE - SECTION C
33 Videos
- SAMPLE PAPER 2019BOOK - X BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - SAMPLE PAPER 2019EXERCISE - SECTION-C
5 Videos
Similar Questions
If (3,-6) is the mid point of the line segment joining (0,0) and (x,y), then the point (x,y) is
(A) (-3,6)
(B) (6,-6)
(C) (6,-12)
(D) (3/2,-3)
View SolutionIf (3,-6) is the mid point of the line segment joining (0,0) and (x,y), then the point (x,y) is
(A) (-3,6)
(B) (6,-6)
(C) (6,-12)
(D) (3/2,-3)
View SolutionFind the mid point of the line segment joining (3,6) and (5,-2).
View SolutionIf P(x, 6) is the mid-point of the line segment joining A(6, 5) and B(4, y) , find y .
View SolutionIf P(2, 6) is the mid-point of the line segment joining A(6, 5) and B(4, y) , find y .
View SolutionShow that the mid-point of the line segment joining the points (5,7) and (3,9) is also the mid-point of the line segment joining the points (8,6) and (0,10) .
View SolutionM is the mid-point of the line segment joining the points A(0, 4) and B(6, 0). M also divides the line segment OP in the ratio 1:3. Find :
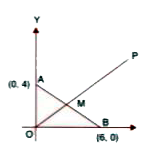
co-ordinates of M
View SolutionM is the mid-point of the line segment joining the points A(0, 4) and B(6, 0). M also divides the line segment OP in the ratio 1:3. Find :
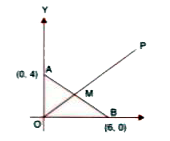
co-ordinates of P
View SolutionM is the mid-point of the line segment joining the points A(0, 4) and B(6, 0). M also divides the line segment OP in the ratio 1:3. Find :
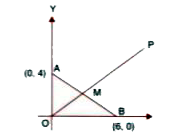
length of BP
View SolutionThe slope of a line which passes through the origin the mid-point of the line segment joining the points (0,−4) and (8,0) is
View Solution
