Let z be a complex number satisfying equation zp−z−q=0, where p,q∈N,then (A) if p=q , then number of solutions of equation will be infinite. (B) if p=q , then number of solutions of equation will be finite. (C) if p≠q , then number of solutions of equation will be p+q+1. (D) if p≠q , then number of solutions of equation will be p+q.
To solve the equation zp−z−q=0 where p,q∈N, we will analyze the cases when p=q and p≠q.
Step 1: Rewrite the equation
We start with the equation:
zp−z−q=0
This can be rewritten as:
zp=z−q
Step 2: Multiply both sides by zq
To eliminate the negative exponent, we multiply both sides by zq:
zp+q=1
Step 3: Analyze the solutions for zp+q=1
The equation zp+q=1 represents the (p+q)-th roots of unity. The solutions to this equation are given by:
z=ei2kπp+qfor k=0,1,2,…,p+q−1
This means there are p+q distinct solutions.
Step 4: Consider the case when p=q
If p=q, then we have:
z2p=1
The solutions to this equation are the 2p-th roots of unity, which gives us 2p distinct solutions.
Step 5: Consider the case when p≠q
If p≠q, we have already established that:
zp+q=1
Thus, the number of solutions in this case is p+q.
Conclusion
From the analysis, we can summarize the findings:
- If p=q, the number of solutions is infinite (since zp=z−p leads to an infinite number of roots).
- If p≠q, the number of solutions is p+q+1.
Final Answer
Thus, the correct options are:
- (A) if p=q, then the number of solutions of the equation will be infinite.
- (C) if p≠q, then the number of solutions of the equation will be p+q+1.
We start with the equation:
zp−z−q=0
This can be rewritten as:
zp=z−q
To eliminate the negative exponent, we multiply both sides by zq:
zp+q=1
The equation zp+q=1 represents the (p+q)-th roots of unity. The solutions to this equation are given by:
z=ei2kπp+qfor k=0,1,2,…,p+q−1
This means there are p+q distinct solutions.
If p=q, then we have:
z2p=1
The solutions to this equation are the 2p-th roots of unity, which gives us 2p distinct solutions.
If p≠q, we have already established that:
zp+q=1
Thus, the number of solutions in this case is p+q.
From the analysis, we can summarize the findings:
- If p=q, the number of solutions is infinite (since zp=z−p leads to an infinite number of roots).
- If p≠q, the number of solutions is p+q+1.
Thus, the correct options are:
- (A) if p=q, then the number of solutions of the equation will be infinite.
- (C) if p≠q, then the number of solutions of the equation will be p+q+1.
Topper's Solved these Questions
Similar Questions
Knowledge Check
The following diagram represents the solution of two sets P and Q on the number line:
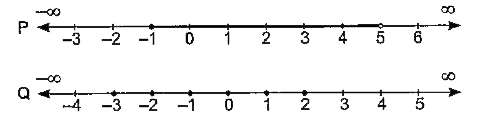
The solution set of Q in set builder form is:A{x:−3<x<2,x∈R}B{x:−3≤x≤2,x∈I}C{x:−3<x≤2,x∈N}D{x:−3≤x<2,x∈W}The following diagram represents the solution of two sets P and Q on the number line:
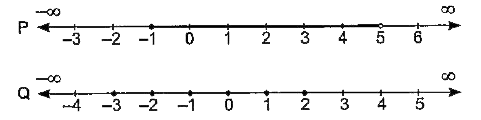
The solution set of Q in roaster form is:A{−2,−1,0,1}B{1, 2}C{−3,−2,−1,0,1,2}D{0,1,2}The following diagram represents the solution of two sets P and Q on the number line:
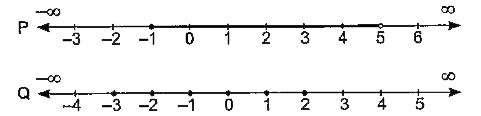
The solution set of P in set-builder form isA{x:−1<x≤5,x∈R}B{x:−1≤x<5,x∈R}C{x:−1≤x≤5,x∈R}D{x:−1<x<5,x∈R}
Let p,qε{1,2,3,4}. The number of equations of the form px2+qx+1=0 having real roots, is
View SolutionFind the values of p and q for which the following system of equations has infinite number of solutions: 2x+3y=7, (p+q)x+(2p−q)y=21
View SolutionFind the values of p and q for which the following system of equations has infinite number of solutions: 2x+3y=7, (p+q)x+(2p−q)y=21
View SolutionFor a complex number z, the equation z2+(p+iq)z+r+ is =0 has a real root (where p, q, r, s are non - zero real numbers and i2−1), then
View SolutionFind p, where p,q belongs to R If p and q are the roots of equation x2−px+q=0 then p=,
View Solution
