Let f,g:[−1,2]→R be continuous functions which are twice differentiable on the interval (-1, 2). Let the values of f and g at the points –1, 0 and 2 be as given in the following table : x=−1x=0x=2f(x)360g(x)01−1 In each of the intervals (-1,0) and (0, 2) the function (f – 3g)'' never vanishes. Then the correct statement(s) is(are)
To solve the problem, we need to analyze the functions f and g based on the given values and the conditions provided. We will define a new function h(x)=f(x)−3g(x) and evaluate it at the specified points.
1. Define the function h(x):
h(x)=f(x)−3g(x)
2. Evaluate h(x) at the given points:
- For x=−1:
h(−1)=f(−1)−3g(−1)=3−3(0)=3
- For x=0:
h(0)=f(0)−3g(0)=6−3(1)=6−3=3
- For x=2:
h(2)=f(2)−3g(2)=0−3(−1)=0+3=3
3. Summary of evaluations:
- h(−1)=3
- h(0)=3
- h(2)=3
4. Check the conditions for Rolle's Theorem:
Since h(−1)=h(0)=h(2)=3, the function h(x) is continuous on the closed interval (−1,2) and differentiable on the open interval (−1,2). Therefore, by Rolle's Theorem, there exists at least one point c∈(−1,2) such that h′(c)=0.
5. Analyze the second derivative:
We know that (f−3g)′′ never vanishes in the intervals (−1,0) and (0,2). This implies that h′′(x) does not change sign in these intervals, indicating that h′(x) is either always increasing or always decreasing.
6. Conclusion:
Since h′(c)=0 for some c and h′′(x) does not change sign, it implies that h′(x) has a local extremum at c. Thus, the function h(x) is constant in the intervals considered.
Final Statements:
The correct statements based on the analysis are:
- The function h(x) has the same value at the endpoints and at the critical point, confirming the conditions of Rolle's Theorem.
- The second derivative h′′(x) does not vanish, ensuring the behavior of h′(x) is consistent across the intervals.
h(x)=f(x)−3g(x)
- For x=−1:
h(−1)=f(−1)−3g(−1)=3−3(0)=3
- For x=0:
h(0)=f(0)−3g(0)=6−3(1)=6−3=3
- For x=2:
h(2)=f(2)−3g(2)=0−3(−1)=0+3=3
- h(−1)=3
- h(0)=3
- h(2)=3
Since h(−1)=h(0)=h(2)=3, the function h(x) is continuous on the closed interval (−1,2) and differentiable on the open interval (−1,2). Therefore, by Rolle's Theorem, there exists at least one point c∈(−1,2) such that h′(c)=0.
We know that (f−3g)′′ never vanishes in the intervals (−1,0) and (0,2). This implies that h′′(x) does not change sign in these intervals, indicating that h′(x) is either always increasing or always decreasing.
Since h′(c)=0 for some c and h′′(x) does not change sign, it implies that h′(x) has a local extremum at c. Thus, the function h(x) is constant in the intervals considered.
The correct statements based on the analysis are:
- The function h(x) has the same value at the endpoints and at the critical point, confirming the conditions of Rolle's Theorem.
- The second derivative h′′(x) does not vanish, ensuring the behavior of h′(x) is consistent across the intervals.
Similar Questions
Let f,g:[−1,2]→ be continuous functions which are twice differentiable on the interval (-1,2). Let the values of f and g at the points and 2 be as given in the following table:
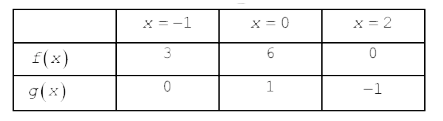
In each of the intervals (-1,0) and (0, 2) the function (f-3g) never vanishes. Then the correct statements(s) is(are) :
View SolutionLet f(x) be a differentiable function in the interval (0, 2) then the value of ∫20f(x)dx
View SolutionLet f:[0,1]→[0,1]be a continuous function such that f(f(x))=1forallx∈[0,1]then:
View SolutionLet f:[0,1]→R be a function. Suppose the function f is twice differentiable, f(0)=f(1)=0 and satisfies f''(x)−2f'(x)+f(x)≥ex,x∈[0,1] Which of the following is true for 0<x<1?
View SolutionLet f(x)=cot−1g(x)] where g(x) is an increasing function on the interval (0,π) Then f(x) is
View SolutionLet f(x) be a function satisfyingf'(x)=f(x) withf(0)=1 and g(x) be a function that satisfies f(x)+g(x)=x2 . Then the value of the integral ∫10f(x)g(x)dx, is
View SolutionIf f:R→R is a differentiable function such that f(x)>2f(x) for all x∈R and f(0)=1, then
View SolutionLet f:[0,1]→R be a continuous function then the maximum value of ∫10f(x).x2dx−∫10x.(f(x))2dx for all such function(s) is:
View SolutionIf f(x) is a twice differentiable function such that f(a)=0, f(b)=2, f(c)=-1,f(d)=2, f(e)=0 where a < b < c < d e, then the minimum number of zeroes of g(x)=f'(x)2+f''(x)f(x) in the interval [a, e] is
View SolutionLet f(x) be a continuous function for all x∈Randf'(0)=1 then g(x)=f(|x|)−√1−cos2x2,atx=0,
View Solution
