A swimmer S is in the sea at a distance d km from the closest point A on a straight shore The house of the swimmer is at a distance Lkm from A on the shore. He can swim at a speed of ukm/hr and walk at a speed of vkm/hr(v>u). At what point on the shore should he land so that he reaches his house in the shortest possible time?
To solve the problem, we need to find the point on the shore where the swimmer should land in order to reach his house in the shortest possible time. Let's break down the solution step by step.
Step 1: Understand the Problem
We have:
- A swimmer S at a distance d km from point A on the shore.
- The swimmer's house is at a distance L km from point A.
- The swimmer swims at a speed of u km/hr and walks at a speed of v km/hr (where v>u).
Step 2: Define the Variables
Let:
- x = distance from point A to the landing point B on the shore.
- The distance from B to the house H will then be (L−x) km.
Step 3: Calculate Distances
Using the Pythagorean theorem, the distance SB (from S to B) can be calculated as:
\( SB = \sqrt{d^2 + x^2} \)
Step 4: Write the Time Functions
The total time T taken by the swimmer to reach his house can be expressed as:
\( T = \text{Time to swim to B} + \text{Time to walk to H} \)
\( T = \frac{SB}{u} + \frac{BH}{v} \)
Substituting the distances:
\( T = \frac{\sqrt{d^2 + x^2}}{u} + \frac{L - x}{v} \)
Step 5: Differentiate the Time Function
To minimize the time, we need to differentiate T with respect to x and set the derivative equal to zero:
\( \frac{dT}{dx} = \frac{1}{u} \cdot \frac{x}{\sqrt{d^2 + x^2}} - \frac{1}{v} = 0 \)
Step 6: Solve for x
Rearranging the equation gives:
\( \frac{x}{\sqrt{d^2 + x^2}} = \frac{u}{v} \)
Squaring both sides:
\( \frac{x^2}{d^2 + x^2} = \frac{u^2}{v^2} \)
Cross-multiplying:
\( v^2 x^2 = u^2 (d^2 + x^2) \)
Expanding:
\( v^2 x^2 = u^2 d^2 + u^2 x^2 \)
Rearranging gives:
\( (v^2 - u^2) x^2 = u^2 d^2 \)
Thus, we find:
\( x^2 = \frac{u^2 d^2}{v^2 - u^2} \)
Taking the square root:
\( x = \frac{ud}{\sqrt{v^2 - u^2}} \)
Step 7: Conclusion
The swimmer should land at a point B on the shore that is x=ud√v2−u2 km away from point A to reach his house in the shortest possible time.
We have:
- A swimmer S at a distance d km from point A on the shore.
- The swimmer's house is at a distance L km from point A.
- The swimmer swims at a speed of u km/hr and walks at a speed of v km/hr (where v>u).
Let:
- x = distance from point A to the landing point B on the shore.
- The distance from B to the house H will then be (L−x) km.
Using the Pythagorean theorem, the distance SB (from S to B) can be calculated as:
\( SB = \sqrt{d^2 + x^2} \)
The total time T taken by the swimmer to reach his house can be expressed as:
\( T = \text{Time to swim to B} + \text{Time to walk to H} \)
\( T = \frac{SB}{u} + \frac{BH}{v} \)
Substituting the distances:
\( T = \frac{\sqrt{d^2 + x^2}}{u} + \frac{L - x}{v} \)
To minimize the time, we need to differentiate T with respect to x and set the derivative equal to zero:
\( \frac{dT}{dx} = \frac{1}{u} \cdot \frac{x}{\sqrt{d^2 + x^2}} - \frac{1}{v} = 0 \)
Rearranging the equation gives:
\( \frac{x}{\sqrt{d^2 + x^2}} = \frac{u}{v} \)
Squaring both sides:
\( \frac{x^2}{d^2 + x^2} = \frac{u^2}{v^2} \)
Cross-multiplying:
\( v^2 x^2 = u^2 (d^2 + x^2) \)
Expanding:
\( v^2 x^2 = u^2 d^2 + u^2 x^2 \)
Rearranging gives:
\( (v^2 - u^2) x^2 = u^2 d^2 \)
Thus, we find:
\( x^2 = \frac{u^2 d^2}{v^2 - u^2} \)
Taking the square root:
\( x = \frac{ud}{\sqrt{v^2 - u^2}} \)
The swimmer should land at a point B on the shore that is x=ud√v2−u2 km away from point A to reach his house in the shortest possible time.
Similar Questions
A boy goes to a school from his home at a speed of x km/hr and comes back at a speed of y km/hr, then the average speed is given by
View SolutionA car travels a distance AB at a speed of 40 km/hr and returns to A at a speed of 30 km/hr. What is the average speed for the whole journey?
View SolutionDoes the escape speed of a body from the earth depend on.
(i)mass of the body
(ii)the location from where it is projected
(iii)the direction of projection
(iv)the height of the location from where the body is launched?
View SolutionA man starting from point P cross a 4km wide lagoon and reaches point Q in the shortest possible time by the path shown in the figure. If the person swims at a speed of 3km//hr and walks at a speed of 4km//hr, then find his time fo journey (in minutes).
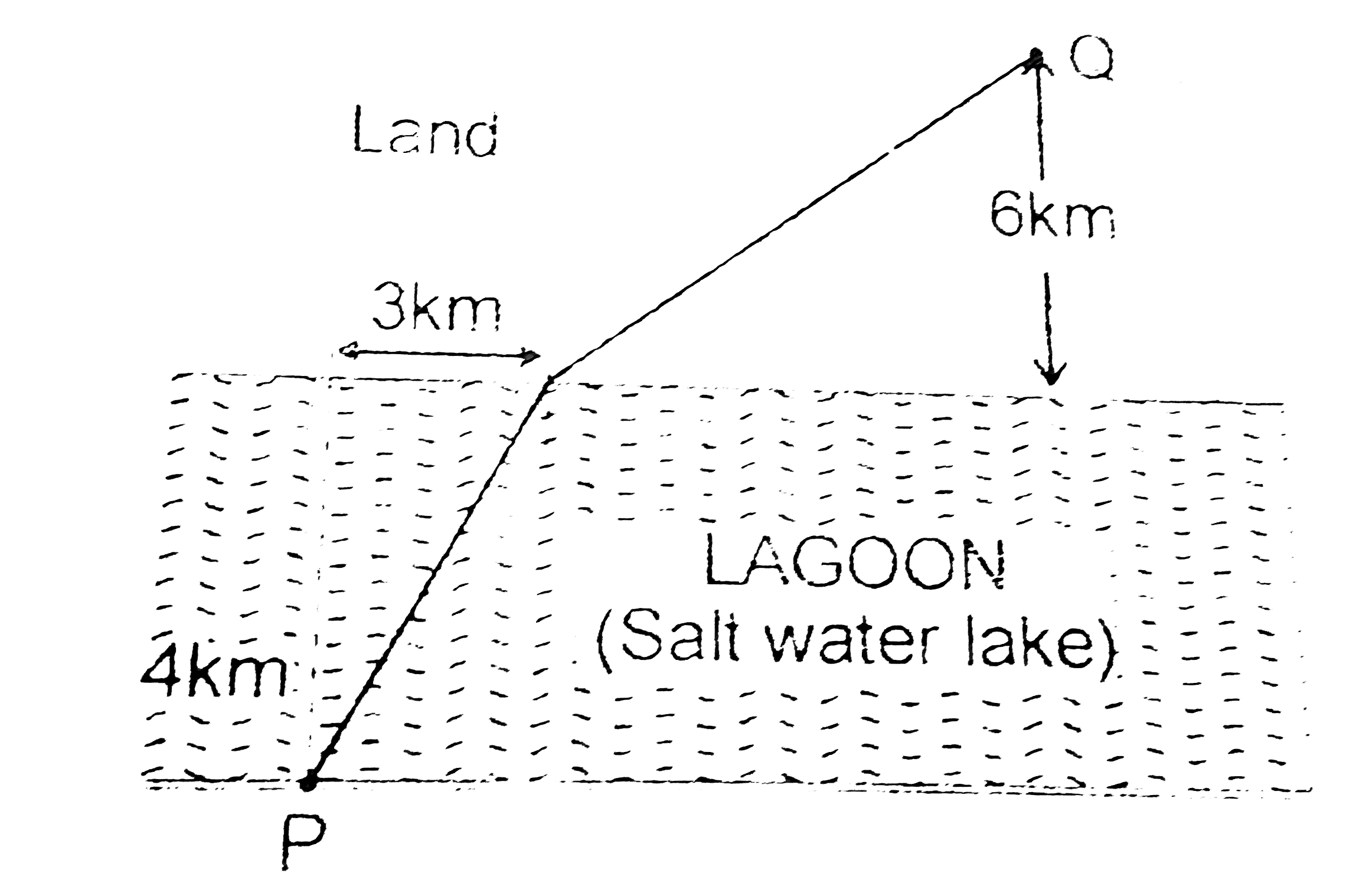 .
.
View SolutionA man travels at a speed of 20 Km/hr. and then returns at a speed of 30 Km/hr. His average speed of whole journey is
View SolutionIf a bus travels a distance of 30 km in 45 minutes. What will be its speed in:
km/hr
View SolutionOne car moving on a straight road covers one third of the distance with 20 km/hr and the rest with 60 km/hr. The average speed is
View SolutionOne day a boy walked from his house to his school at the speed of 4 km/hr and he reached ten minutes late to the school. Next day, he ran at the speed of 8 km/hr and was 5 minutes early to the school. Find the distance between his house and school.
View SolutionThe stream of a river is flowing with a speed of 2km/h. A swimmer can swim at a speed of 4km/h. What should be the direction of the swimmer with respect to the flow of the river ot cross the river straight ?
View SolutionA driver having a definite reaction time is capable of stopping his car over a distance of 30 m on seeing a red traffic signal, when the speed of the car is 72 km/hr andover a distance of 10 m when the speed is 36 km/hr. Find the distance over which he can stop the car if it were running at a speed of 54 km/hr. Assume that his reaction time and the deceleration of the car remains same in all the three cases.
View Solution
