Let f(x)={x35,ifx≤1and(x−2)3,ifx>1 Then the number of critical points on the graph of the function is___
To find the number of critical points of the function f(x), we first need to understand the function's definition and then determine where its derivative is either zero or undefined.
Step 1: Define the function
The function is given as:
f(x)={x3/5if x≤1(x−2)3if x>1
Step 2: Find the derivative of each piece
We will differentiate each piece of the function separately.
1. For x≤1:
f(x)=x3/5
To find the derivative:
f′(x)=35x−2/5=35√(5)x2
2. For x>1:
f(x)=(x−2)3
To find the derivative:
f′(x)=3(x−2)2
Step 3: Find critical points
Critical points occur where f′(x)=0 or where f′(x) is undefined.
1. For x≤1:
f′(x)=35√(5)x2 is undefined at x=0
Setting f′(x)=0:
35√(5)x2=0 has no solution.
Thus, the critical point in this interval is x=0.
2. For x>1:
f′(x)=3(x−2)2
Setting f′(x)=0:
3(x−2)2=0⟹(x−2)2=0⟹x=2
Thus, the critical point in this interval is x=2.
Step 4: Check the point of transition
We also need to check the point where the definition of the function changes, which is at x=1. We need to ensure that the derivative exists at this point.
- For x=1:
f′(1)=35√(5)12=35 (exists)
Step 5: Count the critical points
The critical points we found are:
1. x=0 (from x≤1)
2. x=1 (transition point)
3. x=2 (from x>1)
Thus, the total number of critical points is 3.
Final Answer
The number of critical points on the graph of the function is 3.
The function is given as:
f(x)={x3/5if x≤1(x−2)3if x>1
We will differentiate each piece of the function separately.
f(x)=x3/5
To find the derivative:
f′(x)=35x−2/5=35√(5)x2
f(x)=(x−2)3
To find the derivative:
f′(x)=3(x−2)2
Critical points occur where f′(x)=0 or where f′(x) is undefined.
f′(x)=35√(5)x2 is undefined at x=0
Setting f′(x)=0:
35√(5)x2=0 has no solution.
Thus, the critical point in this interval is x=0.
f′(x)=3(x−2)2
Setting f′(x)=0:
3(x−2)2=0⟹(x−2)2=0⟹x=2
Thus, the critical point in this interval is x=2.
We also need to check the point where the definition of the function changes, which is at x=1. We need to ensure that the derivative exists at this point.
f′(1)=35√(5)12=35 (exists)
The critical points we found are:
1. x=0 (from x≤1)
2. x=1 (transition point)
3. x=2 (from x>1)
The number of critical points on the graph of the function is 3.
Similar Questions
Knowledge Check
The function y=f(x) is graphed in the standard (x, y) coordinate plane below.
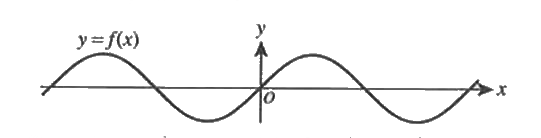
The points on the graph of the function y=3+f(x−1) can be obtained from the points on y=f(x) by a shift of:A1 unit to the right and 3 unit up.B1 unit to the right and 3 unit down.C3 unit to the right and 1 unit up.D3 unit to the right and 1 unit down.
Let f(x) = sin x (1+cos x) ,x∈(0,2π). Find the number of critical points of f(x) . Also identify which of these critical points are points of Maxima/Minima.
View SolutionLet f(x)=[x3−3], where [.] is the greatest integer function, then the number of points in the interval (1,2) where function is discontinuous is (A) 4 (B) 5 (C) 6 (D) 7
View SolutionDraw a graph of the function f(x)=cos−1(4x3−3x),x∈[−1,1] and find the ara enclosed between the graph of the function and the x-axis varies from 0 to 1.
View SolutionFind the critical points of f(x)=x2/3(2x−1)
View SolutionLet f be a composite function.of x defined by f(u)=1u3−6u2+11u−6 where u(x)=1x.Then the number of points x where f is discontinuous is
View SolutionConsider the function f(x)={2x+3,x≤1and−x2+6,x>1} Then draw the graph of the function y=f(x),y=f(|x|)andy=|f(x)|.
View SolutionFind the points of discontinuity, if any, of the following function: f(x)={|x|+3ifx≤−3,−2x,if−3<x<3,6x+2,ifx>3
View Solution
