A factory D is to be connected by a road with a straight railway line on which a town A is situated. The distance DB of the factory to the railway line is 5√3km. Length AB of the railway line is 20km. Freight charges on the road are twice the charges on the railway. The point P(AP<AB) on the railway line should the road DP be connected so as to ensure minimum freight charges from the factory to the town is BP=5km (b) AP=5km BP=7.5km (d) none of these
To solve the problem step by step, we will follow a systematic approach involving geometry and calculus.
Step 1: Understand the Problem
We have a factory D that is 5√3 km away from a railway line at point B. The length of the railway line from A to B is 20km. We need to find the point P on the railway line such that the total freight charges from the factory to the town A are minimized. The freight charges on the road are twice that of the railway.
Step 2: Set Up the Diagram
1. Draw a horizontal line representing the railway line with points A and B.
2. Mark point B at the origin (0,0).
3. Point A will be at (20,0) since AB=20km.
4. Mark point D at (0,5√3) since it is 5√3km above point B.
Step 3: Define the Variables
Let P be the point on the railway line such that BP=xkm. Therefore, the distance AP will be 20−xkm.
Step 4: Calculate the Distances
1. The distance DP can be calculated using the Pythagorean theorem:
\(
DP = \sqrt{(x - 0)^2 + (0 - 5\sqrt{3})^2} = \sqrt{x^2 + (5\sqrt{3})^2} = \sqrt{x^2 + 75}
\)
Step 5: Set Up the Cost Function
Let the cost per km on the railway be k. The cost for traveling from D to P (road) is 2k per km. Therefore, the total cost C can be expressed as:
\(
C = k(20 - x) + 2k\sqrt{x^2 + 75}
\)
This simplifies to:
\(
C = k(20 - x + 2\sqrt{x^2 + 75})
\)
Step 6: Differentiate the Cost Function
To minimize the cost, we differentiate C with respect to x:
\(
\frac{dC}{dx} = -k + k \cdot \frac{2x}{\sqrt{x^2 + 75}}
\)
Setting the derivative equal to zero for minimization:
\(
-k + k \cdot \frac{2x}{\sqrt{x^2 + 75}} = 0
\)
This simplifies to:
\(
\frac{2x}{\sqrt{x^2 + 75}} = 1
\)
Step 7: Solve for x
Squaring both sides:
\(
4x^2 = x^2 + 75
\)
\(
3x^2 = 75
\)
\(
x^2 = 25 \quad \Rightarrow \quad x = 5 \text{ (since distance cannot be negative)}
\)
Step 8: Calculate BP
Now, we find BP:
\(
BP = x = 5 \text{ km}
\)
Conclusion
The point P on the railway line should be such that BP=5km.
Final Answer
The correct option is (a) BP=5km.
We have a factory D that is 5√3 km away from a railway line at point B. The length of the railway line from A to B is 20km. We need to find the point P on the railway line such that the total freight charges from the factory to the town A are minimized. The freight charges on the road are twice that of the railway.
1. Draw a horizontal line representing the railway line with points A and B.
2. Mark point B at the origin (0,0).
3. Point A will be at (20,0) since AB=20km.
4. Mark point D at (0,5√3) since it is 5√3km above point B.
Let P be the point on the railway line such that BP=xkm. Therefore, the distance AP will be 20−xkm.
1. The distance DP can be calculated using the Pythagorean theorem:
\(
DP = \sqrt{(x - 0)^2 + (0 - 5\sqrt{3})^2} = \sqrt{x^2 + (5\sqrt{3})^2} = \sqrt{x^2 + 75}
\)
Let the cost per km on the railway be k. The cost for traveling from D to P (road) is 2k per km. Therefore, the total cost C can be expressed as:
\(
C = k(20 - x) + 2k\sqrt{x^2 + 75}
\)
This simplifies to:
\(
C = k(20 - x + 2\sqrt{x^2 + 75})
\)
To minimize the cost, we differentiate C with respect to x:
\(
\frac{dC}{dx} = -k + k \cdot \frac{2x}{\sqrt{x^2 + 75}}
\)
Setting the derivative equal to zero for minimization:
\(
-k + k \cdot \frac{2x}{\sqrt{x^2 + 75}} = 0
\)
This simplifies to:
\(
\frac{2x}{\sqrt{x^2 + 75}} = 1
\)
Squaring both sides:
\(
4x^2 = x^2 + 75
\)
\(
3x^2 = 75
\)
\(
x^2 = 25 \quad \Rightarrow \quad x = 5 \text{ (since distance cannot be negative)}
\)
Now, we find BP:
\(
BP = x = 5 \text{ km}
\)
The point P on the railway line should be such that BP=5km.
The correct option is (a) BP=5km.
Similar Questions
The point P(a,b) lies on the straight line 3x+2y=13 and the point Q(b,a) lies on the straight line 4x−y=5 , then the equation of the line PQ is
View SolutionThe point P(a,b) lies on the straight line 3x+2y=13 and the point Q(b,a) lies on the straight line 4x−y=5 , then the equation of the line PQ is
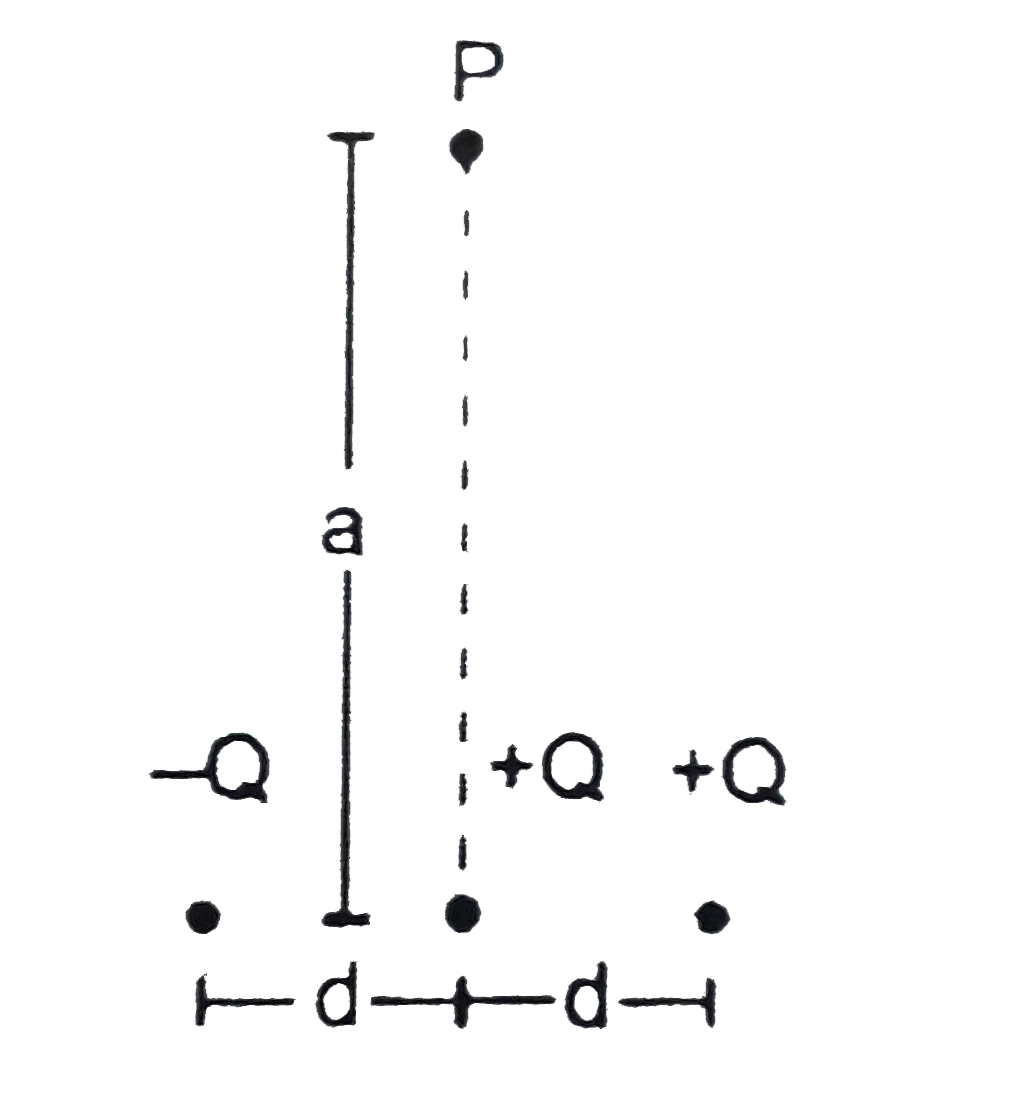
View SolutionThree point charges -Q, Q and Q are placed on a straight line with distance d between charges as shown. Find the magnitude of the electric field at the point P in the configuration shown which is at a distance a from middle charge Q in the system provided that a>>d. Take 2Qd=p.
View SolutionA particle of mass m carrying charge q is projected with velocity v from point P toward aninfinite line of charge from a distance a . Its speed reduces to zero momentarily at point Q, which is at a distance a/2 from the line of charge. If another particle with mass m and charge −q is projected with the same velosity v from P toward the line of charge, what will be its speed at Q ?
View SolutionP and Q are points on the line joining A(−2,5) and B(3,1) such that AP=PQ=QB . Then, the distance of the midpoint of PQ from the origin is 3 (b) √372 (b) 4 (d) 3.5
View SolutionP and Q are points on the line joining A(−2,5) and B(3,1) such that AP=PQ=QB . Then the distance of the midpoint of PQ from the origin is (a)3 (b) √372 (c) 4 (d) 3.5
View SolutionIf the straight line drawn through the point P(√3,2) and making an angle π6 with the x-axis meets the line √3x−4y+8=0 at Q, find the length of PQ.
View SolutionA train is running at 20 m/s on a railway line with radius of curvature 40,000 metres. The distance between the two rails is 1.5 metres. For safe running of train the elevation of outer rail over the inner rail is (g=10m/s2)
View SolutionTwo spheres A and B are charged with the charges of +10 and +20 coulomb respectively and separated by a distance of 80 cm. The electric field at point on the line joining the centres of the two spheres will be zero at a distance from the sphere A :
View SolutionA straight line passing through P(3,1) meets the coordinate axes at AandB . It is given that the distance of this straight line from the origin O is maximum. The area of triangle OAB is equal to
View Solution
