Two points O(0,0) and A(3,√3) with another point P form an equilateral triangle. Find the coordinates of P.
To find the coordinates of point P that, along with points O(0,0) and A(3,√3), forms an equilateral triangle, we can follow these steps:
Step 1: Calculate the distance OA
The distance OA can be calculated using the distance formula:
OA=√(x2−x1)2+(y2−y1)2
Substituting the coordinates of points O and A:
OA=√(3−0)2+(√3−0)2=√32+(√3)2=√9+3=√12
Step 2: Set the distances OP and AP
Since OAP is an equilateral triangle, the distances OP and AP are also equal to OA:
OP=AP=√12
Step 3: Write the equations for OP and AP
Let the coordinates of point P be (x,y).
1. For OP:
OP=√x2+y2=√12
Squaring both sides:
x2+y2=12(Equation 1)
2. For AP:
AP=√(x−3)2+(y−√3)2=√12
Squaring both sides:
(x−3)2+(y−√3)2=12
Expanding this:
(x2−6x+9)+(y2−2y√3+3)=12
Combining like terms:
x2+y2−6x−2y√3+12=12
Subtracting 12 from both sides:
x2+y2−6x−2y√3=0(Equation 2)
Step 4: Substitute Equation 1 into Equation 2
From Equation 1, we know x2+y2=12. Substitute this into Equation 2:
12−6x−2y√3=0
Rearranging gives:
6x+2y√3=12
Dividing the entire equation by 2:
3x+y√3=6
Step 5: Solve for y
Rearranging gives:
y√3=6−3x
Thus,
y=6−3x√3(Equation 3)
Step 6: Substitute Equation 3 back into Equation 1
Substituting y from Equation 3 into Equation 1:
x2+(6−3x√3)2=12
Expanding y2:
x2+(6−3x)23=12
Expanding further:
x2+36−36x+9x23=12
Multiplying through by 3 to eliminate the fraction:
3x2+36−36x+9x2=36
Combining like terms:
12x2−36x+36=36
Subtracting 36 from both sides:
12x2−36x=0
Factoring out 12x:
12x(x−3)=0
Step 7: Find the values of x
Setting each factor to zero gives us:
1. 12x=0 → x=0
2. x−3=0 → x=3
Step 8: Find corresponding y values
1. For x=0:
y=6−3(0)√3=6√3=2√3
2. For x=3:
y=6−3(3)√3=6−9√3=−3√3=−√3
Final Coordinates of P
Thus, the coordinates of point P can be:
1. P(0,2√3)
2. P(3,−√3)
2. x−3=0 → x=3
2. P(3,−√3)
Topper's Solved these Questions
Similar Questions
If (-4, 0) and (4,0) are two vertices of an equilateral triangle, find the coordinates of its third vertex.
View SolutionIf the point (0,0),(2,2√3), and (p,q) are the vertices of an equilateral triangle, then (p ,q) is
View SolutionShow that the points
(2,4),(2,6)(2+√3,5) form an equilateral triangle.View SolutionIf three points (0, 0), (3, √3) and (3, λ) form an equilateral triangle, then λ= (a) 2 (b) −3 (c) −4 (d) None of these
View SolutionShow that the points (a,a),(−a,−a) and (−√3a,√3a) are the vertices of an equilateral triangle. Also, find its area.
View SolutionProve that the points A(1,1),B(−1,−1)andC(√3,−√3) are the vertices of an equilateral triangle.
View SolutionIf (-4,3) and (4,3) are two vertices of an equilateral triangle, then find the coordinates of the third vertex, given that the origin lies in the interior of the triangle.
View SolutionIn the given figure, ΔABC is an equilateral triangle of side 3 units. Find the coordinates of the other two vertices.
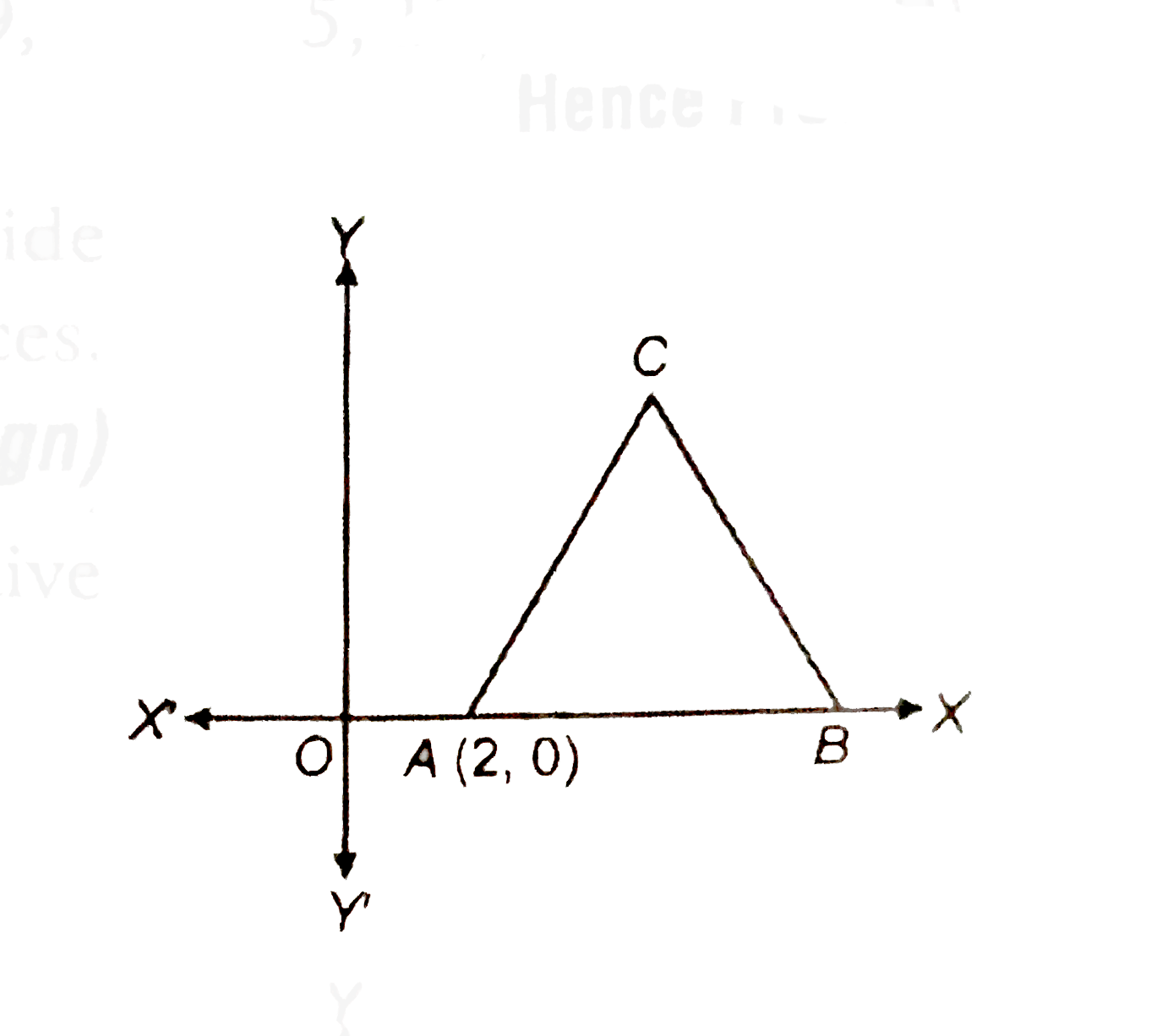 View Solution
View SolutionShow that the points (a,a),(−a,−a)and(−3a,3a)are the vertices of an equilateral triangle. Also, find its area.
View SolutionIf O is the origin, OP=3 with direction ratios −1,2,and−2, then find the coordinates of P.
View Solution

