If (x,y) and (x,y) are the coordinates of the same point referred to two sets of rectangular axes with the same origin and it ux+vy, where u and v are independent of xandy , becomes VX+UY, show that u2+v2=U2+V2.
To solve the problem, we need to show that if the coordinates of the same point in two sets of rectangular axes are given by the equations ux+vy and vx+uy, then it follows that u2+v2=U2+V2.
1. Understanding the Coordinates:
Let the coordinates of the point in the original axes be (x,y) and in the new axes be (X,Y). The transformation between these coordinates can be represented by a rotation of angle θ.
2. Rotation Transformation:
The coordinates can be expressed as:
x=Xcosθ−Ysinθ
y=Xsinθ+Ycosθ
3. Substituting into the Expressions:
Substitute these expressions into the given equations. We start with:
ux+vy
Substituting for x and y:
ux+vy=u(Xcosθ−Ysinθ)+v(Xsinθ+Ycosθ)
Expanding this gives:
ux+vy=uXcosθ−uYsinθ+vXsinθ+vYcosθ
Rearranging terms, we can group the coefficients of X and Y:
=(ucosθ+vsinθ)X+(vcosθ−usinθ)Y
4. Equating with the New Expression:
According to the problem statement, this expression equals vX+uY. Therefore, we have:
(ucosθ+vsinθ)X+(vcosθ−usinθ)Y=vX+uY
5. Setting Up the Equations:
By comparing coefficients of X and Y, we get two equations:
ucosθ+vsinθ=v(1)
vcosθ−usinθ=u(2)
6. Squaring and Adding the Equations:
Now we will square both equations and add them:
(ucosθ+vsinθ)2+(vcosθ−usinθ)2=v2+u2
Expanding both sides:
u2cos2θ+2uvcosθsinθ+v2sin2θ+v2cos2θ−2uvcosθsinθ+u2sin2θ=v2+u2
Simplifying gives:
u2(cos2θ+sin2θ)+v2(cos2θ+sin2θ)=v2+u2
Since cos2θ+sin2θ=1:
u2+v2=u2+v2
7. Conclusion:
Thus, we have shown that:
u2+v2=U2+V2
Hence, the proof is complete.
Let the coordinates of the point in the original axes be (x,y) and in the new axes be (X,Y). The transformation between these coordinates can be represented by a rotation of angle θ.
The coordinates can be expressed as:
x=Xcosθ−Ysinθ
y=Xsinθ+Ycosθ
Substitute these expressions into the given equations. We start with:
ux+vy
Substituting for x and y:
ux+vy=u(Xcosθ−Ysinθ)+v(Xsinθ+Ycosθ)
Expanding this gives:
ux+vy=uXcosθ−uYsinθ+vXsinθ+vYcosθ
Rearranging terms, we can group the coefficients of X and Y:
=(ucosθ+vsinθ)X+(vcosθ−usinθ)Y
According to the problem statement, this expression equals vX+uY. Therefore, we have:
(ucosθ+vsinθ)X+(vcosθ−usinθ)Y=vX+uY
By comparing coefficients of X and Y, we get two equations:
ucosθ+vsinθ=v(1)
vcosθ−usinθ=u(2)
Now we will square both equations and add them:
(ucosθ+vsinθ)2+(vcosθ−usinθ)2=v2+u2
u2cos2θ+2uvcosθsinθ+v2sin2θ+v2cos2θ−2uvcosθsinθ+u2sin2θ=v2+u2
Simplifying gives:
u2(cos2θ+sin2θ)+v2(cos2θ+sin2θ)=v2+u2
Since cos2θ+sin2θ=1:
u2+v2=u2+v2
Thus, we have shown that:
u2+v2=U2+V2
Hence, the proof is complete.
Topper's Solved these Questions
- COMPLEX NUMBERS AND QUADRATIC EQUATIONSBOOK - CENGAGE ENGLISHCHAPTER - COMPLEX NUMBERS AND QUADRATIC EQUATIONSEXERCISE - All Questions
886 Videos
- DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS BOOK - CENGAGE ENGLISHCHAPTER - DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS EXERCISE - Multiple correct answers type
11 Videos
Similar Questions
If (x,y) and (X,Y) be the coordinates of the same point referred to two sets of rectangular axes with the same origin and if ax+by becomes pX+qY, where a,b are independent of x,y, then
View SolutionFind the number of distinct terms in the expansion of (x+y+z+u)10. (where x, y, z and u are independent variable)
View Solution
Knowledge Check
If u=x−32andv=y−23, then cov(u,v) = k cov(x,y) . The value of k is
A6B16C5D15
If (x+iy)3=u+iv, then show that ux+vy=4(x2−y2)
View SolutionIf (x+iy)3=u+iv,then show that ux+vy=4(x2−y2).
View SolutionIf (x+iy)3=u+iv,then show that ux+vy=4(x2−y2).
View SolutionThe table given below shows the position of element U, V, X, Y and Z.

Which is larger in size V or X
View SolutionThe table given below shows the position of element U, V, X, Y and Z
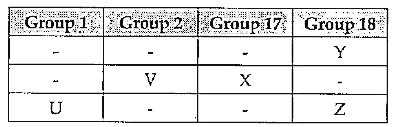
Which ions are formed by elements U and X.
View SolutionThe table given below shows the position of element U, V, X, Y and Z.

Which is larger in size Y or Z
View SolutionTest dimensionally if the v2=u2+2ax may be correct.
View Solution
