If the coordinates of the points A,B,C and D be (a,b),(a',b'),(−a,b) and (a',−b') respectively, then the equation of the line bisecting the line segments AB and CD is
To find the equation of the line bisecting the line segments AB and CD given the points A, B, C, and D with coordinates A(a,b), B(a′,b′), C(−a,b), and D(a′,−b′), we will follow these steps:
Step 1: Find the Midpoint of Line Segment AB
The coordinates of points A and B are A(a,b) and B(a′,b′).
The formula for the midpoint MAB of a line segment with endpoints (x1,y1) and (x2,y2) is:
MAB=(x1+x22,y1+y22)
Substituting the coordinates of A and B:
MAB=(a+a′2,b+b′2)
Step 2: Find the Midpoint of Line Segment CD
The coordinates of points C and D are C(−a,b) and D(a′,−b′).
Using the midpoint formula:
MCD=(−a+a′2,b+(−b′)2)=(−a+a′2,b−b′2)
Step 3: Find the Slope of the Line through Midpoints M_AB and M_CD
Let MAB=(x1,y1)=(a+a′2,b+b′2) and MCD=(x2,y2)=(−a+a′2,b−b′2).
The slope m of the line through these two points is given by:
m=y2−y1x2−x1
Substituting the values:
m=b−b′2−b+b′2−a+a′2−a+a′2
=b−b′−b−b′2−a+a′−a−a′2=−2b′2−2a2=−b′−a=b′a
Step 4: Use the Point-Slope Form to Write the Equation of the Line
Using the point-slope form of the equation of a line:
y−y1=m(x−x1)
Substituting m=b′a and (x1,y1)=(a+a′2,b+b′2):
y−b+b′2=b′a(x−a+a′2)
Step 5: Rearranging to Standard Form
To simplify this equation, we can multiply through by 2a to eliminate the fractions:
2a(y−b+b′2)=b′(x−a+a′2)
2ay−(b+b′)a=b′x−b′(a+a′)2
Rearranging gives us:
b′x−2ay+(b+b′)a−b′(a+a′)2=0
This is the equation of the line bisecting segments AB and CD.
The coordinates of points A and B are A(a,b) and B(a′,b′).
MAB=(x1+x22,y1+y22)
MAB=(a+a′2,b+b′2)
The coordinates of points C and D are C(−a,b) and D(a′,−b′).
MCD=(−a+a′2,b+(−b′)2)=(−a+a′2,b−b′2)
Let MAB=(x1,y1)=(a+a′2,b+b′2) and MCD=(x2,y2)=(−a+a′2,b−b′2).
m=y2−y1x2−x1
m=b−b′2−b+b′2−a+a′2−a+a′2
=b−b′−b−b′2−a+a′−a−a′2=−2b′2−2a2=−b′−a=b′a
Using the point-slope form of the equation of a line:
y−y1=m(x−x1)
y−b+b′2=b′a(x−a+a′2)
To simplify this equation, we can multiply through by 2a to eliminate the fractions:
2a(y−b+b′2)=b′(x−a+a′2)
2ay−(b+b′)a=b′x−b′(a+a′)2
b′x−2ay+(b+b′)a−b′(a+a′)2=0
Topper's Solved these Questions
Similar Questions
If the coordinates of the points A, B, C, D be (1,2,3), (4,5,7), (4,3,6)and (2,9,2)respectively, then find the angle between the lines AB and CD.
View SolutionIf the coordinates of the points A,B,C be (−1,5),(0,0) and (2,2) respectively, and D be the middle point of BC, then the equation of the perpendicular drawn from B to the line AD is
View Solution
Knowledge Check
In the rectangular coordinate system shown, ABCD is a parallogram. If the coordinates of the points A,B,C and D are (0,2),(a,b),(a,2) and (0,0), respectively, then b =
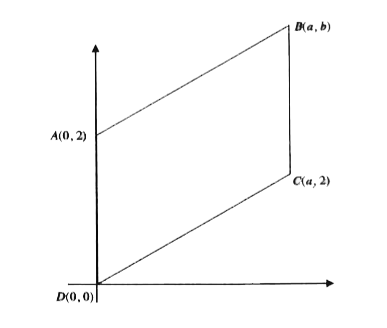 A0B2C3D4
A0B2C3D4
Point A and B have co-ordinates (7, -3) and (1,9) respectively. Find :
the equation of perpendicular bisector of the line segment AB.
View SolutionIn an isosceles triangle ABC, the coordinates of the points Band C on the base BC are respectively (1,2) and (2.1). If the equation of the line AB is y=2x, then the equation of the line AC is
View SolutionIf A and B are (−2, −2) and (2, −4), respectively, find the coordinates of P such that AP=37ABand P lies on the line segment AB.
View SolutionThe co-ordinates of A, B, C are respectively (-4, 0), (0, 2) and (-3, 2). Find the co-ordinates of the point of intersection of the line which bisects the angle CAB internally and the line joining C to the middle point of AB is
View SolutionIf A and B are (2,2) and (2,4) , respectively, find the coordinates of P such that AP=37AB and P lies on the line segment AB.
View SolutionDoes the line 3x=y+1 bisect the line segment joining A (-2, 3) and B (4, 1)?
View SolutionFind the equation of the right bisector of the line segment joining the points (a,b)and (a1,b1).
View Solution