Two parallel tangents to a given circle are cut by a third tangent at the points AandB. If C is the center of the given circle, then ∠ACB (a)depends on the radius of the circle. (b)depends on the center of the circle. (c)depends on the slopes of three tangents. (d)is always constant
To solve the problem, we need to analyze the situation involving the circle, the tangents, and the angles formed. Here’s a step-by-step breakdown of the solution:
Step 1: Understand the Configuration
We have a circle with center C and two parallel tangents that touch the circle at points A and B. A third tangent intersects these two tangents.
Step 2: Draw the Tangents and Circle
Draw the circle with center C. Draw two parallel lines (tangents) that touch the circle at points A and B. Let’s denote these tangents as line R (touching at A) and line S (touching at B).
Step 3: Identify the Angles
The radius CA is perpendicular to tangent R at point A, and the radius CB is perpendicular to tangent S at point B. Therefore, we have:
- ∠CAB=90∘
- ∠CBA=90∘
Step 4: Analyze the Angles Formed
Let’s denote the angle ∠ACB as θ. The angles formed at point C can be analyzed as follows:
- The angle ∠ACB is formed by the lines CA and CB.
- Since both angles CAB and CBA are 90∘, we can use the property of angles in a triangle.
Step 5: Use Triangle Properties
In triangle ACB:
- The sum of angles in triangle ACB is 180∘.
- Therefore, we can write:
∠ACB+∠CAB+∠CBA=180∘
Substituting the known angles:
θ+90∘+90∘=180∘
This simplifies to:
θ=180∘−180∘=0∘
Step 6: Conclusion
Since ∠ACB is consistently 90∘ regardless of the radius, center, or slopes of the tangents, we conclude that:
- The angle ∠ACB is always constant.
Final Answer
The correct option is (d) is always constant.
---
We have a circle with center C and two parallel tangents that touch the circle at points A and B. A third tangent intersects these two tangents.
Draw the circle with center C. Draw two parallel lines (tangents) that touch the circle at points A and B. Let’s denote these tangents as line R (touching at A) and line S (touching at B).
The radius CA is perpendicular to tangent R at point A, and the radius CB is perpendicular to tangent S at point B. Therefore, we have:
- ∠CAB=90∘
- ∠CBA=90∘
Let’s denote the angle ∠ACB as θ. The angles formed at point C can be analyzed as follows:
- The angle ∠ACB is formed by the lines CA and CB.
- Since both angles CAB and CBA are 90∘, we can use the property of angles in a triangle.
In triangle ACB:
- The sum of angles in triangle ACB is 180∘.
- Therefore, we can write:
∠ACB+∠CAB+∠CBA=180∘
Substituting the known angles:
θ+90∘+90∘=180∘
This simplifies to:
θ=180∘−180∘=0∘
Since ∠ACB is consistently 90∘ regardless of the radius, center, or slopes of the tangents, we conclude that:
- The angle ∠ACB is always constant.
The correct option is (d) is always constant.
Topper's Solved these Questions
- COMPLEX NUMBERS AND QUADRATIC EQUATIONSBook:CENGAGE ENGLISHChapter:COMPLEX NUMBERS AND QUADRATIC EQUATIONSExercise:All Questions
Explore 886 Videos
- DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS Book:CENGAGE ENGLISHChapter:DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS Exercise:Multiple correct answers type
Explore 11 Videos
Similar Questions
Two parallel tangents to a given circle are cut by a third tangent at the point RandQ . Show that the lines from RandQ to the center of the circle are mutually perpendicular.
View SolutionTwo parallel tangents of a circle meet a third tangent at points P and Q. Prove that PQ subtends a right angle at the centre.
View Solution
Knowledge Check
In the xy-plane , a circle with center (6,0) is tangent to the line y=x. what is the radius of the circle ?
A2√6B2√3C3√2D3In the figure, O is the center of the circle. What is average of the numbers a, b, c and d?
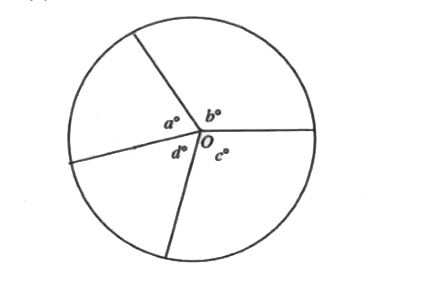 A45B60C90D180
A45B60C90D180
The line 2x−y+1=0 is tangent to the circle at the point (2, 5) and the center of the circle lies on x−2y=4 . Then find the radius of the circle.
View SolutionThe line y=x is a tangent at (0,0) to a circle of radius unity. The center of the circle is
View SolutionIn the given circle if Figure-1, number of tangents parallel to tangents PQ is
View SolutionIn the given circle if Figure-1, number of tangents parallel to tangents PQ is

View SolutionIf the tangent at a point P to a circle with centre O cuts a line through O at Q such that PQ=24cm and OQ=25cm . Find the radius of the circle.
View SolutionA charge particle moves in a circle around an infinite line charge with the center of circle at the line and line being perpendicular to the plane of circle. Let r be the radius of circle. The velocity of the particle depends upon which power of r.
View Solution
