An ellipse slides between two perpendicular straight lines. Then identify the locus of its center.
To solve the problem of finding the locus of the center of an ellipse that slides between two perpendicular straight lines, we can follow these steps:
Step 1: Understand the Geometry
We have an ellipse that is constrained between two perpendicular lines. Let's denote these lines as L1 and L2. The center of the ellipse will be denoted as point C(h,k).
Hint: Visualize the ellipse and the two perpendicular lines. The center of the ellipse will always be at a certain distance from these lines.
Step 2: Identify the Tangents
Since the ellipse is sliding between these lines, at any position, the lines L1 and L2 are tangents to the ellipse. The point where these two lines intersect can be denoted as point P.
Hint: Remember that the tangents to the ellipse from a point outside the ellipse will always be perpendicular to the radius drawn to the point of tangency.
Step 3: Use the Properties of the Ellipse
The equation of the ellipse can be given as:
x2a2+y2b2=1
The center of the ellipse C will always maintain a constant distance from point P (the intersection of the tangents).
Hint: The distance from the center of the ellipse to the point P remains constant as the ellipse slides.
Step 4: Identify the Locus
Since the distance from the center C(h,k) to the point P is constant, we can say that the locus of point C is a circle. The radius of this circle is equal to the distance from the center of the ellipse to the point P.
Hint: A locus that maintains a constant distance from a fixed point is always a circle.
Step 5: Conclusion
Thus, the locus of the center of the ellipse sliding between two perpendicular lines is a circle.
Final Answer: The locus of the center of the ellipse is a circle.
---
We have an ellipse that is constrained between two perpendicular lines. Let's denote these lines as L1 and L2. The center of the ellipse will be denoted as point C(h,k).
Since the ellipse is sliding between these lines, at any position, the lines L1 and L2 are tangents to the ellipse. The point where these two lines intersect can be denoted as point P.
The equation of the ellipse can be given as:
x2a2+y2b2=1
The center of the ellipse C will always maintain a constant distance from point P (the intersection of the tangents).
Since the distance from the center C(h,k) to the point P is constant, we can say that the locus of point C is a circle. The radius of this circle is equal to the distance from the center of the ellipse to the point P.
Thus, the locus of the center of the ellipse sliding between two perpendicular lines is a circle.
Topper's Solved these Questions
- COMPLEX NUMBERS AND QUADRATIC EQUATIONSBOOK - CENGAGE ENGLISHCHAPTER - COMPLEX NUMBERS AND QUADRATIC EQUATIONSEXERCISE - All Questions
886 Videos
- DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS BOOK - CENGAGE ENGLISHCHAPTER - DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS EXERCISE - Multiple correct answers type
11 Videos
Similar Questions
An ellipse of semi-axes a,b slides between two perpendiuclar lines. Prove that the locus of its foci is (x2+y2)(x2y2+b4)=4a2x2y2, the two lines being taken as the axes of coordinates.
View SolutionA line of fixed length a+b moves so that its ends are always on two fixed perpendicular straight lines. Then the locus of the point which divides this line into portions of length aandb is (a) an ellipse (b) parabola (c) straight line (d) none of these
View Solution
Knowledge Check
A line of length a+b moves in such a way that its ends are always on two fixed perpendicular straight lines. Then the locus of point on this line which devides it into two portions of length a and b ,is :
AA. ParabolaBB. CircleCC. EllipseDD. Hyperbola
If the extremities of a line segment of length l moves in two fixed perpendicular straight lines, then the locus of the point which divides this line segment in the ratio 1 : 2 is-
View SolutionA rod of length l slides with its ends on two perpendicular lines. Find the locus of its midpoint.
View SolutionA rod of length l slides with its ends on two perpendicular lines. Find the locus of its midpoint.
View SolutionA rod of length / slides with its ends on two perpendicular lines. Find the locus of its mid-point.
View SolutionAn iron rod of length 2l is sliding on two mutually perpendicular lines . Find the locus of the midpoint of the rod.
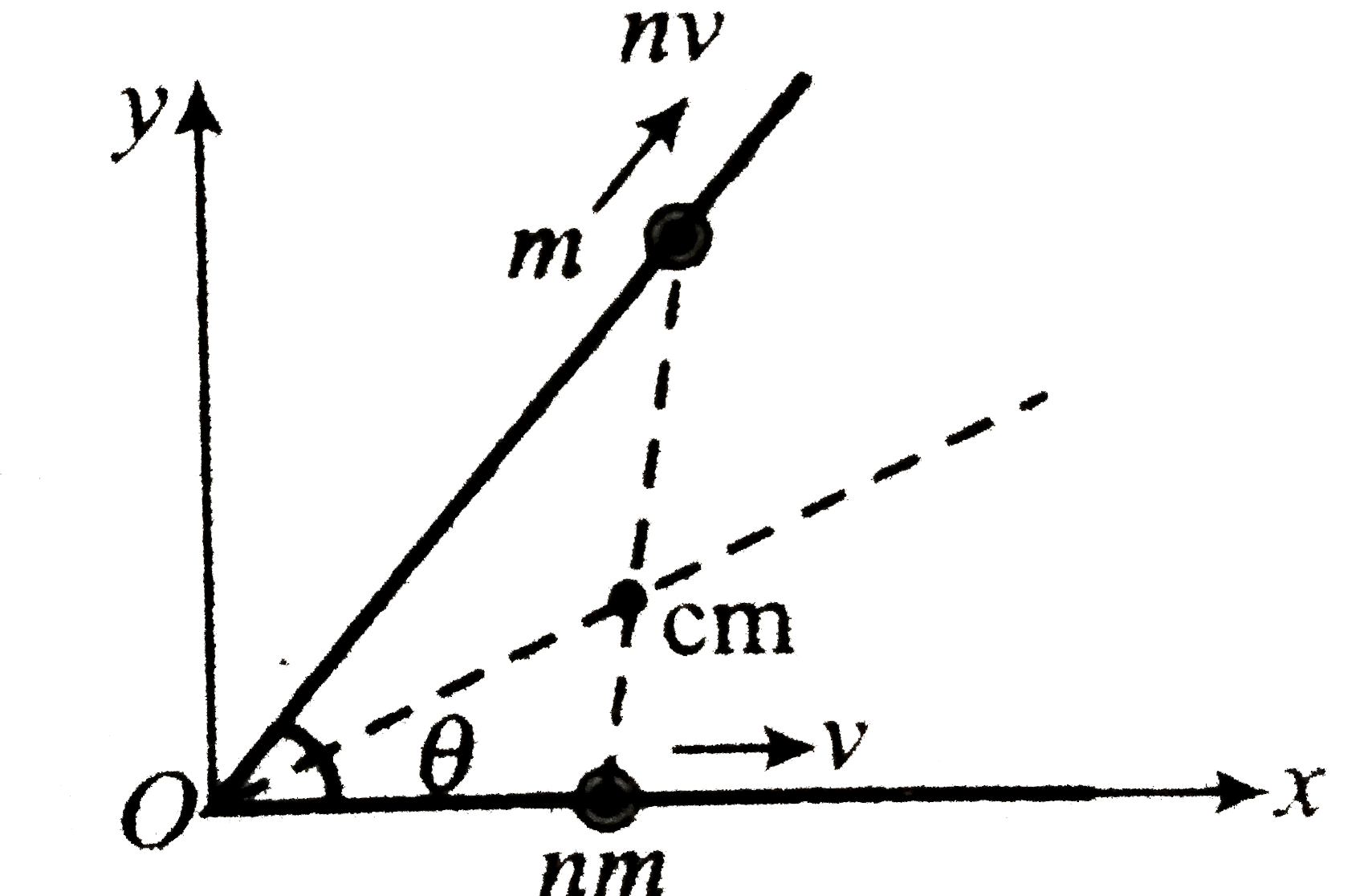
View SolutionTwo masses nm and m, start simultaneously from the intersection of two straight lines with velocities v and nv respectively. It is observed that the path of their centre of mass is a straight line bisecting the angle between the given straight lines. Find the magnitude of the velocity of centre of mass. [Here θ=angle between the lines]
View SolutionA circle cuts two perpendicular lines so that each intercept is of given length. The locus of the centre of the circle is conic whose eccentricity is
View Solution